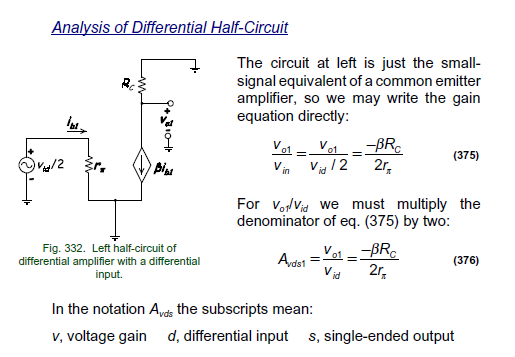
I can't understand why is 2Rpi in equation 375/376. It looks like the current ib1 goes through Rpi of first stage and through Rpi of second stage but it must go through first Rpi to ground and back to signal source. Why it's so?
\$\begingroup\$
\$\endgroup\$
2
-
\$\begingroup\$ The 2 in those formulas has nothing to do with \$r_\pi\$, the 2 comes from \$V_{id}/2\$ because we're looking at one half of a differential pair. \$\endgroup\$– BimpelrekkieCommented Oct 24, 2017 at 7:57
-
\$\begingroup\$ You may want to read through to the last part of my answer here: electronics.stackexchange.com/questions/273922/… I also discuss the narrow limits of the "1/2" assumption, as well. But it isn't targeted directly at your question. So.. maybe it helps, maybe not. \$\endgroup\$– jonkCommented Oct 24, 2017 at 8:03
Add a comment
|
1 Answer
\$\begingroup\$
\$\endgroup\$
Here think of the differential mode half-circuit as the small-signal equivalent of a common-emitter with no degeneration. The gain of such circuit is \$A_v= \frac{v_o}{v_i}=\$ \$-g_m.R_C\$. Here \$v_i=\frac{v_d}{2}\$ and \$g_m=\frac{\beta}{r_\pi}\$. Substituting \$v_i\$ in \$A_v\$ you get \$A_{dm_1}=\frac{2v_{o1}}{v_d}=\$ \$-g_m.R_c\$. Now dividing both sides by 2, you get \$A_{dm_1}=\frac{v_{o1}}{v_d}=\frac{-g_m.R_c}{2}\$. This is the differential gain of a single-ended output of a differential pair.
