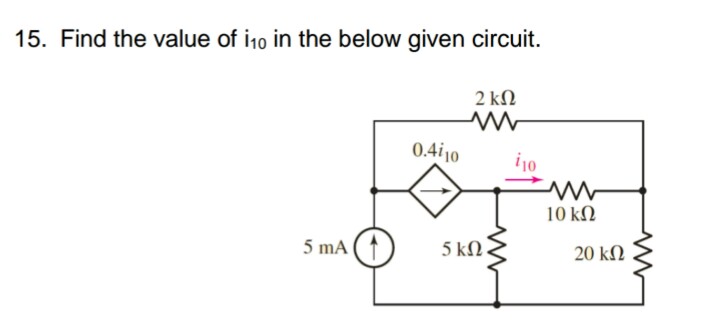
I've spent more than 3 hours with the idea that we should find current across every resistor in terms of \$i_{10}\$. This is somehow not working, I mean putting the KCL (Kirchoff's Current Law) equations of different nodes into each other just makes left and right sides equal. It doesn't give any value of \$i_{10}\$. The dependent current source only makes it more difficult. Please guide me on the best approach to solve this question or if you're kind enough give the solution.
The solution will be highly appreciated!
\$\begingroup\$
\$\endgroup\$
4
-
1\$\begingroup\$ Write down the current through each branch in terms of \$i_{10}\$ and then apply KVL around some loop to figure out the current \$i_{10}\$. \$\endgroup\$– dirac16Commented Oct 28, 2017 at 19:58
-
\$\begingroup\$ @dirac16 would you mind posting the solution? That would be kind of you. Thanks. \$\endgroup\$– Hasaan AhmedCommented Oct 28, 2017 at 20:11
-
3\$\begingroup\$ To the OP: just write the current with an arrow over each resistor. Except, write the current so the only variable is i10. For example, the current through 2k is (5mA - 0.4*i10). The current through 10k is i10. The current through 20k is (i10 + (5mA - 0.4*i10)). Current through 5k is (0.4*i10 - i10). Then sum up the voltage drops going around 10k, 5k, 20k. The total voltage must be zero. At that point you will only have one equation and one unknown. \$\endgroup\$– user57037Commented Oct 28, 2017 at 21:53
-
\$\begingroup\$ Thanks @mkeith. Gives -4mA if my calculations are not wrong. \$\endgroup\$– Hasaan AhmedCommented Oct 29, 2017 at 19:13
Add a comment
|
1 Answer
\$\begingroup\$
\$\endgroup\$
1
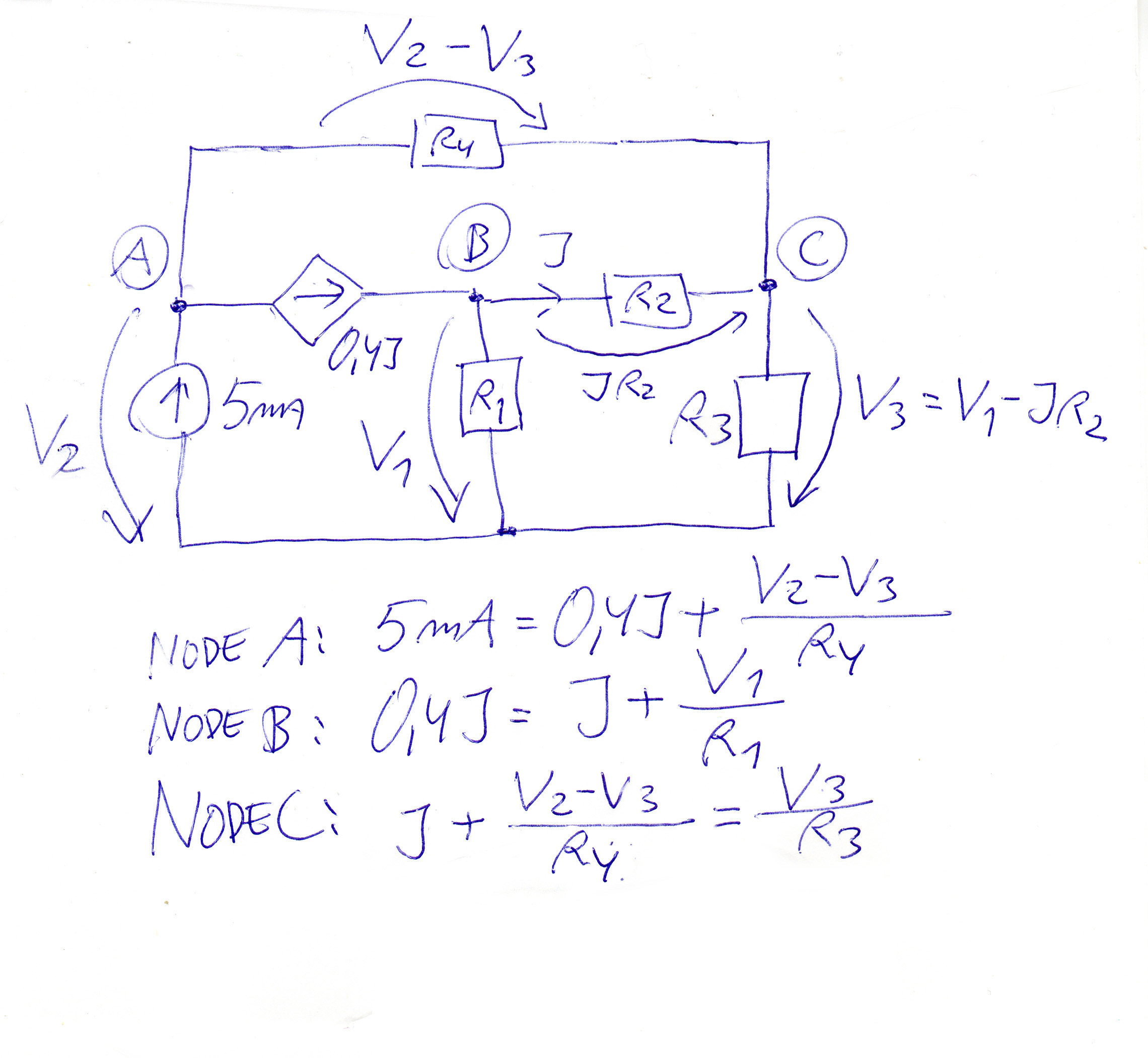
This easily leads to one more unknown voltage or current than the number of equations, if one tries blindly to apply some systematic method. The missing equation fortunately can be found after a little thinking:
Here we have three node equations and four unknowns. The missing equation here is the fact that V3 = V1-I*R2. By inserting it we have 3 unknowns left and our three node equations are enough.
(stop, I never give full solutions to homeworks)
-
1\$\begingroup\$ Thank you for help, will try to finish it now. Thanks again. \$\endgroup\$ Commented Oct 28, 2017 at 21:14