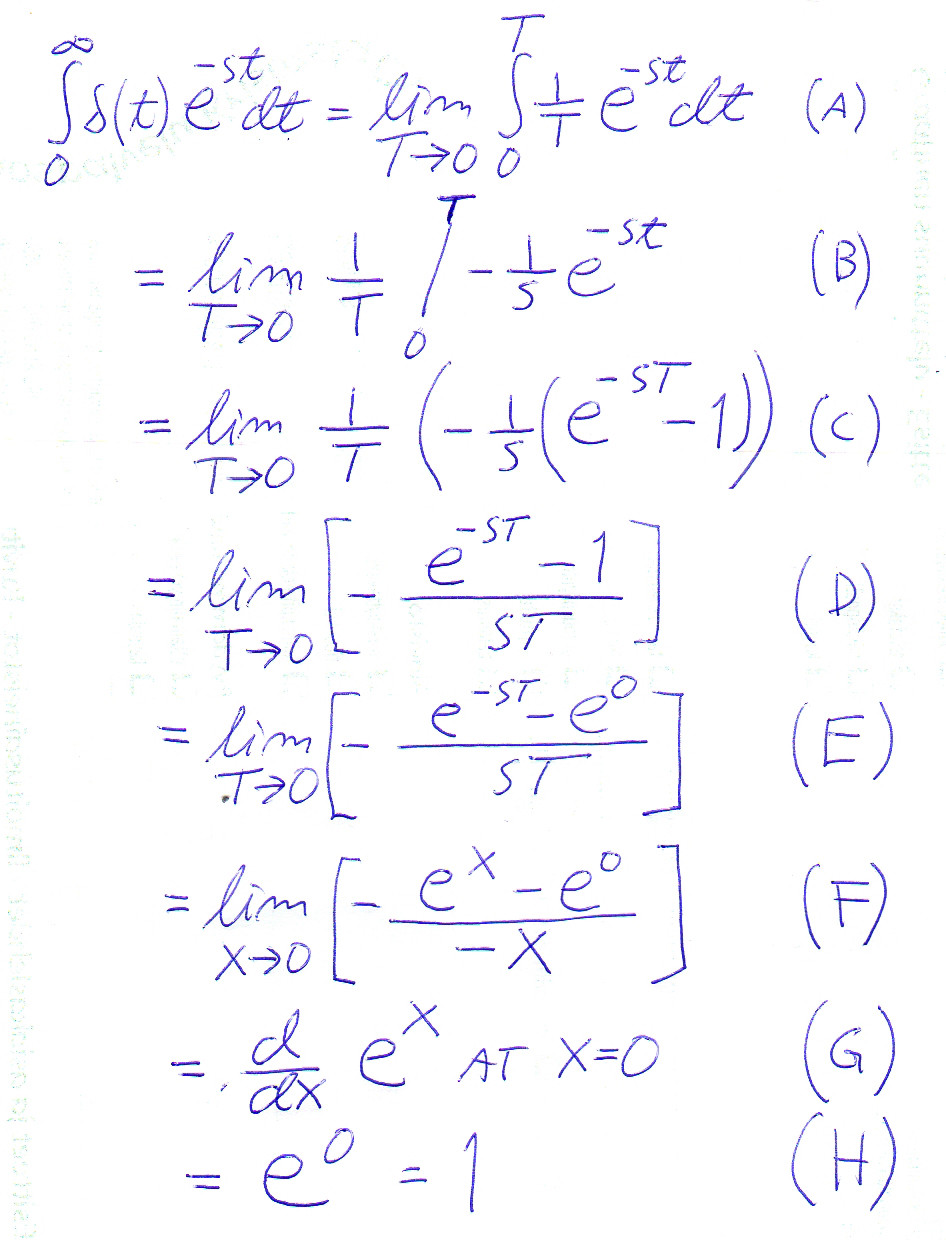Does an impulse function at t=0 go to infinity, or 1?

Question

Impulse function is basically the derivative of the step function u(t):

$$ u(t)= \begin{cases} 0 & \text{if $t<0$} \\ 1 & \text{if $t\geq0$} \end{cases} $$

I was taught that in laplace domain, if the transfer function $$H(s)=\frac{N(s)}{D(s)}$$ has a higer degree in the numerator than in the denominator, then the circuit is unstable because that would mean $$H(s)=1+\frac{R(s)}{D(s)}$$ and the inverse laplace of 1 is the impulse function, which my professor says goes to infinity.

But what I don't get is why the laplace transform of the impulse function is equal to $$\int^{\infty}_{0}\delta(t)e^{-st}dt=e^{-s\times 0}=1$$

Answer 1

The \$\delta(t)\$ is defined as positive infinite amplitude and infinitesimal width with an area of 1 at \$\delta(0)\$, and 0 otherwise.

As Wikipedia states:

\$\int^\infty_{-\infty}\delta(t)~dt = 1\$

Since it only has one point that has a non-zero value, which is at \$\delta(0)\$, then that is the only point we need to consider. And the area at that point is 1 per definition.

---

And \$x^0=1\$ which is why \$e^{-s×0}=1\$

Answer 2

$$\int^{\infty}_{0}\delta(t)e^{-st}dt$$ If you re-phrase this expression as, say: $$\int^{\infty}_{0}f(t)\times g(t)dt$$ now since, $$f(t)=\delta(t)$$ it has non-zero value only at t = 0. Which means the whole integral has non-zero value ONLY at t = 0. For all other values of t, the summation or the integral is just 0. So we can simplify the integral expression to: $$f(0)\times g(0) = \delta(0)e^{-0\times s} = 1$$

Answer 3

We, electricians, use often simplified math which isn't exact. Impulse is probably the most common example. The next are "infinitesimal" time and voltage intervals.

We use those concepts to avoid the complexity of proper limit processes that exact math uses. Mathematicians have a long time ago investigated our impulses and infinitesimal intervals, defined them logically ok and told us how we should use those things to get right results.

If one wants to write his formulas perfectly (in math) without impulses, he needs to do his work as complex limit process using a sequence of pulses or a parametrized pulse which grows limitlessly as the parameter - the lenghth of the pulse - nears to zero, but at all lengths the pulse still has the same definite time integral =1.

A fortunately large class of useful cases exist, where our shortcut - the impulse - gives the same results than a mathematically sound limit process. We must only remember a few properties of the impulse. Two of them:

• it's zero when t is not zero
• the Laplace transform of the impulse is =1

Impulse can be used also to take samples of a continuous signal and much more, but that is another story.

To explain the Laplace transform of the impulse properly, we must do the limit process. This is not full proof, because we have only one simple class of pulses.

Let's do the limit process using pulses which start at t=0, have length =T and the height =1/T. (Sorry, I speak LaTex so poorly that I skip it)

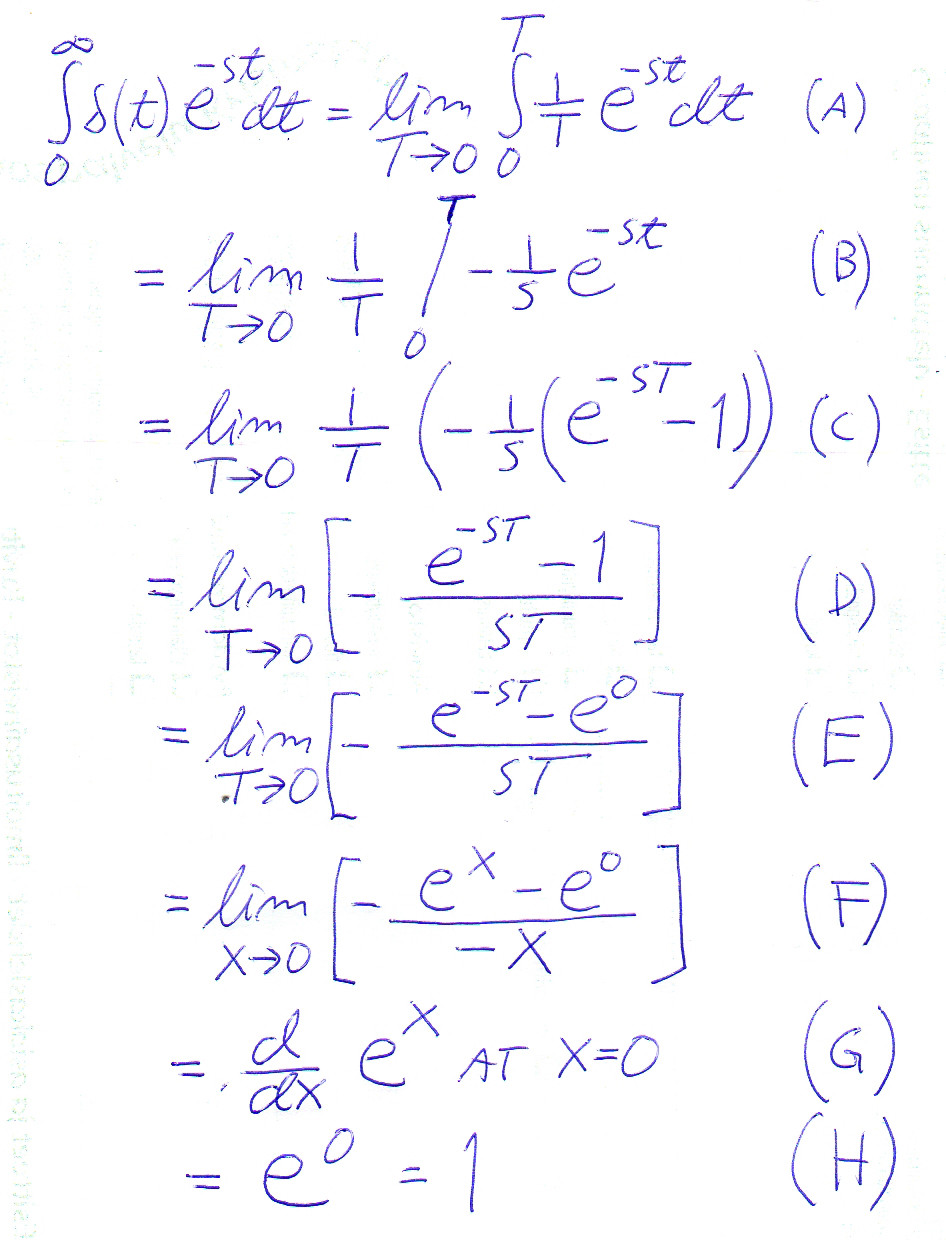
I believe that rows A to E are trivial for everyone who can understand the definition of the Laplace transform. Row F has a new variable x=-sT. With it we get rid of two minus signs. In addition our limit expression clearly is the derivative of the exponent function, calculated at x=0. It is =1.

So, this is a proof, when we go through rectangular pulses which have "area"=1 and the length approaches to zero. I must admit that I do not perform limit process circus numbers like this especially often. I do like user Harry Svensson wrote. I think the impulse as infinitely high pulse which has area=1 and which is placed at t=0. In Laplace transform definition equation the impulse takes along only the value of the exponential at t=0.