Diode Circuits using expotential model

Question

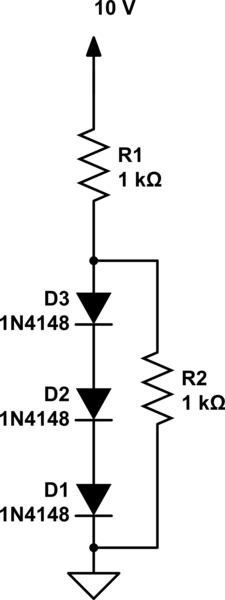
^{simulate this circuit – Schematic created using CircuitLab}

Hi, Question: In the following, a string of three diodes is used to provide a constant voltage of about 2.1 V. We want to calculate the percentage change in this regulated voltage caused by (a) a ± 10% change in the power-supply voltage and (b) connection of a 1-kΩ load resistance. Assume n = 2.

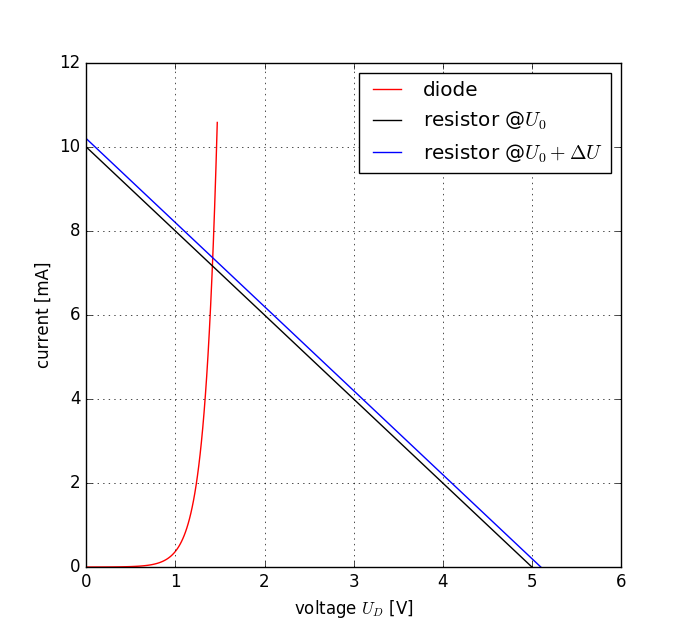
I had some doubts regarding the small signal analysis to be carried out in part B. After asking here, I got enough help to understand and solve it. But the next passage in the book (Microelectronic Circuits) states that while using small signal analysis we get .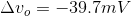

But if we follow the detailed calculations using the exponential model we would get .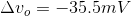

I am not able to understand how to derive the answer using the exponential model. What are the steps to find the solution using the exponential model?

Link (to the previously asked question: Diode Modelling)

Answer 1

Writing down KCL equations in \$v_{OUT}\$, while assuming all diodes are equal such that \$v_D = \frac{v_{OUT}}{3}\$ (current flowing in = current flowing out):

\$\frac{10V-v_{OUT}}{R_1} = \frac{v_{OUT}}{R_2} + I_s\left(e^{\frac{v_{OUT}}{3n\ U_t}}-1\right)\$

This is a transcendental equation that cannot be solved analytically. It should be solved using a numerical method, which is what circuit simulators do.

Answer 2

First you can replace the voltage source and voltage divider by its Thevenin equivalent and the three diodes by one which has just different parameters:

^{simulate this circuit – Schematic created using CircuitLab}

Assuming the diodes are modelled by the Shockley diode equation
\$I(U) = I_s (e^{\frac{U}{U_c}} - 1)\$
then the combined diode (tripple diode) can be modelled by
\$I(U) = I_s (e^{\frac{U}{3U_c}} - 1)\$

So the problem looks simpler; basically there is no difference compared to a circuit with voltage source, one resistor and one diode.

Now look at the I-U graphs of diode and resistor and the load line:

As you can see the curvature of the diodes I-U-line (red) doesn't change much in the region where it intersects the load lines (black, blue).

You can calculate the voltage at which the load lines intersect approximately the diodes line using linear approximation.

The difference of the voltages of the intersection will be much smaller than \$\Delta U\$ because the diodes graph is much steeper in that region than the load line.