Colpitts oscillator's capacitors

Question

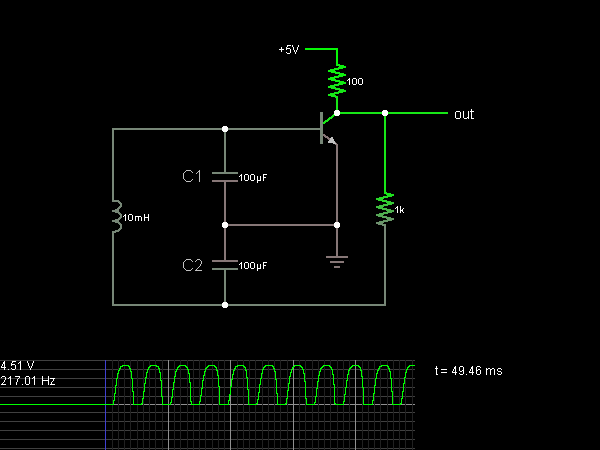
I've been reading more about how oscillators work and one of the more simple ones is the Colpitts oscillator. My understanding is that two capacitors in combination with the inductor can produce oscillations at a given frequency, and the transistor ensures that the oscillations persist. Other schematics use additional capacitors which I'm unclear about, but which I don't think affect the frequency unless they're part of the tank circuit. I'm definitely confused about what the values of the capacitors should be relative to each other, because some schematics suggest using capacitors with the same values or two different values, and I'm unclear on how or if that affects the output of the circuit (if you had two capacitors with the same value or two with different values but their combined capacitance was the same). Also, what part of the oscillator is generally used for the output? Should it be the collector and emitter to get a full AC waveform?

Answer 1

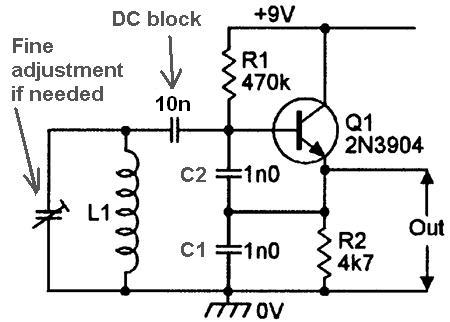
The common collector Colpitts oscillator is not what you have drawn. It is like this: -

Image modified from BIPOLAR TRANSISTOR COOKBOOK — PART 5 by Ray Marston

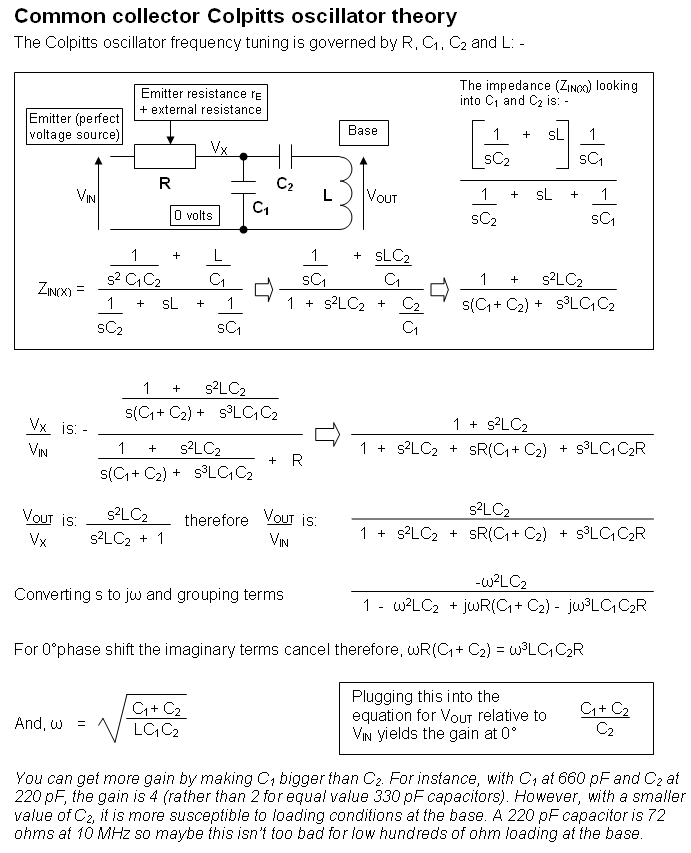
Here I derive the oscillation frequency and the capacitor ratio: -

Answer 2

The key for designing a working oscillator circuit is to know the basic principle of the feedback arrangement.

The oscillation condition (Barkhausen) requires a (positive) loop gain of unity (or - for practical reasons - somewhat larger than unity). That means: Feedback with zero phase shift. Because there is a phase inversion between base an collector of the transistor (180deg phase shift) we need a feedback network that can produce another 180deg phase shift at at one single frequency.

For this purpose, these oscillator types (Colpitt, Pierce. Clapp) use a 3rd-order lowpass or highpass network (ladder topology).

The given circuit contains a 3rd-order lowpass ladder network:

(100+1k)-C2-L-C1.

Hence, you have nothing to do than to find the transfer function of the passive feedback circuit (lowpass) and to select proper parts values to realize a phase shift of -180deg at the desired oscillation frequency. (Of course, you also can use corresponding formulas which can be found in the literature).

Comment 1: As you will have noticed, I did not mention the term "tank circuit" at all. The working principle of the oscillator can best be explained using the lowpass view.

Comment 2: The transistor needs a suitable DC bias point - otherwise you cannot expect a sinusoidal output voltage.

Comment 3: It is a simple task to find the frequency for which the phase shift is 180deg. Find the imaginary part of the transfer function for the passive feedback network - and set the imag. part equal to zero )because fr 180 deg phase shift the function is negative-real). Then, solve for the frequency wo.

Answer 3

We have a seesaw, into which we inject energy. Initiially the energy is just amplified thermal noise, probably some moderate nanovolts, unless power-supply noise occurs at the Fosc frequency

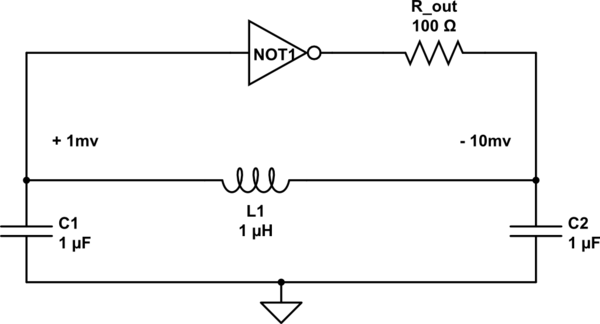
^{simulate this circuit – Schematic created using CircuitLab}

If the amplifier (the NOT1) has gain of -10x, the signal will increase approximately 10x on each pass thru the seesaw resonant filter. Starting at a few nanoVolts, the amplitude grows, constrained on each pass by the ability to transfer energy thru the 100 ohm resistor into the C2 capacitor.