Impedance Matching With L-Network, Terminology Issues

Question

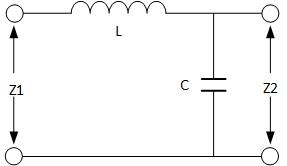
Note: Using L Networks

I am having some confusion with the terminology of impedance matching. Specifically majority of questions i have done state match a load to the source impedance which i interpret as the load must be essentially the source impedance after the other components are found (i.e. the cap and inductor etc).

However i have come across a question which stated to match the output impedance of a signal source to a resistive load at a specified frequency f0 for maximum power transfer. I am confused for some reason by this wording. Does it mean that the source must be matched to the load instead so the source should be seen as the load after the other components are calculated? Or can i just do it like the other questions by matching the load to the source?

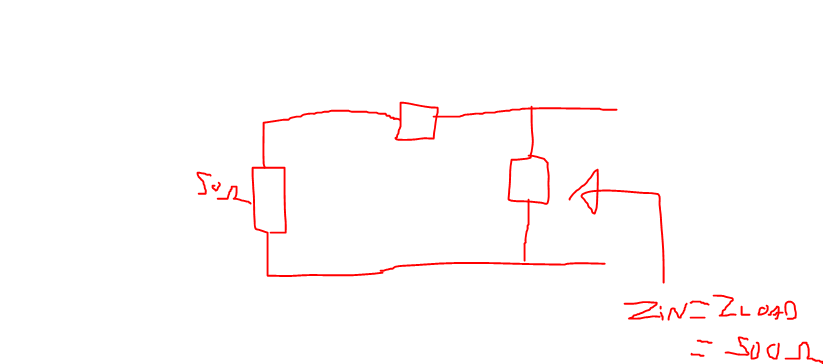
As for the example 1 below if we are matching the source (50 ohm) to the load (500ohm), does this mean for calculations zin would be the load or not?

Or is this the same as just doing it the normal way by matching the load to the source. i.e.: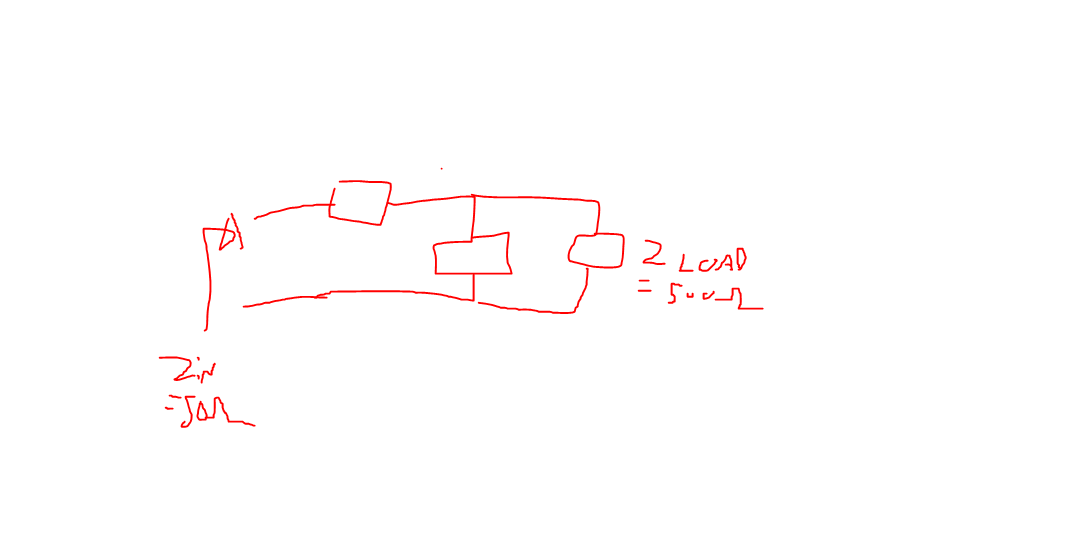

If it is the same why is it the same? Thanks in Advance

Answer 1

It's probably less confusing to describe an L-Network in terms of the Hi-Z and Lo-Z ports, since it only transforms in one "direction." Referring to the sketch below, I have labeled the two ports Z1 and Z2. The way it's drawn, Z2 must always be higher impedance than Z1. This would be the case in your question where it appears you are trying to match a 50 ohm source to a 500 ohm load: Z1 would be 50 ohms and Z2 would be 500 ohms.

If you need to transform your 50 ohm source to say, 10 ohms, then your source would be connected to Z2 and the 10 ohm load to Z1. Or course, you would probably redraw the circuit so that your source is on the left, which simply means the capacitor now shunts the source instead of the load.

Just remember, with the L-Network, the capacitor always shuts the higher impedance port.