0
\$\begingroup\$

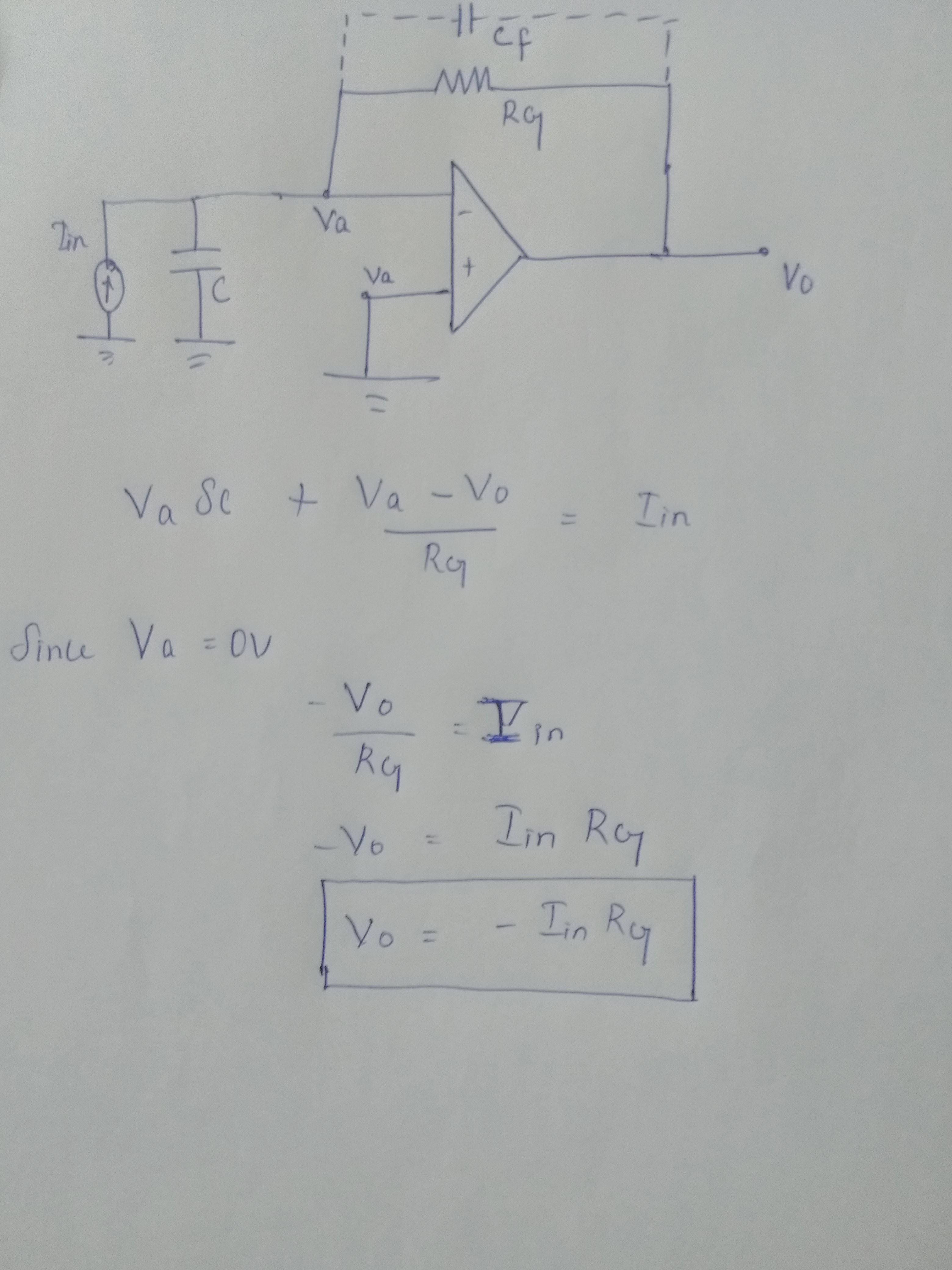
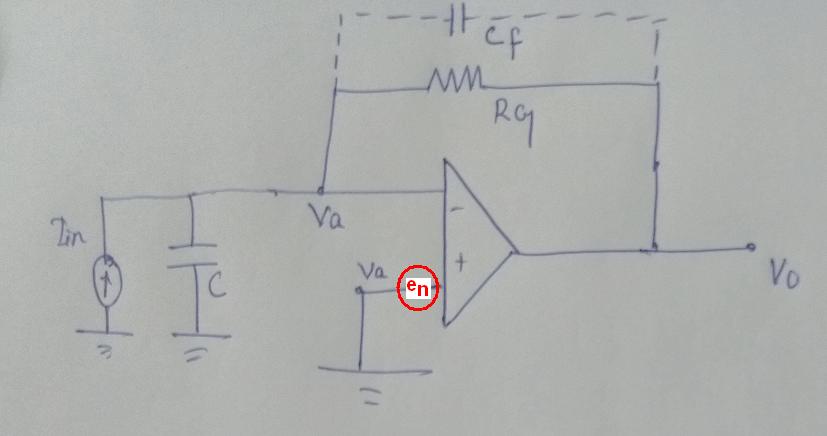
I am learning analog design. I am trying to understand transimpedance amplifier using Op-Amp. Here is my transfer function.

enter image description here

My understanding is assuming Node Va as virtual group I got (Vout= -Iin*Rg) which means feedback is frequency independent (No impact because of input Capacitor C). Here what is the purpose of adding a capacitor in feedback path for compensation(adding Zero) since feedback is frequency independent. How to find where to add zero and what value cap to be added? Can someone point to good reading book/website?

Please correct me if my derivation is wrong.

\$\endgroup\$
2
  • \$\begingroup\$ Look up papers by Bonnie Baker (once Burr Brown, now at TI I think -- I've spoken with her from time to time) and Jerald Graeme (also TI) and Phil Hobbs (whom I've also worked with from time to time.) Papers also on the ACF2101 and DDC112 as well as technical discussions from Hamamatsu, regarding their photodiodes. Books by any of those three authors would be helpful, I think. Also see: Feedback Plots Define OP AMP AC Performance by Graeme, perhaps? \$\endgroup\$
    – jonk
    Apr 12, 2018 at 6:12
  • \$\begingroup\$ I recall good discussions with Jim Todsen on the DDC112, so anything from him may also help some. \$\endgroup\$
    – jonk
    Apr 12, 2018 at 6:17

1 Answer 1

Reset to default
1
\$\begingroup\$

Consider that every amplifier has a voltage noise source (\$e_n\$) associated with the inputs. Here is one realization of how that may be analysed: -

enter image description here

So, if the feedback capacitor were not there and you calculated how much output noise the op-amp would produce (due to \$e_n\$), you would see that at some frequency (\$R_g/X_c\$) the output noise would start to rise.

For the perspective of the noise source (\$e_n\$), noise gain is: -

\$1+ \dfrac{R_g}{X_c}\$ and, as \$X_c\$ gets smaller, output noise rises to a maximum.

This is why \$C_f\$ is used - it tries to counter the noise effects by shunting \$R_g\$.

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge that you have read and understand our privacy policy and code of conduct.

Not the answer you're looking for? Browse other questions tagged or ask your own question.