Why is wireless power transfer done between two coils at their self-resonant frequency?

Question

I was reading this paper about wireless power transfer via strongly coupled magnetic resonance:

http://www.ijirem.org/DOC/1_Wireless%20Power%20Transfer%20via%20Strongly%20Coupled%20Magnetic7a7f01ee-bd3f-43bc-a14d-3309f1040291.pdf

I understand that power is transferred more efficiently between two coils - the transmitter and receiver coils - when they are at resonance, i.e., when they have the same frequency. I am fine with this.

But then the report mentions that the two coils operate at the self-resonance frequency, which is when the inductive component of a coil is equal to its stray capacitance. Doesn't this mean that the inductive and capacitive components of the transmitter coil cancel out each other, and no magnetic field is produced by the transmitter coil?

Answer 1

at self-resonance there is a very high impedance, so shouldn't the coil behave like an open circuit?

For a parallel tuned resonance: -

The current into the inductor at resonance is exactly opposite in sign but equal in magnitude to the current into the capacitor hence those currents add to become perfectly zero in the feed wire to the tuned circuit.

However there is still an excitation voltage attached to the tuned circuit and there is still an inductor attached to that excitation voltage hence there is still inductor and capacitor current.

Probably what confuses you is that how can you make "some" current from "zero" current. Well it doesn't begin that way, when you first apply a resonating sine wave to a parallel tuned circuit you don't instantly get infinite impedance - you have to get energy flowing into the two reactive components and this takes time so what you have is energy taken from the supply.

Once the energy delivered to the reactive components is done, those two perfect components can sit there swishing their voltage and currents back and forth to each other even if the sine wave is removed (just like setting a pendulum in motion by pushing it).

So, a magnetic field is produced by the coil and if this induces eddy currents into a local piece of metal then energy is removed and the sine wave has to restore the energy. If this magnetic field couples energy to another coil then energy is removed and this will be restored by the drving sine wave hence power transfer.

Answer 2

Please see the ideal transformer equivalent circuit.

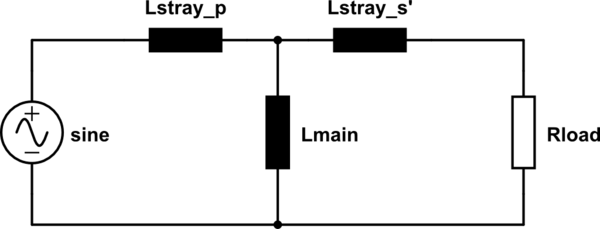
^{simulate this circuit – Schematic created using CircuitLab}

There are no losses, our ideal transformer is only inductances. As the stray inductances do nothing but store energy in the magnetic field of the free space, we want to have them small – we do this by tight magnetic coupling and only small air gaps.

So the main inductance is all we have to talk about. We want its reactance being huge. Why? Because it's a short across our load! The power source have to supply all the current through the load, that's our "payed load", but it also has to supply all the magnetization current through the main inductance, an "unnecessary" current our supply has to be built for. It's a purely reactive current so energy is not lost, but the supply has to be bigger than necessary if the reactance of the main inductivity is low.

If you consider a standard 50/60Hz transformer, the main reactance is made huge by putting a lot of windings on an iron core. We cannot do that if we had a huge air gap. Our main inductance is not much higher than the stray inductances. So we have to increase the frequency. But this has a downfall, capacitances become important:

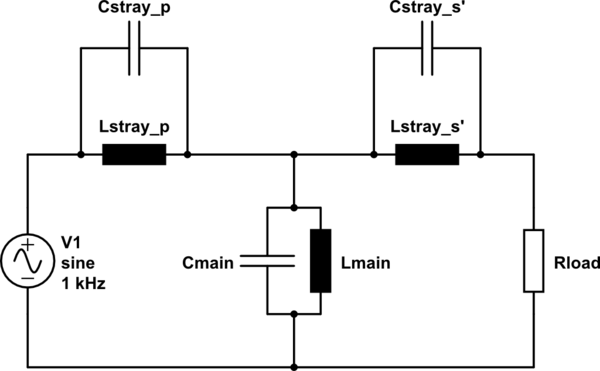
^{simulate this circuit}

If we had to optimize this, it easy to see we cannot just increase the frequency. Because we also had to supply the reactive current through the main capacitance, and the size of our source is what we want to minimize. So the solution is to drive the transformer at the resonant frequency of its main inductance/capacitance.

(The main capacitance in other terms is also a stray capacitance, of course.)

Answer 3

I think you are talking about rotary transformers which normally run at 10 KHZ. They last longer than slip-rings but cannot transfer 100's of amps to a gun turret in a tank.

Then there are proximity transformers which vary a lot in design and range. Your cell-phone dock in your car or at home uses a frequency compatible with coils that may not be perfectly aligned. Charging is slow this way.

I do not understand your notion of self-resonance. The coils transmitting the power and the coils receiving the power are wound to match the frequencies used. You could call it a 'tuned' circuit, but to imply precise resonance under all conditions is not possible. If it is 90% efficient that usually is good enough.

The only self-resonant circuit I know of is called a tank circuit, usually a capacitor in parallel with an inductor. At some ideal frequency they resonate, and that was the beginning of AM radio.

There are many variations of RCL resonate circuits. Search Google with 'RCL' and you will get plenty to read.