15
\$\begingroup\$

Either my textbook author is a scoundrel or I don't have the prerequisites to understand even a simple op-amp circuit. I understand how a basic inverting amplifier works and I get how the gain falls off due to the internal RC circuit (miller C).

What I don't understand in below circuit is how the value of the resistor \$R\$ changes the bandwidth. Since gain-bandwidth product is generally constant, this circuit must be very clever to manipulate bandwidth without touching the gain. I'm attaching the full snapshot of my textbook explanation. It says bandwidth varies with \$R\$ and gives equations, but doesn't explain how or why. Please help me understand how this works.

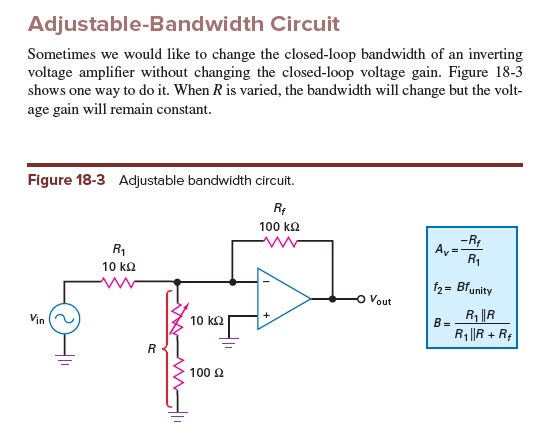
textbook 1

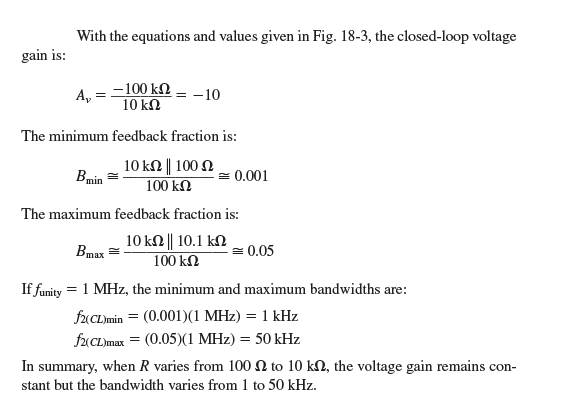
textbook 2

\$\endgroup\$
4
  • 1
    \$\begingroup\$ Without capacitors it makes no sense, and no circuit would normally be designed that way. It may do as you say, but it is abnormally straining the limits of what an op-amp can do. I suspect it has to do with R being an adjustable LPF based on R without a capacitor. \$\endgroup\$
    – user105652
    Commented May 26, 2018 at 6:46
  • 1
    \$\begingroup\$ Sparky256 - I do not agree. The shown circuit modification is one of the methods of input compensation. The resistor R has no influence on the closed-loop gain but it lowers the LOOP GAIN (and, hence, the bandwidth of the closed-loop gain). As a result, the stability margin is improved and you can use opamps which are NOT unity-gain compensated for gain values as low as unity. \$\endgroup\$
    – LvW
    Commented May 26, 2018 at 8:45
  • \$\begingroup\$ rsadhvika-just for the sake of exactness: Your first comment to ishank`s answer is wrong! In his answer as well as your comment you have forgotten the influence of the feedback signal (which also is reduced due to R). \$\endgroup\$
    – LvW
    Commented May 26, 2018 at 16:30
  • \$\begingroup\$ Read up on the concept of 'noise gain' (It is effectively the gain that would exist if you drove the non inverting pin), as it is this gain that is the one in GBP, and it should be obvious that the noise gain in the circuit varies from ~21 (1 + 100k/~5k) to ~1000 (1 + 100k/~100). \$\endgroup\$
    – Dan Mills
    Commented Oct 13, 2019 at 14:18

3 Answers 3

Reset to default
13
\$\begingroup\$

The author is correct in saying that the bandwidth varies with R but the gain doesn't.
This result can be understood easily if we combine the voltage source that is in parallel with R with R itself to get a Thevenin equivalent at the inverting terminal of the opamp.
The Thevenin equivalent will be $$R_{th} = R_1 ||R$$ $$V_{th} = \frac{V_{in}(R_1||R)}{R_1}$$ And the expression for the gain is $$A_v = \frac{V_o}{V_i} = -\frac{R_f}{R_1}$$ Which is independant of R.

As the OP has correctly pointed out, the Gain Bandwidth product of an amplifier remains constant no matter the extent of feedback. More on this can be found here and here.
The trick is that the input to the feedback amplifier (inverting amplifier) is Vth and not Vin.
So by increasing R the gain is falling (Denominator increases), since the gain is $$\frac{V_o}{V_{th}} = -\frac{R_f}{R_1 ||R}$$ and consequently, since GBW remains constant, Bandwidth must increase.

\$\endgroup\$
11
  • 1
    \$\begingroup\$ I think I get it ! Because of \$R\$, opamp is seeing a reduced input voltage of \$V_{th}\$ at its inverting input. (\$V_{th} \lt V_{in}\$ .) GBW refers to the gain \$\dfrac{V_o}{V_{\color{red}{th}}}\$, not the gain \$\dfrac{V_o}{V_{\color{red}{in}}}\$ right ? If so, I think this has been my big mistake.. Thank you for making it so clear :) \$\endgroup\$
    – AgentS
    Commented May 26, 2018 at 8:26
  • \$\begingroup\$ Ishank Juneja-bandwidth is increasing? Typing error? In contrary, addition of the resistor decreases loop gain and with it, of course, also the closed-loop bandwidth \$\endgroup\$
    – LvW
    Commented May 26, 2018 at 9:16
  • \$\begingroup\$ @rsadhvika absolutely, since Vth is what the std inverting amplifier sees and not Vi \$\endgroup\$
    – ijuneja
    Commented May 26, 2018 at 9:53
  • \$\begingroup\$ @LvW for the given inverting amplifier configuration, loop gain is proportional to 1/gain hence the result. \$\endgroup\$
    – ijuneja
    Commented May 26, 2018 at 10:09
  • \$\begingroup\$ Ishank Juneja - sorry, this is NOT true. It is the advantage of the circuit modification to CANCEL the direct connection between loop gain and closed-loop gain. As I have mentioned - you can have a small loop gain (because of stability reasons) and, at the same time, a very small closed-loop gain (unity gain). Please tell me if I am wrong while saying: Addition of a resistor R REDUCES the bandwidth of the closed-.loop gain (due to a loop gain reduction). \$\endgroup\$
    – LvW
    Commented May 26, 2018 at 14:00
11
\$\begingroup\$

Intuitive Answer

Since R attenuates both input and feedback to 0V the internal transistors must use more internal gain to supply an output signal voltage so the input current to Vin(-) cancels and remains a virtual ground. i.e. Vin/Rin=Vout/Rf .

So attenuating Vin to Vin(-) with Rin to R to gnd does not affect outside DC loop gain but the op amp transistors have to use more internal gain to match the output , but at the expense of BW due to fixed GBW.

The outside “DC” loop gain up to attenuated New GBW product ... is what I intended TY @LvW

\$\endgroup\$
3
  • \$\begingroup\$ Oh so when they say fixed GBW, they're not referring to the below gain ? $$A_v = -\dfrac{R_f}{R_1}$$ \$\endgroup\$
    – AgentS
    Commented May 26, 2018 at 8:05
  • \$\begingroup\$ Tony I get it, ty :) Opamp supplies negative voltage to maintain \$V_- \approx 0V\$. But \$V_{in}\$ is trying to pull \$V_-\$ up to \$V_{th}\$ only. Since the output is fixed at \$V_{in}(-R_f/R_1)\$, the opamp has to increase its gain to compensate the decreased input. \$\endgroup\$
    – AgentS
    Commented May 26, 2018 at 8:39
  • \$\begingroup\$ Tony - do you say that the "outside loop gain" is not affected? I think, in contrary - it is the main purpose of this modification (additional R) to reduce the loop gain thereby improving the stability properties of the closed-loop system. The feedack factor - and with it the loop gain - is reduced to (R1||R)/[(R1||R) + Rf] \$\endgroup\$
    – LvW
    Commented May 26, 2018 at 15:49
10
\$\begingroup\$

The shown circuit modification with a resistor R between the opamp input terminals is a very popular method for improving the stability margin of the closed lopp gain (input compensation).

For ideal opamps (very large open-loop gain) the resistor R has no influence on the closed-loop gain but it lowers the LOOP GAIN (and, hence, the bandwidth of the closed-loop gain).

As a result, the stability margin is improved and we are allowed to use even opamps which are NOT unity-gain compensated for applications requiring closed-loop gain values as low as unity.

Intuitive explanation (for uneffected closed-loop gain): Assuming that the open-oop gain Aol is infinity, the closed loop gain is Acl=-Hf/Hr with

Forward factor Hf=Vn/Vin for Vout=0 (Vn: Voltage at the "-"opamp terminal) and

Feedback factor (return) Hr=Vn/Vout for Vin=0.

It is easy to show that the additional resistor R lowers both factors in the same way so that the value of "R" cancels out in the ratio Hf/Hr.

Calculation:

Forward factor: Hf=(Rf||R)/[(Rf||R) + R1]

Feedback factor: Hr=(R1||R)/[(R1||R) + Rf]

After evaluation (and some mathematical manipulations) of the ratio Acl=-Hf/Hr we arrive at Acl=-Rf/R1 (R cancels out).

However, the loop gain (which is essential for stability properties) can be made as low as necessary by varying R:

Loop gain LG=-Hr*Aol (Aol: Open-loop gain of the opamp)

\$\endgroup\$
7
  • 3
    \$\begingroup\$ It's worth to mention that this type of compensation increases the noise gain. \$\endgroup\$
    – Mike
    Commented May 26, 2018 at 8:59
  • 1
    \$\begingroup\$ Mike - yes, that is correct. This effect again shows that - in general - it is not possible to improve one performance parameter without influencing another performance parameter adversely. Hence, each good design is always a trade-off between conflicting effects. \$\endgroup\$
    – LvW
    Commented May 26, 2018 at 9:06
  • \$\begingroup\$ How about the gain accuracy? \$\endgroup\$
    – analogsystemsrf
    Commented May 26, 2018 at 14:59
  • \$\begingroup\$ I think, the gain accuracy is - as always - determined by the tolerances of the passive parts -as long as the finite open-loop gain of the opamp can be set to approximately infinity. \$\endgroup\$
    – LvW
    Commented May 26, 2018 at 15:31
  • \$\begingroup\$ @LvW I feel I get it now. You're using superposition to define forward and feedback factors and the expressions for closed loop gain and loop gain look really neat! Thank you :) \$\endgroup\$
    – AgentS
    Commented May 27, 2018 at 14:05

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.