I've been trying to understand how the dc bias is removed at the ouput inside an op amp. Below circuit seems to work
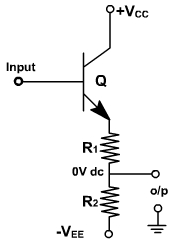
Some explanation is given below by the author
To shift the output dc level to zero, level translator circuits are used. An emitter follower with voltage divider is the simplest form of level translator as shown in fig. 2.
Thus a dc voltage at the base of Q produces 0V dc at the output. It is decided by R1 and R2.
But I don't quite get how R1 and R2 manage to maintain 0V at the output no matter what dc value we put at the Input. As the Input changes, \$V_{CE}\$ of the transistor \$Q\$ varies on the load line, so I don't see how the o/p can stay at 0V. Appreciate any help.
