0
\$\begingroup\$

I am reading this doc.

At one point, the author says the current equation form a critically damped series RLC circuit when a step function is applied is:

\$ I_L(t) = I_{\infty} + (B_1 + B_2t)e^{-\alpha t} \$

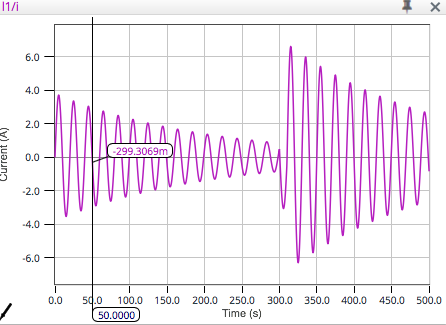
As far as I know, on a critically damped RLC circuit current will oscillate and be like this

enter image description here

\$ I_{\infty} \$ will be an equation, not a value, right?

What is the the meaning of this \$ I_{\infty} \$ on the current equation and how do I find it?

\$\endgroup\$
5
  • \$\begingroup\$ The ringing is decreasing with time. A step function has only one change, at t=0, after than it is constant. The current will approach a single value over time. \$\endgroup\$
    – Spehro 'speff' Pefhany
    Commented Aug 30, 2018 at 13:44
  • \$\begingroup\$ thanks and how do I find it from the equations? \$\endgroup\$
    – Duck
    Commented Aug 30, 2018 at 13:49
  • \$\begingroup\$ If you have the solution to the differential equation, then it's simple, allow t to go to infinity and the exponential term goes to zero. If you don't have the solution, well you know that a capacitor acts as an open circuit after a long time, and an inductor acts as a short. \$\endgroup\$
    – Spehro 'speff' Pefhany
    Commented Aug 30, 2018 at 13:57
  • \$\begingroup\$ so, for a circuit where the capacitor/inductor are both initially discharged current at infinity will stabilize to zero, given the capacitor will be an open circuit, right? Please convert your comment to an answer, so I can accept. THANKS \$\endgroup\$
    – Duck
    Commented Aug 30, 2018 at 13:59
  • 3
    \$\begingroup\$ Critically damped is \$\zeta = 1 \$, or no ringing. \$\endgroup\$
    – Chu
    Commented Aug 30, 2018 at 16:26

1 Answer 1

Reset to default
2
\$\begingroup\$

If you have the solution to the differential equation, then it's simple, allow t to go to infinity and the exponential term goes to zero.

The function you show is underdamped (complex roots), overdamped will have real roots and critically damped has repeated real roots.

If you don't have the solution, well you know that a capacitor acts as an open circuit after a long time, and an inductor acts as a short.

So a parallel RLC circuit will have zero voltage after a long time, and a series RLC circuit will have zero current. Regardless of the (presumed finite) initial conditions.

\$\endgroup\$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.