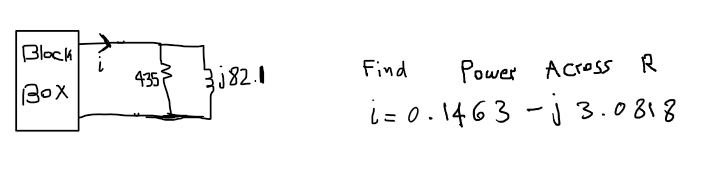Do resistors consume reactive power?

Question

I encountered this problem in one of my books where the problem asked to find the power loss in the core of an induction motor. I worked through the problem and I found the current as in the picture, but it came to my notice that if I find the power across this load and then find the current that passes through the resistor of interest and finally multiply current by voltage the resulted power would be a complex number. But my intuition tells me that this is a big NO. As it is known that resistors cannot store energy, therefore, a complex power doesn't make much sense.

Now, I found in similar problems that people multiply the magnitude of the phasor of each component to find power across the resistor and they simply ignore the phase angle as if it has zero phase degree. But, I am unable to understand why they just assume that a phase does not exist.

Can someone explain it to me in depth?

Answer 1

Do resistors consume reactive power?

No, resistors only consume active power. Also reactive power is not "consumed" - it is stored or returned.

Any "complex current" that would flow through the resistor would generate a voltage across the resistor proportional to this current. The power consumed by the resistor would be \$|I|.R\$ where \$|I|\$ is the magnitude of the current. The current represented as a complex number also provides information about the phase of the current versus the voltage at the frequency that is not mentionned in your example (it is already used to determine the reactance of the inductor).

The example

The complex current in the example must be split in its real part and its reactive part. The real part flows through the resistor and the reactive part through the inductor.

This can be explained by the fact that the current flowing through the resistor does not have a phase shift with regards to the voltage across the resistor terminals - and this voltage is the source voltage itself.

So the power consumed by the resistor is \$63.6405\mathrm{W}\$.

The example, if the resistor and the inductor would be in series

If the resistor and the inductor would have been in series, then the current is not split this way, but it still has an non null imaginary part because the current phase will be shifted with regards the source voltage. The resistor would have consumed as much as indicated in the first part of this answer, i.e. \$1340.60\mathrm{W}\$. In fact, in that case, we only need the magnitude of the current - its phase would be irrelevant.

Side note

It could be argued that the question is incompletely stated as we do not really known what the reference for the current is. We assume that the phase is referenced to the output voltage of the black box and not referenced to the black box's internal ideal voltage source ahead of the black box's internal source impedance (which can be complex itself). If the internal voltage is the reference, then we need more information about the black box output voltage phase (complex voltage like \$5\mathrm{V}+j*.5\mathrm{V}\$).

Answer 2

By KCL, some of the current i flows through the resistor and some through the inductor. You can use the current divider rule to find the current through the resistor. Let's call that \$i_R\$.

Whatever \$i_R\$ is, the voltage across the resistor will be \$(435\ \Omega)i_R\$. If we represent \$i_R\$ as \$I\angle\theta\$, then the voltage will be \$RI\angle \theta\$.

So the phase difference between the resistors current and voltage will be 0.

it came to my notice that if I find the power across this load and then find the current that passes through the resistor of interest and finally multiply current by voltage the resulted power would be a complex number.

This isn't clear. How did you use the power into the load to find the current through the resistor without first separating the resistive and inductive parts? You would have to assume that the inductor is responsible for all reactive power and the resistor is responsible for all real power. Then of course you will have the resistor power being purely real.