DC Black Box and Thenevin Equivalence

Question

There's a DC Black Box, and my goal is find the Thevenin resistance within the black box. By connecting a digital mulitmeter to the terminal, I have found that the "open-circuit" output is \$0.647\ \text{V}\$. Then I connected a variable resistance box and the multimeter in parallel to the black box. Then I increased the load resistance to \$1410\ \Omega\$ till I got 50% of the open circuit reading. Which is \$0.3235\ \text{V}\$.

Then I know that \$V=I\cdot R_{th}\$

I proceeded to find \$I\$ by using the relationship created by the load, \$0.3235 = I\cdot 1410 \rightarrow I = 0.000230780142\ \text{A}\$

Then using this value I solved for \$R_{th}\$

\$0.647 = 0.000230780142\cdot R_{th} \rightarrow R_{th} = 2803 \Omega.\$

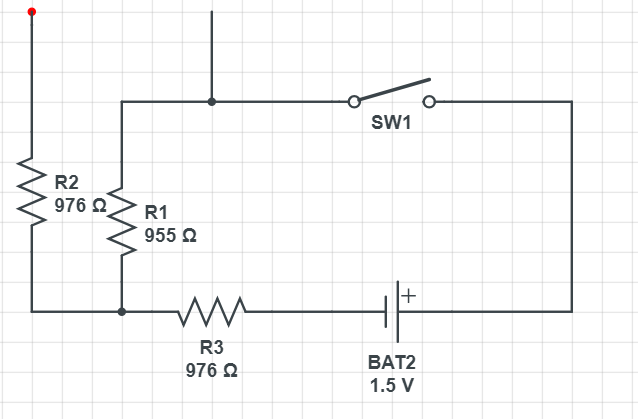
Then I was allowed to check the Black Box contents and the circuit looked like this:

Shouldn't the effective resistance then be, \$R = R_3 + (\frac{1}{R_1} + \frac{1}{R_2})\$?

This doesn't even come close my calculation.

Any help would be greatly appreciated.

Answer 1

To find the Thevenin resistance you divide the open-circuit voltage by the short-circuit current. The current value you used is not the short circuit current. However, it turns out that the resistance value you used (1410 \$\Omega\$) is itself \$R_{TH}\$. See if you can figure out why.

Answer 2

Where do you get \$R_{th} = R_3 + (\frac{1}{R_1} + \frac{1}{R_2})\$?

Hint: Try re-drawing the circuit in the black-box to arrange your open-circuit terminals to be oriented vertically.