Where does the negative sign come from in this transfer function?

Question

I have a transfer function that was given to me. It's been a really long time since I've done block diagram math, so sorry if this is a newbie question.

Based on the block diagram below (I forgot to edit the image, but Tin is the output y, that last arrow)

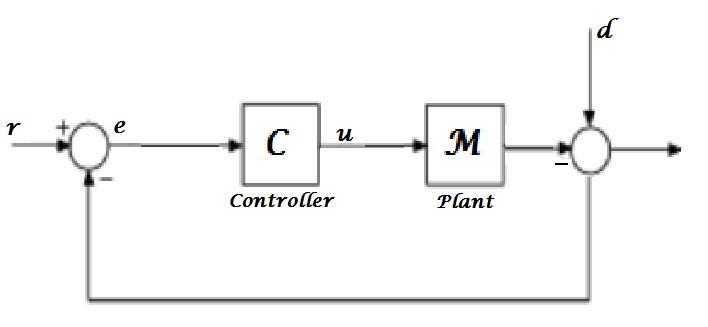
I am give the equations

$$T{_i}{_n} = T{_d}{_i}_s{} - T{_m}{_o}{_t}$$

$$G{_r}{_e}{_f}{\rightarrow}{_i}{_n} = \frac{ -C(s)M(s)}{1 - C(s)M(s)}$$

I can't for the life of me figure out where the two negative signs come from in these equations. Can anybody point me in the right direction as to where these come from?

Answer 1

The output signal: \$\quad y = d-C(s)M(s)e\$

The error signal: \$\quad e = r-y\$

$$ y = d-C(s)M(s)[r-y] $$ $$ (1-C(s)M(s))y = d-C(s)M(s)r $$ From superposition principles, take \$d\$ as 0 and you get the transfer function from \$r\$ to \$y\$: $$ y = \frac{-C(s)M(s)}{1-C(s)M(s)}r $$

As can be seen, the minus sign comes from the first equation. If you were to redefine \$y = d+C(s)M(s)e\$, then the following equation would hold: $$ y = \frac{C(s)M(s)}{1+C(s)M(s)}r $$

Answer 2

If you follow the signal around the loop the plant output goes into a negating input to the right summing junction, then back to the left into a negating input in the left summing junction. Because the loop gain is positive there's a minus sign in the denominator. The forward path only hits a negating input at the right summing junction, which accounts for the minus sign in the numerator.