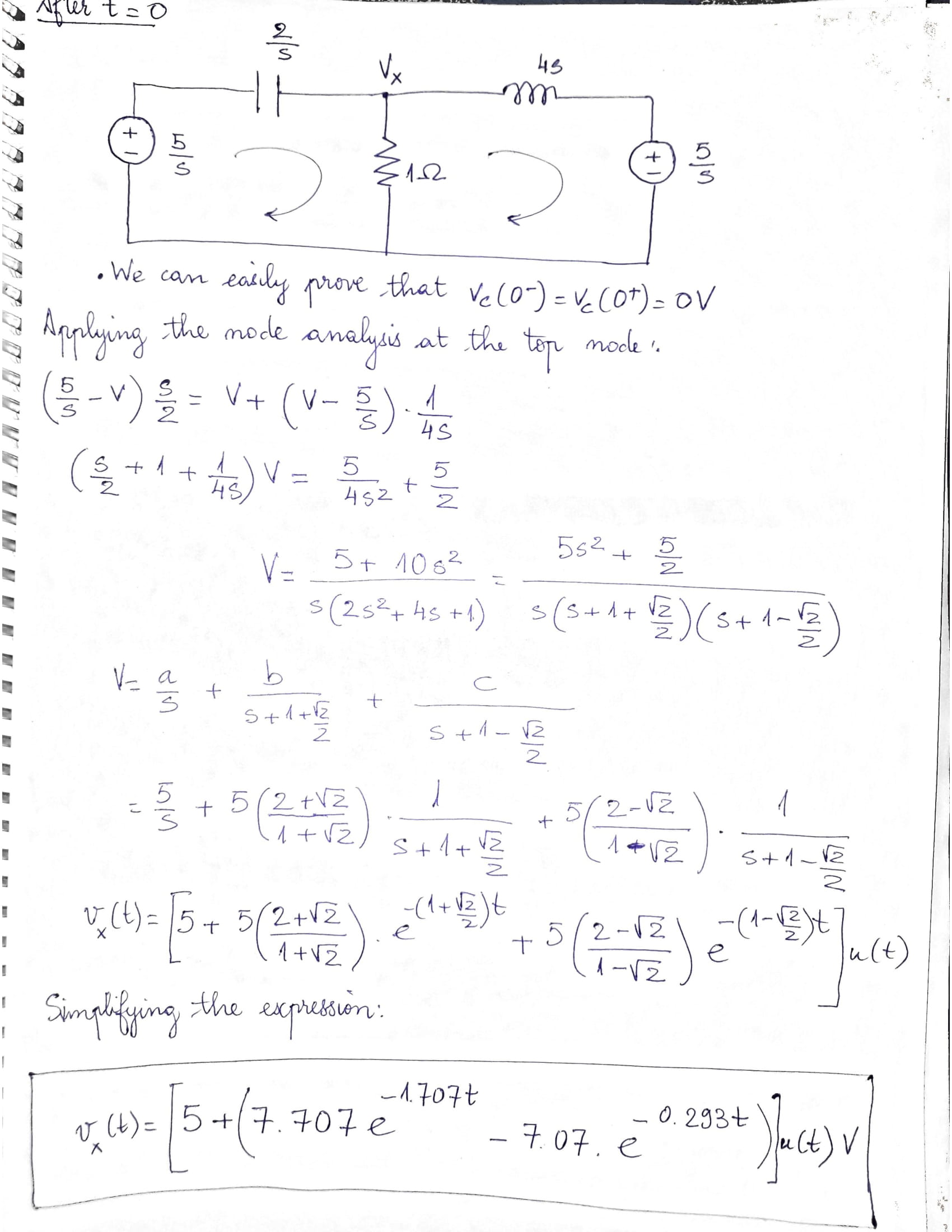
I finally figured out the solution. The key is to firstly find the initial current flowing through the inductor.
For \$t<0\$, the equivalent circuit is the following:
From this circuit, we will calculate the capacitor voltage \$v_c(\infty)\$ and the inductor current \$ i_L(\infty)\$ which will serve as initial stored energy values in the original circuit.
- It is clear that \$v_c(\infty)=0\text{ Volts}\$
- Mesh analysis on the two loops will yield \$i_L(\infty)\$
From Mesh 1:
$$\begin{align*}
\frac{1}{s} &= I - I_L +\frac{2}{s}I\\
\frac{1}{s} &= \left(1+\frac{2}{s}\right)I - I_L
\end{align*}$$
From Mesh 2:
$$\begin{align*}
\frac{1}{s} &= I - I_L -\left(4s\right)I_L\\
\frac{1}{s} &= I - \left(1+4s\right)I_L
\end{align*}$$
The linear system is the following:
$$
\begin{cases}
\left(1+\frac{2}{s}\right)I - I_L &= \frac{1}{s}, &\text{from Mesh 1}\\
I - \left(1+4s\right)I_L &= \frac{1}{s}, &\text{from Mesh 2}
\end{cases}
$$
Solving the system, we end up with the expression of \$I_L\$:
$$\begin{align*}
I_L &=\frac{{}^{-1}{\mskip -5mu/\mskip -3mu}_2}{s\left(s^2+2s+{}^1{\mskip -5mu/\mskip -3mu}_2\right)}\\
I_L &= \frac{{}^{-1}{\mskip -5mu/\mskip -3mu}_2}{s\left(s+1+\frac{\sqrt{2}}{2}\right)\left(s+1-\frac{\sqrt{2}}{2}\right)}
\end{align*}$$
Proceeding with the decomposition of the rational expression:
$$
I_L=\frac{-1}{2}\left(\frac{a}{s}+\frac{b}{\left(s+1+\frac{\sqrt{2}}{2}\right)}+\frac{c}{\left(s+1-\frac{\sqrt{2}}{2}\right)}\right)
$$
where \$a,b,c\$ are real numbers. Taking the inverse Laplace trasform of \$I_L\$:
$$\begin{align*}
\mathcal{L}^{-1}\{I_L\}=i(t)&=\frac{-1}{2}\left(a+b e^{-\left(1+{}^{\sqrt{2}}{\mskip -5mu/\mskip -3mu}_2\right) t} +c e^{-\left(1-{}^{\sqrt{2}}{\mskip -5mu/\mskip -3mu}_2\right) t}\right) u(t)\\
i(\infty)&= \frac{-a}{2}
\end{align*}$$
Identifying \$a\$, we find that:
$$
a=2 \quad \text{and}\quad i(\infty)=-1 \text{ A}
$$
Now, in the second part at \$t\geq 0\$, we return to the intial circuit which becomes as:
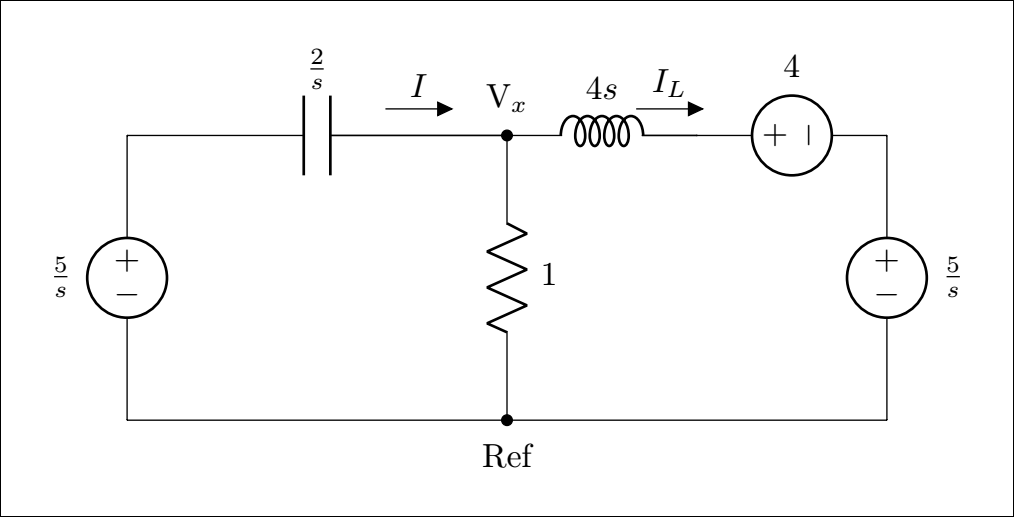
Calculating \$V_x\$:
$$\begin{align*}
\left(\frac{5}{s}-V_x\right)\frac{s}{2} &=V_x+\left(V_x-4-\frac{5}{s}\right)\frac{1}{4s}\\
\left(\frac{5}{s}-V_x\right)\frac{s}{2} &= \left(1+\frac{1}{4s}\right)V_x-\left(\frac{1}{s}+\frac{5}{4s^2}\right)\\
V_x &= \frac{10s^2+4s+5}{s\left(4s+2s^2+1\right)}\\
V_x &= \frac{5s^2+2s+{}^{5}{\mskip -5mu/\mskip -3mu}_2}{s\left(s+1+\frac{\sqrt{2}}{2}\right)\left(s+1-\frac{\sqrt{2}}{2}\right)}
\end{align*}$$
Decomposing the rational expression:
$$
V_x=\frac{d}{s}+\frac{e}{s+1+\frac{\sqrt{2}}{2}}+\frac{f}{s+1-\frac{\sqrt{2}}{2}}
$$
where \$d,e,f\$ are real numbers. Identifying them, we find that:
$$
d=5, \qquad e=4\left(\frac{2+\sqrt{2}}{1+\sqrt{2}}\right), \qquad f=4\left(\frac{2-\sqrt{2}}{1-\sqrt{2}}\right)
$$
Taking the inverse Laplace transform:
$$
\mathcal{L}^{-1}\{V_x\}=v_x(t)=\left[5+4\left(\frac{2+\sqrt{2}}{1+\sqrt{2}}\right)e^{-\left(1+{}^{\sqrt{2}}{\mskip -5mu/\mskip -3mu}_2\right)t}+4\left(\frac{2-\sqrt{2}}{1-\sqrt{2}}\right)e^{-\left(1-{}^{\sqrt{2}}{\mskip -5mu/\mskip -3mu}_2\right)t}\right]
$$
Finally, simplifying the expression:
$$
v_x(t) =\left(5+5.657e^{-1.707t}-5.657e^{-0.293t}\right)u(t) \text{ V}
$$