Capacitive crosstalk switching frequency vs rise/fall time

Question

I'm breaking my head over the frequency dependent behaviour of capacitive crosstalk. Time domain analysis seems to conflict with frequency domain analysis, obviously one must be wrong.

Let's assume we have 2 square waves, one of 1MHz and one of 10MHz, both with rise and fall times of 10ns.

1) Frequency analysis will lead me to the conclusion that crosstalk will become worse with increasing frequency. This seems to be wrong (simulation) but I don't know why. If we decompose the square wave into harmonics, the 3rd and 5th harmonics are also 1/3 and 1/5 in amplitude and so on. So the higher harmonics (around 100MHz for example) of the 1MHz square wave are lower in amplitude than the higher harmonics (around 100MHz) of the 10MHz square wave. The lower amplitude of the higher harmonics should result in lower crosstalk as the impedance of the parasitic/coupling capacitance decreases with frequency.

2) According to time domain analysis, the crosstalk only depends on rise and fall times, and not on switching frequency. I=coupling C*(delta voltage /delta time), delta time is the signal amplitude and delta time is the rise/fall time, hence no frequency dependent behaviour. It's all caused by the rise/fall time here. 'I' will cause a noise voltage over the victims impedance (R1 in simulation).

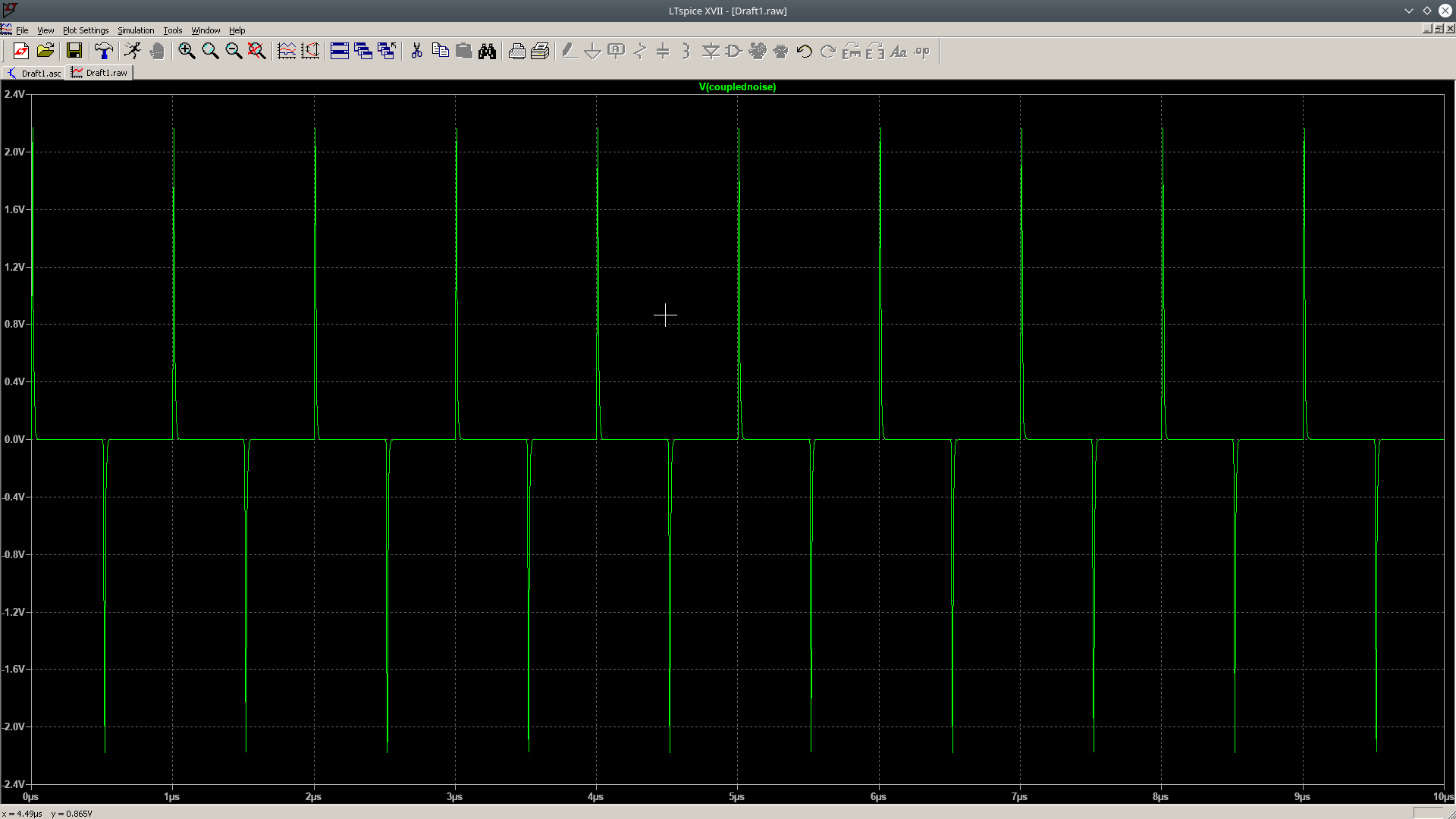
3) Simulation 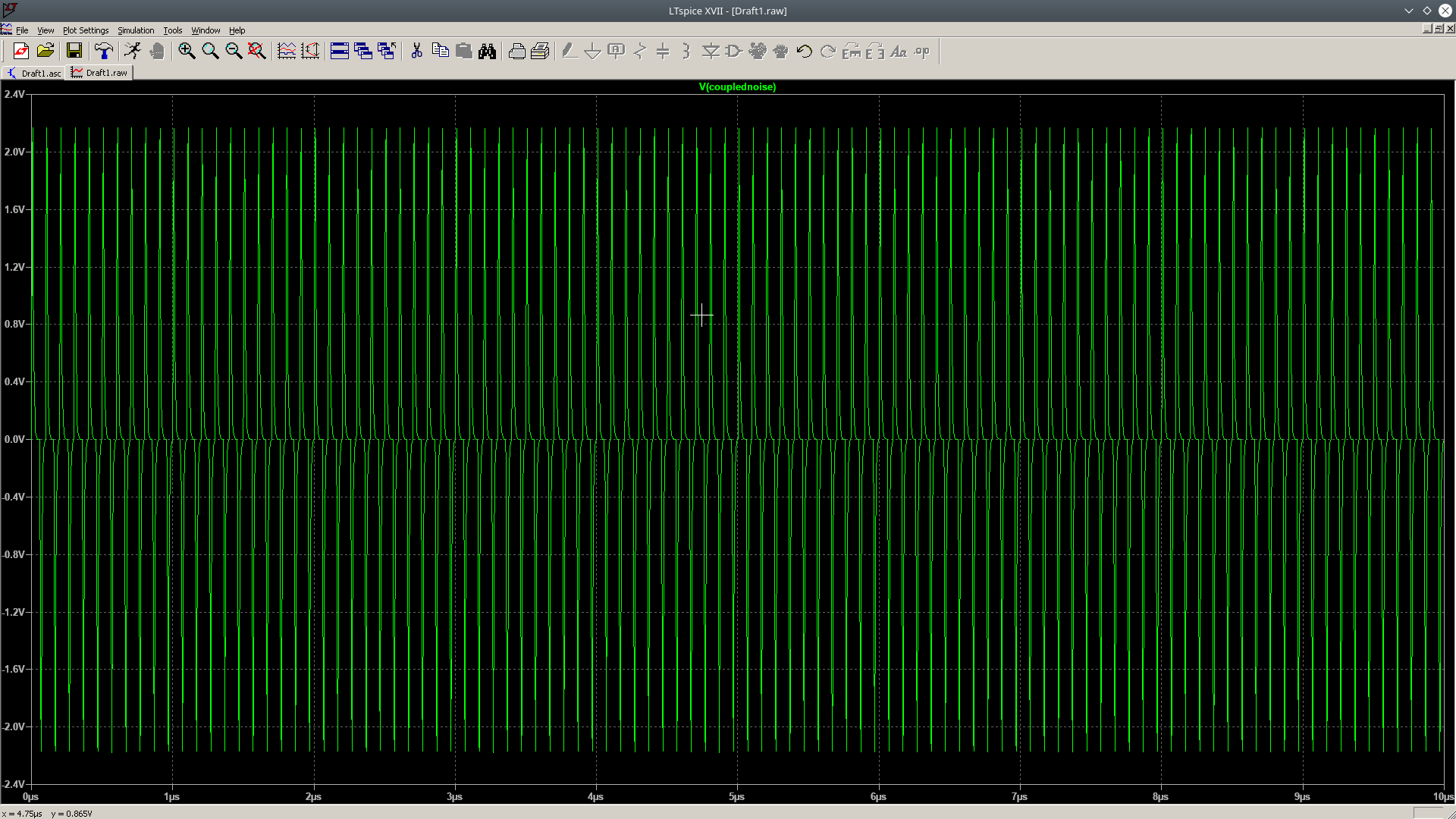As we can see here, the crosstalk amplitude is the same in both cases, so there must be something wrong with my frequency domain analysis.

Can someone help me out here?

Answer 1

Let's assume we have 2 square waves, one of 1MHz and one of 10MHz, both with rise and fall times of 10ns.

And this is contradicted by this: -

If we decompose the square wave into harmonics, the 3rd and 5th harmonics are also 1/3 and 1/5 in amplitude and so on.

For perfect square waves that would be true but you have told us that you are using waveforms with real numerical rise and fall times of 10 ns and, as you approach frequencies like 100 MHz the results won't be as clear cut.

But generally the individual harmonics of the 1 MHz waveform at (say) 49 MHz will be much lower than the 5th harmonic of 10 MHz (50 MHz) but, harmonics are occuring much more often with the 1 MHz waveform (i.e. 47 MHz, 49 MHz, 51 MHz etc.) and this also needs to be factored in.

In short, your conclusions are faulty because you are not considering the bigger picture in the frequency domain. Analysing the crosstalk from the perspective of rise and fall times is the clear-cut method as you have demonstrated.