How can an electron have 0 electric potential after exiting a resistor but have current?

Question

Unfortunately, I am not able to respond and ask a question on this specific post so I am going to ask a question based on the answers of the user "Transistor" in this post because I am still confused.

https://electronics.stackexchange.com/a/217000/211088

All my statements are based on the assumption that electrons have 0 electric potential after they go through the last load or is at the end of the circuit. (Statement # 2).

Please tell me if these statements are correct:

1. Electric Potential is the force created from all the electrons in the negative terminal of the battery. The force is created from the electrons pushing away from each other trying to go to the positive terminal which also pulls the electrons.

So does this create 2 times the force because of an electron's push and the positive terminal's pull? Or does the push and pull just the electric potential? I'm confused about this part of statement 1.

2. If I have one battery and one resistor only, from what I understand is that after electrons exit the resistor, they have 0 electric potentials.

3. My understanding based on the first answer of "Transistor":

It's getting pushed by the potential difference in other parts of the circuit.

This statement is referring to electrons in between resistors.

After the electrons exit the resistor, even though they have an electric potential of 0, they still flow to the positive terminal because of the fact that the electrons currently flowing through the resistor has an electric potential, therefore, it needs to move forward and exit the resistor which pushes the electrons already out of the resistor towards the positive terminal. Doesn't this mean that the electrons out of the resistor already has an electric potential because the electrons in the resistor provide a force for them? Aren't they have 0 electric potentials at the end of the circuit?

4. In figure 2, in the post that I linked above, when the user "Transistor" replies

What's driving the current is the potential difference between the top of the tank (battery +) and the open end of the pipe (battery -).

Does this mean that after the electrons exit the resistor, the negative terminal is no longer apply a force or push but now the positive terminal is applying a pull force? So if I place a voltmeter on each side of the resistor, it reads the voltage of the negative electric potential. Is this why electrons are still able to flow to the positive terminal even though it has 0 electric potential? Basically the same question as statement 1. Can someone explain the quote above.

Sorry for bad format, this is my first post.

Answer 1

If you want to think about the force electrons experience when they are moving in a current carrying conductor, you should not think in terms of voltage, instead electric field is what is forcing the electrons to move (and what is creating the potential difference). In terms of electric field, the Ohm's law can be expressed as $$j = \sigma E,$$ where, \$j\$ is the current density (per unit area), E is the electric field and \$\sigma\$ is the conductivity. The resistors have a finite conductivity, hence a finite current (through resistor) requires it to have an electric field to force the electrons move in one direction. Due to this field, each electron will experience a force qE and will drift slightly in the direction of force (which is what is called current).
Where does this field come from?
Could come from a battery connected across the terminals of resistor.

Now, assuming the resistor is connected to the battery by an ideal wire having infinite conductivity, from Ohm's law you can see that even if the electric field is zero, you can still have a finite current density. A zero electric field means there is no potential drop across the wire but it still can have finite current.
In reality, the wire does have a finite but very high conductivity so a small electric field (and consequently potential difference) is sufficient to drive current through it. This potential difference can be neglected for practical purposes.

Answer 2

I'll draw a picture of a single battery with a single resistor, connected together with wiring. The diagram below is what things look like after the steady-state surface charges have been established (this takes a very small period of time that is difficult to measure.)

The highly conductive wiring itself is filled uniformly with conduction band electrons. For copper at room temperature this is about \$n=8.49\times 10^{22}\,\frac{\text{electrons}}{\text{cc}}\$. In short, a lot. However, the battery adds some electrons to the wiring on the left side and subtracts some electrons from the wiring on the right side. This slight difference occurs at the surface of the copper wiring. The conduction band electrons repel each other, of course, such that their density throughout the metal is roughly uniform. It is only at the surface (which transitions to the insulator or air or vacuum) that there is a slight density difference.

The density of charges shown is roughly qualitative. So on the left side you see lots and lots of (-) charges on the wiring there. This merely represents the surface charge density. Similarly, on the right side you see lots and lots of (+) charges.

The copper wire has very little resistance so the surface charge density close to the battery terminals is almost exactly the same as it is nearer to the resistor (thin wire, as shown), itself. It is only at the resistor that you see a rapid transition. So the gradient is very low in the highly conductive wiring, but higher through the resistor.

---

At first, prior to establishing steady state (before applying the battery to the circuit), the wiring and the resistor are neutral and the surface charges are similar throughout the entire system (wire + resistor + more wire.) But the moment the battery is connected, the electrons per second entering from the negative end of the battery into the wire is large (\$n\,A_\text{wire}\,\overline{v}\$) and similar to the number of electrons per second leaving the wire and into the positive end of the battery. But at the resistor (represented by a very thin wire here), fewer can move through at first (\$n\,A_\text{resistor}\,\overline{v}\$) and so electrons "pile up" on the negative end (entrance) of the resistor. (Similarly, some of the mobile electron charges are removed from the exit end of the resistor, as they travel towards the positive end of the battery. You can think of this as a "pile up" of positive charges, I suppose. But the details are beyond the scope of what I want to write here.)

Once steady state (as shown in the picture) is achieved, which happens very very quickly, the currents throughout must all be the same. They must be equal in the wiring connected to the (-) end of the battery, equal in the wiring connected to the (+) end of the battery, and equal in the resistor. (If the currents weren't the same in the steady state then surface charge would build up on the resistor and, of course, that would mean we still haven't reached steady state.)

The charges that have piled up on either end of the resistor provides a high enough drift speed within the resistor such that the net current in the resistor equals the current in the wires at either end. And now that the currents are the same in the wires as in the resistor, no more charges pile up to further increase the drift speed in the resistor.

In this analogy, I've used a very thin wire for the resistor using the same material (copper, let's say) for the resistor as for the wiring. This simplification allows another conclusion by very simple reasoning. The electric field (volts per meter) in the resistor must be quite a lot larger than the electric field in the wiring (assuming the materials are identical) since the mobile electron density is identical throughout and the electron mobility is identical throughout. Given that, it must be the case that the electric field is quite different between the resistor and wiring.

Of course, real resistors aren't made of the same material. So the above statement needs to be somewhat nuanced. But in all cases this relationship must be true: $$n_\text{wire}\,\mu_\text{wire}\,A_\text{wire}\,E_\text{wire}=n_\text{resistor}\,\mu_\text{resistor}\,A_\text{resistor}\,E_\text{resistor}$$

(\$E\$ is the electric field in volts per meter, \$\mu\$ is the mobility, \$n\$ is the mobile electron density, and \$A\$ is the cross-section area.)

---

Looking at the above picture I provided, you can see that surface charge neutrality only occurs in the center of the resistor. If the battery were to be replaced by a high voltage supply, the surface charges that have piled up towards the positive end of the resistor (or negative) would be sufficient that a very light, neutral ball (pith ball, for example) would be initialy attracted to the charges, stick momentarily to the wire while transferring some of the surface charges to the pith ball, and then suddenly repelled away by the like charges. (Suspended near the center of the resistor (which is neutral) if long enough to do so, nothing much would happen.) Unfortunately, at the more commonly found voltages in everyday systems, there's not enough surface charge for the effect to be detected in this way.

---

Now, as a thought experiment to try out, take the above picture and insert another resistor somewhere in it and then sketch out the charge densities after thinking about the above descriptions.

---

For those interested in a somewhat more detailed discussion, as well as more pictures, please go to page 766 (in Chapter 19) of the 3rd edition of *"Matter & Interactions," by Chabay and Sherwood.

Answer 3

All my statements are based on the assumption that electrons have 0 electric potential after they go through the last load or is at the end of the circuit.

That's not what "potential" means. Individual electrons cannot "have potential," since potentials are always measured between two points. Depending on your choice of the other point, a single electron can have many different potentials, all at the same time. (It can have an infinite number of potentials. It can even have negative potential, so would that mean it's carrying negative energy? No, that's not how potentials work.)

Huh, is it big-wall-of-text time again? Guess so!

---

This problem becomes far easier to understand if we pretend that electric potentials are like altitudes.

When the babbling brook has passed over the wooden waterwheel, and the water falls downwards, and some work on the wheel has been performed, and the millstones have ground some wheat into flour ...are the exiting water-molecules now at zero altitude? No, since a molecule of water cannot "have altitude," and besides, "zero altitude" does not exist. Altitude is always measured between two points, and we get to choose the second point. If our altitude-reference is up at the water-level above the waterwheel, then the downstream exit is at negative altitude. Does this mean that the water molecules must have sucked energy out of the waterwheel? Has some negative work been performed, as the millstones ground the flour? No, that's just silly, and it rubs our nose in the cause of the misunderstanding.

To understand the waterwheel, we need to stop thinking about absolute altitudes or about "energy stored inside a water molecule." Instead, the stored potential-energy is out in the system as a whole. (Actually it's stored in the gravity fields of the whole landscape, and not stored inside the water molecules of our babbling brook.) When we lift a liter of water higher above ground, we aren't injecting some sort of strange energy into the inside of each H2O molecule. Instead, we're stretching the gravity fields. The water molecules remain exactly the same, whether they're up in orbit, or sitting on Mt. Everest, or down in the Mariana Trench. Looking at individual molecules tells us nothing about gravitational potential energy. The energy is stored in the empty space, in the attractive fields between the molecule and the planet. In a certain way, the "height" itself is the energy. (Well, height-and-a-lifted-mass.) The potential energy is found in the empty space where the altitude exists. But also, if we lift two molecules rather than one, we double the stored potential energy, while the altitude stays the same. Potential energy is a strange combination of mass as well as altitude. The energy is stored in the system as a whole, not inside the mass-particles being lifted.

And, similar is true of electrons.

Electrons don't "have potential," and they cannot store energy inside themselves. The energy is stored in the surrounding field-patterns. Electrical energy travels just outside the wires. Not inside the copper. For electronics, "altitude" becomes "altitude in an e-field," rather than the lift-height in a gravity field. With circuits, their stored energy ends up in the EM fields outside the wires. When coils store energy, it's stored in their magnetic fields, and when capacitors store energy, it's stored in their e-fields. With circuits, both of these are happening at the same time.

Closed water-loops are weird in another way.

If our wooden waterwheel is powered by a distant water-pump, where the pump lifts the exit-water back up into the upper brook, then what happens if we choose the upper brook as our height reference? In that case all the energy is being delivered by the lower exit-stream! It's flowing backwards, and at negative height. Multiply the flow rate by the height to obtain the watts of energy-flow. Negative times negative gives a positive, so we find that energy is going from pump to waterwheel, and entirely flowing through the lower brook.

Yet if instead we choose the lower brook as our height-reference, then all the energy is flowing in the upper brook, and none in the lower! (Heh. Or, if we choose our height-reference at the midpoint, at the axle of the waterwheel, then our calculations will "prove" that each brook delivers half the energy-flow.)

Where then is the true location of the flowing hydraulic energy?

:)

This is much the same problem with circuit-energy. The electrical energy isn't inside the movable charges in the conductors. And, the energy isn't all flowing inside one wire, with zero energy in the second wire (because energy cannot flow inside metals in the first place; instead it all flows in the EM fields outside the wire surfaces.)

Here's one way to cut through the confusion.

Start with two long wires. They're full of protons and electrons, equal amounts, to give neutral net-charge. Now move some charges from one wire to the other. This means that one wire is now negative, the other positive, and energy has been stored in the e-fields extending between them. The two wires have become the two plates of a loooonnng capacitor. When we move some charges, the e-fields spread along the wires at light-speed, and occupy the entire space surrounding the two parallel wires. Notice that if our charge-pumping was performed at one end of the wire-pair, energy is now available at the other end! A long capacitor is a method for transmitting e-field energy.

Next, connect a resistor at the far end of the wire-pair. This "discharges" the capacitor and heats the resistor. The e-field energy found in the space between the wires will all rush into the resistor (it propagates at the speed of light.)

Which wire delivered the flow of energy? Both, obviously. Or neither, since the energy remained entirely outside the metal. There were no "energized" electrons or protons here ...any more than lifting a rock off the ground can create "energized" silica molecules. Instead, we injected energy into the whole "capacitor." Then, the resistor took energy out of the whole "capacitor." The energy traveled as EM waves at lightspeed.

Forest versus trees. If we "zoom in" and look at just one wire, that means we've started ignoring the system-as-a-whole, and we've gone up a conceptual dead-end.

The energy was stored in the entire 2-wire capacitor, and not in one of the wires, and certainly not inside individual electrons.

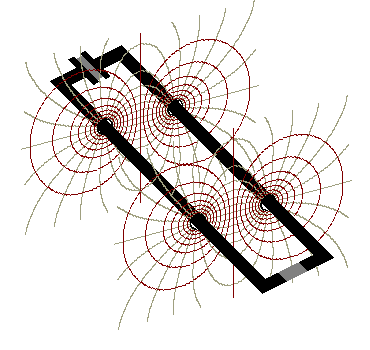
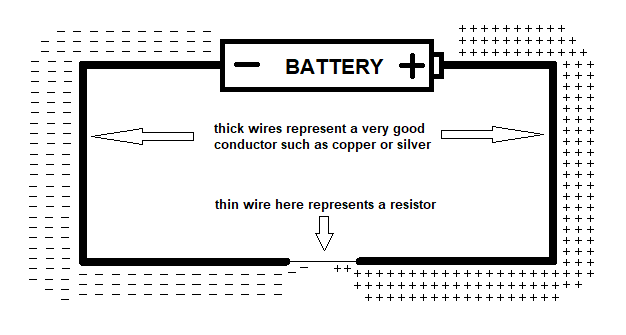
BELOW: a battery, a resistor, and the EM energy-flow in the electric circuit. Red is the magnetic field, grey is the e-field. The circuit as a whole delivers energy from battery to resistor. (The "entire forest" does it. Individual "trees" do not!)