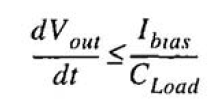Calculation of the slew rate of an inverter with active load

Question

I'm studying the inverter with active load, whose scheme is the following:

Now, the author of my book says:

"We have to ensure a proper large-signal driving of the output node. The load of the inverter is always a capacitance (otherwise, a finite resistance would dissolve the voltage gain) but during transients, the circuit must provide a drain or sink current to charge or discharge that capacitive load. When the current is provided by the input transistor we have no limitations. In contrast, when the current is furnished by the active load, we have an upper limit. It is given by the current generated by the active load itself. This, in turn, sets the slew rate limit

where Cload is the total output capacitance"

I would appreciate if someone can give me some hints on how to calculate the slew rate. Suppose I give a large swing at the input voltage, then what kind of reasoning should I do in order to calculate the slew rate for this circuit? Why do we have that "When the current is provided by the input transistor we have no limitations. In contrast, when the current is furnished by the active load, we have an upper limit"?

Thank you

Answer 1

$$\text{Slew rate} = \frac {\text{current going out from the circuit}} {\text{capacitance at the output}}$$

The output current is the difference of the currents of the input transistor minus the I_bias. It is a function of the load capacitance.

The formula you copied here is valid only for rising edges on the output. Since I_bias is smaller than the maximum current of the input transistor, this slew rate is worse than the falling one, and hence some might consider this value as the "slew rate" of the circuit.