Digital Logic Boolean Algebra

Question

I am having a hard time understanding why A'+B' is not equal to the following expression:

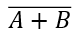
I understand that when I apply the 4 different combinations: 00,01,10,11 to A and B I get different results for reach expression when we have either the combination 01 or 10.

Is that the only explanation? For some reason I always figured the two expressions would yield the same results.

Answer 1

Try writing it out:

        _  _    _   _             _____
A  B |  A  B    A + B    A + B    A + B
-----+----------------+-----------------
0  0 |  1  1      1   |    0        1
1  0 |  0  1      1   |    1        0
0  1 |  1  0      1   |    1        0
1  1 |  0  0      0   |    1        0

\$ \bar A + \bar B \$ is true if either A is false OR B is false.

\$ \overline {A + B} \$ is true if both A and B are false.