I came across this problem for the first time when I started thinking about the clever circuit of Howland current source (see What is the brilliant idea behind the Howland current source?). Let me explain what this is all about with a simple example.
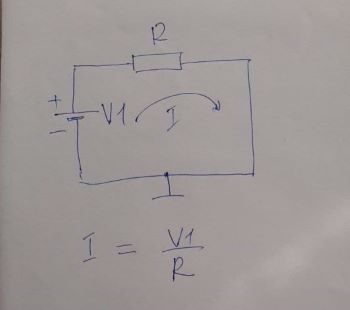
Imagine a simple current source assembled by a voltage source V1 and resistor R. At best, it would be short connected, and the current would be simply I = V1/R.
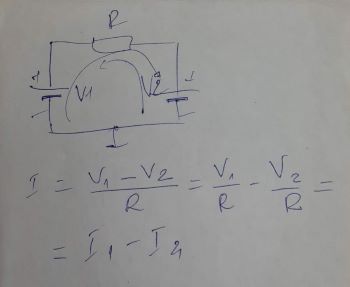
Unfortunately, it is loaded by another voltage source V2 with lower voltage... and the current decreases. We can explain why in two ways - in terms of voltages and in terms of currents.
First we can say that, according to KVL, the effective “current-creating” voltage VR = V1 - V2 across the resistor R decreases… аnd this decreases the current I = (V1 - V2)/R. That is, the current decreases since another opposing voltage is subtracted from the initial voltage.
But with the same success we can say that, according to the superposition principle, the current decreases since another opposing current I2 = V2/R is subtracted from the initial current I1 = V1/R.
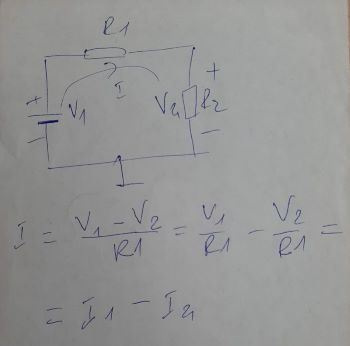
If we replace the voltage source V2 with a resistor R2, we can continue to think the same way… but in the second case we will not be able to make physical sense of what is happening.
That is right, because the superposition principle assumes at least two sources… and here there is only one. The load voltage V2 cannot create current I2 = V2/R1 since it is zero when V1 is zero. That is, this statement makes no physical sense.
At the same time, the principle of superposition is formally valid… and this is the paradox…
I still have an idea of how to see physical meaning. If we measure V2 and replace the resistor R2 with a voltage source with the same voltage, nothing will change… but it will make some physical sense.