0
\$\begingroup\$

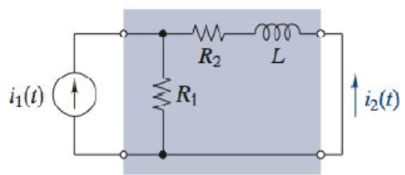
If I have the following circuit:

enter image description here

I know that I can find the transfer function by doing voltage division. However, in this example, where does the negative sign in front of R1 come from? $$T(s)=\frac{-Z_2(s)}{Z_1(s)+Z_2(s)}=\frac{-R_1}{R_1+R_2+L \cdot s}$$

\$\endgroup\$
4
  • \$\begingroup\$ I would say because of \$i_2\$ direction compared to that of \$i_1\$? To stick to a low-entropy format, you can factor \$\frac{R_1}{R_1+R_2}\$ which is your dc gain (\$s=0\$) while the denominator will be in the form of \$1+\frac{s}{\omega_p}\$. Your transfer function correctly expressed is thus \$T(s)=-T_0\frac{1}{1+\frac{s}{\omega_p}}\$ \$\endgroup\$
    – Verbal Kint
    Commented Nov 2, 2019 at 18:38
  • \$\begingroup\$ @VerbalKint But why don't we put a negative sign in front of R2 and L then? \$\endgroup\$
    – user164324
    Commented Nov 2, 2019 at 18:48
  • \$\begingroup\$ If you simulate this circuit, \$i_2\$ circulates in the same direction as \$i_1\$, it's leaving \$L\$. So its value is \$R_1.i_1\$ divided by the sum of \$R_1\$ plus the impedance made of \$R_2\$ and \$sL\$ in series. If you decide to consider \$i_2\$ in the opposite direction, you add a minus sign in front of its definition. \$\endgroup\$
    – Verbal Kint
    Commented Nov 2, 2019 at 20:24
  • \$\begingroup\$ How does voltage division relate to this question? \$T\small (s)\$ is (output current)/(input current). \$\endgroup\$
    – Chu
    Commented Nov 2, 2019 at 20:58

1 Answer 1

Reset to default
0
\$\begingroup\$

If you apply the current divider formula, and observe the direction of \$i_2\$, the result is:

\$T(s) = -\frac {Z_T}{Z_2}\$ where

\$Z_T =\frac{R_1(R_2+sL)}{R_1+R_2+sL}\$ and

\$Z_2 = R_2 + sL\$

so

\$T(s) = -\frac {R_1}{R_1+R_2+sL}\$

The negative sign only arises because \$i_2\$ is defined in the opposite direction to \$i_1\$.

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.