Am I right thinking a resonance circuit sustains oscillation and a tank circuit oscillation dies out
Both the ideal LC circuit and the oscillator tank (as you call it) are resonant circuits. A circuit is called in resonance if the frequency of the driving signal is same as the natural frequency of the circuit.
For an ideal LC circuit (shown below), the natural frequency is \$\omega_0 = \frac{1}{\sqrt{LC}}\$. If this circuit is driven with a current source at this frequency then the circuit will be in resonance. At resonance, the voltage across the capacitor (or inductor) is going to have infinite amplitude. This is because the impedance of the circuit between node A and B, given by \$\frac{1}{sC+\frac{1}{sL}}\$, is infinite.
Further, since there are no losses in the system the circuit can sustain oscillations at the natural frequency.
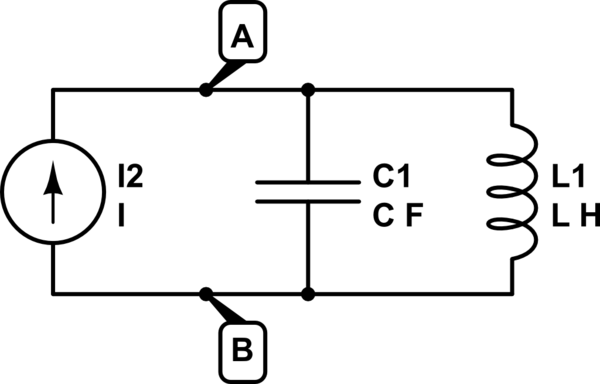
simulate this circuit – Schematic created using CircuitLab
Practically, there cannot be circuit with infinite impedance. And in reality, the inductors and capacitors have parasitic resistances which can be approximately modelled as a resistance parallel to the LC circuit close to the resonant frequency. This ensures that at the resonant frequency the impedance is finite (and equals \$R\$).
But the presence of the R implies losses inside the system and consequently this circuit will not sustain oscillations which will eventually die out.
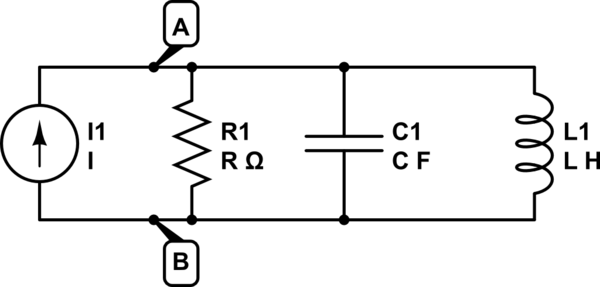
simulate this circuit
To compensate for these losses usually a non-linear active element is placed in parallel with the tank which would compensate for the losses inside the lossy LC tank (due to R).
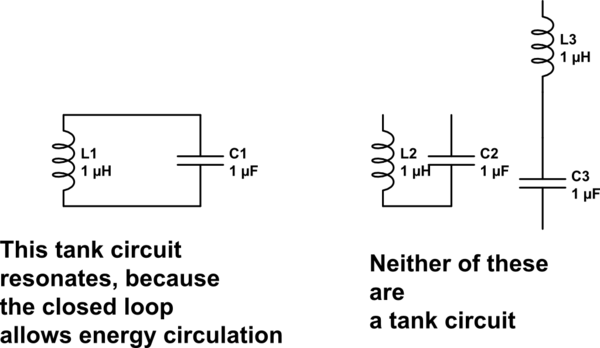
what's the difference between these two circuits?
Both the circuits are parallel resonant LC circuits, ideal LC has no loss while oscillator tank has some loss.
Hopefully, it is clear. Let me know if not.