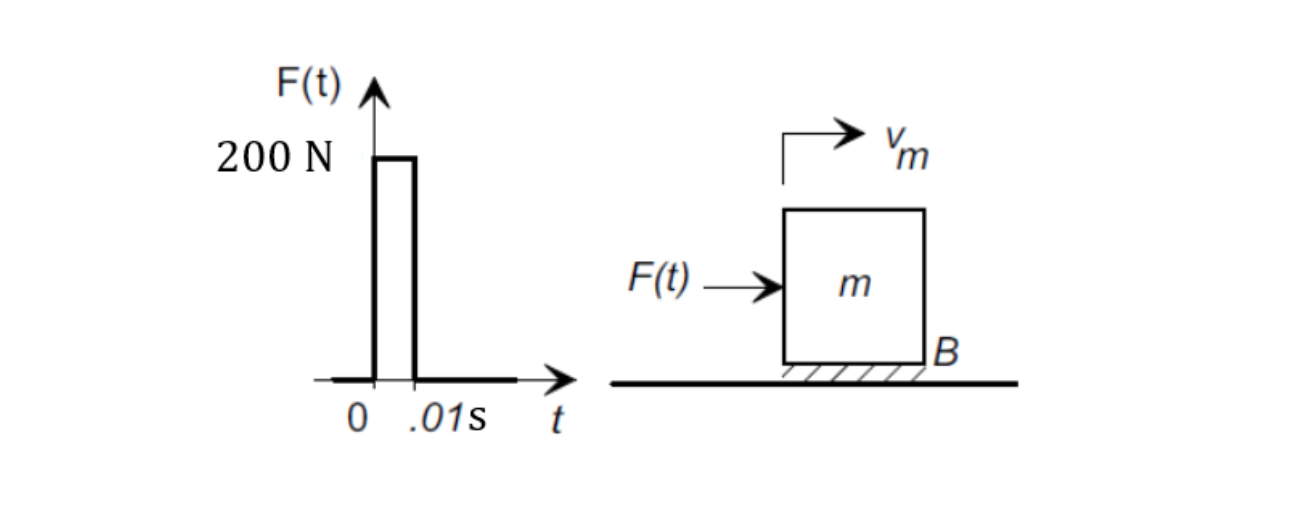
I'm doing a practice problem from https://6002.catsoop.org/F19/ex11/mechanical_analog
The problem is as follows: A mass m = 10 kg is at rest on a horizontal plane with viscous friction coefficient B = 20 N-s/m (which creates a damping force proportional to the velocity \$v_m\$). A short impulsive force of amplitude 200 N and duration 0.01 s is applied
 The electric circuit analog is as follows:
The electric circuit analog is as follows:
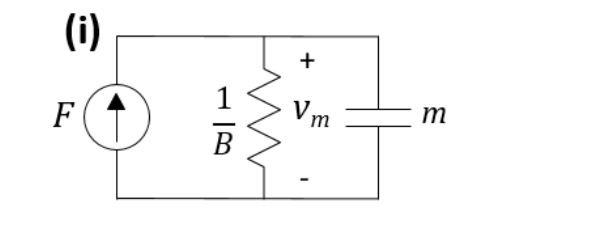
Ultimately, I want to find what the voltage across the capacitor is(aka the velocity). I'm planning to use the formula for RC circuits: $$v_c = v(final) - [v(init) - v(final)]e^{-t/\tau}$$ where \$ \tau = m/B\$ in this case Substituting in values, \$ \tau = (10kg)/(20Ns/m)\$ Now all I need to do is find \$v(init)\$ and \$v(final)\$
A capacitor is going to look like a short at the very beginning so the voltage across the capacitor is going to be zero, \$ v_c = 0\$ while a capacitor towards the end will look like an open circuit. When the capacitor is an open circuit, the voltage across it will just be the voltage across the resistor. So,
$$v_c(final) = F*\frac{1}{B} = 200N * \frac{1}{20} = 10$$
As a result, my answer should be
$$v_c(t) = 10 - 9.8*e^{-2t}$$
However this doesn't seem to be the answer, where did I go wrong?
