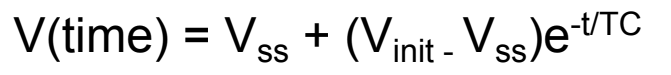1
\$\begingroup\$

I have been given this question by one of my lecturers and have been having difficulty with it. I was wondering if anyone could help me.

In the circuit of Fig. 1, switch SW1 has been closed, and switch SW2 has been open, for a long time. Capacitor C2 has no initial charge. Now, S1 is opened; then, at time, t = 0, S2 is closed.

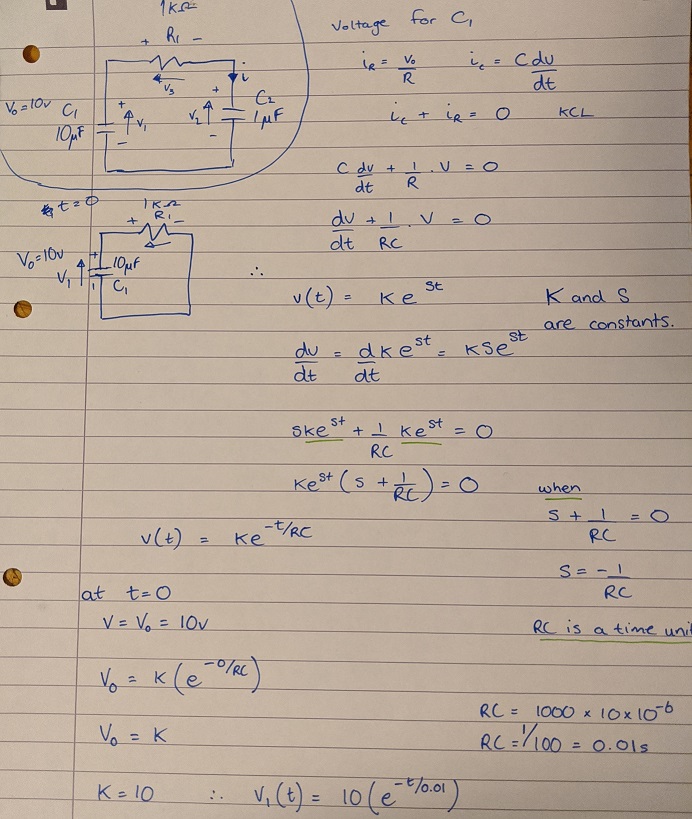
a) Determine the energy stored in C1 for t<0

b) Derive expressions for the capacitor voltages, v1(t) for C1 and v2(t) for C2, for t>0

c) Explain if there is any stored energy in the capacitors as t approaches infinity?

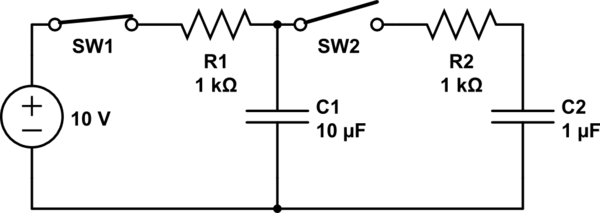
schematic

simulate this circuit – Schematic created using CircuitLab

Thank you for all your help guys. The main issue I'm having is deriving the equations for the voltages across each capacitor. I'm unsure of how to draw up an expression for v2(t) as I think it is strongly related to v1(t) but I'm not sure how to how to formulate an expression for it.enter image description here

\$\endgroup\$
4
  • 1
    \$\begingroup\$ Please show what difficulties you have, in other words, share all your equations and thoughts. This site is not a free homework service providing complete answers, but we're happy to help you on your way finding the answers. (You can use the edit link to update your question) \$\endgroup\$
    – Huisman
    Commented Feb 24, 2020 at 21:29
  • 1
    \$\begingroup\$ So I suppose you can assume when it says, "for a long time", it suggests that it's a fully charged capacitor. After "a long time", when you finally decide to flip the switches SW1 to open and SW2 to close, you essentially have this voltage source at C1 with a rapid exponential decay function. From here you should be able to use normal circuit analysis but instead of having a static DC voltage with a function of \$V(t)=10\$, it'll be something like \$V(t)=10e^{-kt/ \tau}\$... You should be able to write a differential equation for t > 0, or perhaps use the Laplace Transform. \$\endgroup\$
    – user103380
    Commented Feb 24, 2020 at 22:26
  • \$\begingroup\$ Why would your lecturer hand you a question where you can't even start on it? \$\endgroup\$
    – jonk
    Commented Feb 25, 2020 at 0:34
  • \$\begingroup\$ Clues: 1. Energy in a capacitor depends only on capacitance and voltage. 2. When you open SW1 and close SW2 C1 discharges and C2 discharges to some voltage. They will end with the same voltage on each and you will lose some energy in R2 but you can't destroy charge. If you need further help edit the question to show us what you have tried. \$\endgroup\$
    – Warren Hill
    Commented Feb 25, 2020 at 9:01

1 Answer 1

Reset to default
-1
\$\begingroup\$

At T<0 Energy stored in C1 is 500u Joules

Joules = ½ QV where V=10V Q=(C * 10V) so Q=10uF * 10V

At T1 and T2 simple use the decay function V(time) = Vss + (Vinit - Vss)e-t/TC where Vss is steady state (of 5 volts ...which is half of the orig 10 evenly divided between the caps) and Vinit is 10 volts

I suspect each cap (ideally) will retain 5 volts each, as each could/should provide the same equilibrium (being the same size)

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.