Why exactly does peaking of magnetization current occur in a transformer?

Question

Initially assuming that the input current is sinusoidal.

In a practical transformer, flux \$\phi\$ produced in the primary is not linearly proportional to the current \$I\$ through it. Due to saturation, the flux flattens

It's said that this flattening implies the presence of a dominant 3rd harmonic in addition to the fundamental

Questions:

1. Why does the presence of the third harmonic in the flux, alter the current (by adding a third harmonic out of phase by a 180\$^{\circ}\$)?

2. Why is it out of phase?

Answer 1

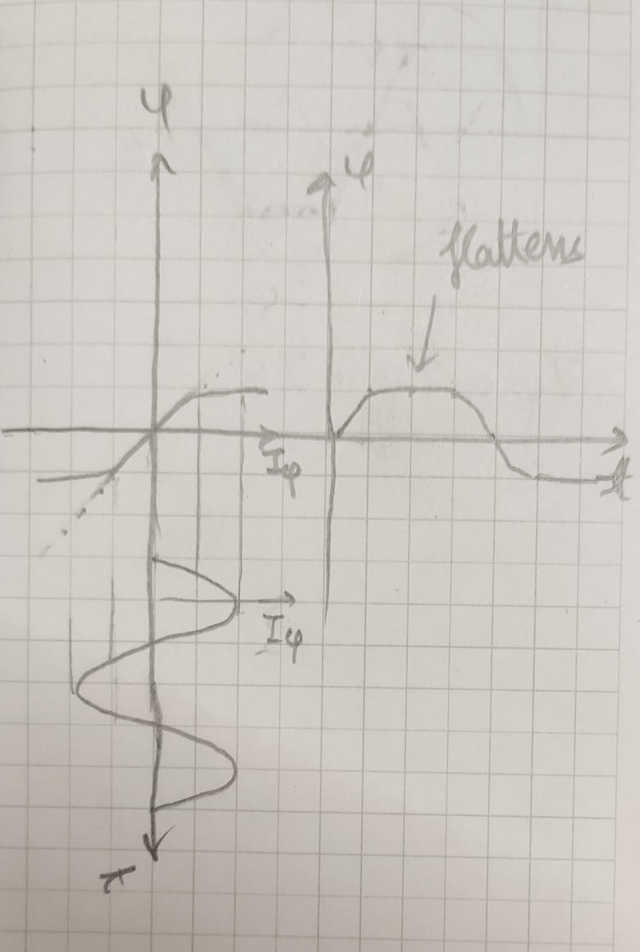
Initially assuming that the input current is sinusoidal.

and

Why does the presence of the third harmonic in the flux, alter the current (by adding a third harmonic out of phase by a 180∘)?

That's the bottom line - no matter what the flux does (in terms of non-linearity), the current is sinusoidal by definition.

However, if the winding were driven by a sine wave voltage then that's a different story and, current can appear very oddly affected by the diminishing flux at higher peak levels: -

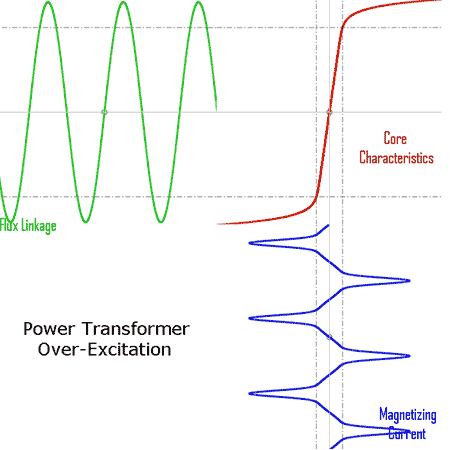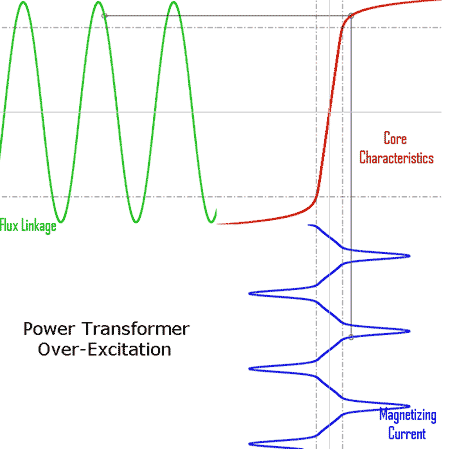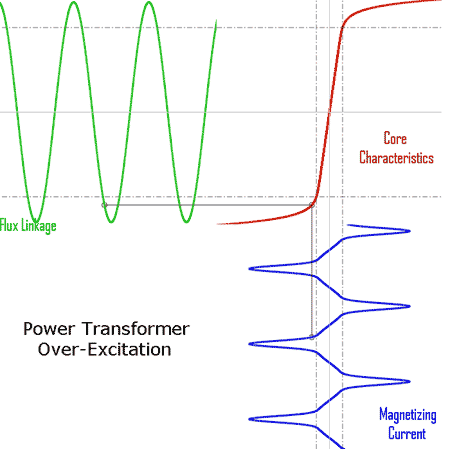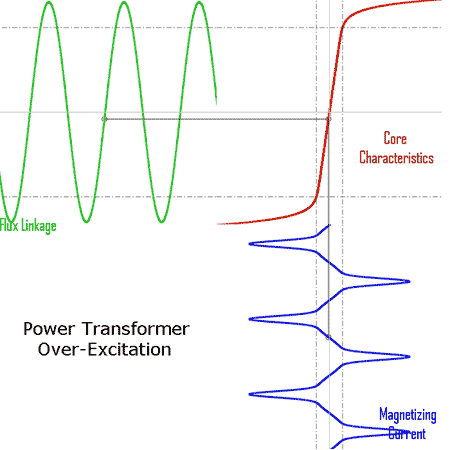
GIF from here.

---

Reason

In a normal inductor this formula applies \$V = L\dfrac{di}{dt}\$

This gives us the sine-cosine relationship between voltage and current but, only if inductance is unchanging. As the core saturates, inductance drops dramatically and this, in turn, leads to a much greater \$\frac{di}{dt}\$.

Then, knowing\$^1\$ that the definition of inductance per turn is \$\dfrac{\Phi}{I}\$, if \$L\$ decreases and \$I\$ increases, then flux remains unaffected.

---

\$^1\$ We are taught that \$V = L\dfrac{di}{dt}\$ and this is taken from Faraday's law: \$V = N\dfrac{d\Phi}{dt}\$.

So, if we equate them: \$\dfrac{L}{N} = \dfrac{d\Phi}{di}\$ or, inductance per turn equals flux per amp.