I am having troubles trying to translate when finding a transfer function of an active filter is it in a 'Close loop' or 'Open loop' state?
Of course, the filter function is available in a closed-loop condition only. It is the frequency-variable feedback network which gives the desired filter characteristic.
When finding the transfer function of these active op-amps how come we don't include the characteristics of the op amp itself?
It is common practice to assume idealized opamps only (infinite gain, no frequency dependent gain). Of course, this simplification causes errors in the transfer characteristic - however, this is acceptable as long as the operating frequency range is limited to a region where other uncertainties (parts tolerances) are dominating. Of course, this means that opamp based filters are used not in the upper MHz range (where opamp non-idealities play a remarkable role).
Please note, that there are many, many different lowpass circuit alternatives which ALL would have the same transfer function in practice (reality) - under the assumption of IDEAL opamps. The difference between these alternatives can be revealed only for REAL opamps. In this context, it is important if the opamp is used as a fixed-gain positive amplifier (Sallen-Key) or as a high-gain amplifier (multi-feedback) or as an integrator (state-variable structures). All these alternatives have different sensitivities against opamp non-idealities.
For example a Op-Amp has an Op-loop gain I would imagine you need to include that in your transfer function otherwise you could use any op amp/disregard any op amp?
Of course, theoretically we could include the open-loop gain of the amplifier into the overall transfer function....but for which purpose? To slightly improve the accuracy of the function ? The price for it would be a very complicated transfer function which is very hard to use for practical realisations.
Remark: There are studies which show how the frequency-dependent open-loop gain of an opamp can be exploited - together with a pure resistive feedback network without external capacitors - for realizing active filters ("R-filter). However, as a severe drawback, it is necessary to know the exact open-loop gain opamp characteristic, which has very large tolerances. Buth these filters have no practical relevance....
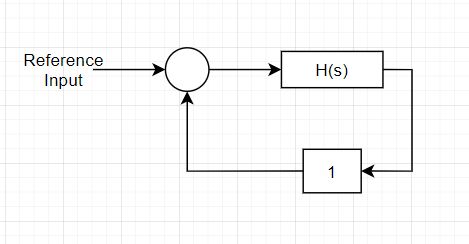
Comment 1: Of course, it is possible to create a block diagram in the classical form with a feedback block (and with a summing junction at the input) and a block with the opamps open-loop gain - but for which purpose?
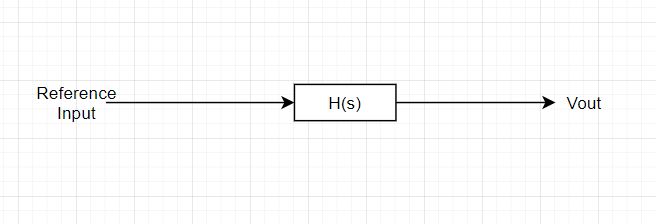
Comment 2: Actually, as an advantage of block diagram visualization, it is very easy to see HOW the S&K lowpass works: There is a passive lowpass (Hforward) with a bad quality factor (pole-Q) Qp<0.5.
However, in the "critical" frequency region (exactly at the pole frequency) the bandpass in the feedback path enhances the amplitude of the lowpass because the bandpass has the same pole frequency (zero phase shift, positive feedback effect). This effect produces a larger Q value (Example: Qp=0.7071 for a Butterworth response).
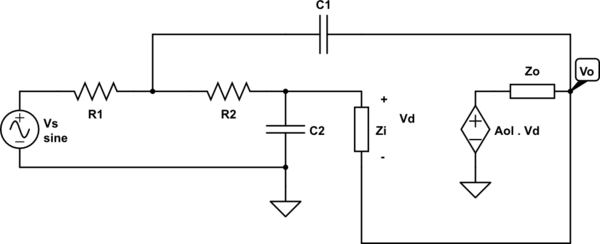
EDIT: Block diagram: Both passive transfer functions (lowpass, bandpasss) are derived from the original circuit. Vp is the signal voltage at the positive (non-inverting) opamp input terminal.

simulate this circuit – Schematic created using CircuitLab

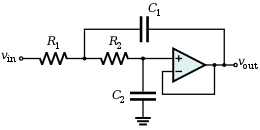

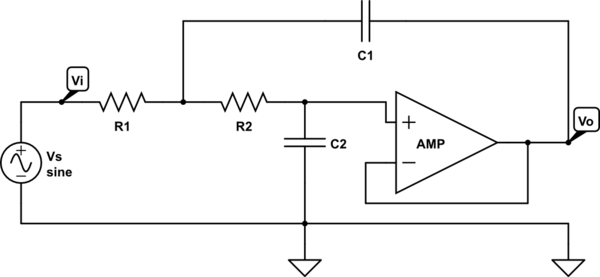
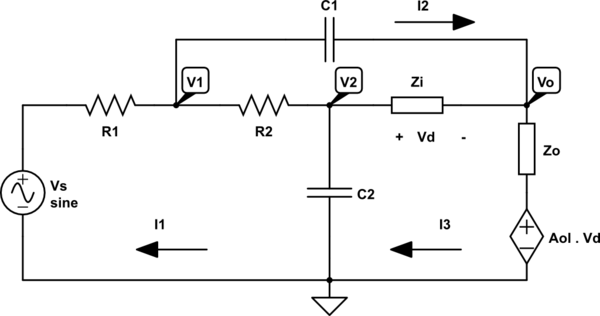
V(out)/V(in), that is, you multiply the denominator of the transfer function withV(out), and the numerator withV(in). \$\endgroup\$