Why doesn't light destroy the PN junction voltage gradient in a solar cell?

Question

I understand that a solar cell is, essentially, a P-N junction. A P-N junction, roughly speaking, will do the following: The free electrons on the N-side will flow over to fill holes on the P-side. This produces a voltage gradient where the P-side is slightly negative and the N-side is slightly positive.

Now, when light strikes the P-N junction, it excites free electrons all over the place. These electrons, if they are close to the junction, are drawn towards the positively charged N-side. This creates current and this is how a solar cell produces power.

The way it has been described to me is that, at first, the N-side is slightly positive and the P-side is slightly negative (it's acting like a normal diode). But after light hits, this reverses. The N-side becomes very negative and the P-side very positive. By attaching a conduit between these sides, you can draw power from that current.

Here is my question

After light strikes the P-N junction, and all those electrons are drawn to the positively charged N-side....wouldn't the N-side quickly lose it's positive charge and return to being neutrally charged? This would destroy the drift voltage... so any free electrons would have no reason to build up on the N side and there wouldn't be any voltage.

What am I missing here?

Answer 1

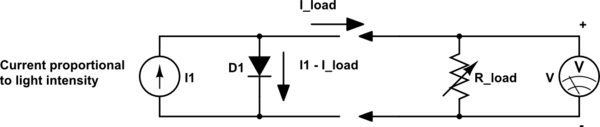
Light DOES destroy the static (or built-in) potential of the PV cell. That's how PV cells work! (At least, at the macro level, that's how the internal voltage-drops behave.)

During darkness, we might find 1.0V static potential between the n-type and p-type parts of the solar cell. This potential is really there, it's the energy-hill appearing in the depletion zone. But it can only be detected by non-contact electrometers (where no metal probes make contact, so no unwanted metal/silicon junctions are formed.)

Besides the junction-potential, we'd also find another potential where the metal terminal connects to the n-type, and a third where the other terminal connects to the p-type. These two "ohmic" or "non-rectifying" built-in potentials are much like static potential of any thermocouple ...though quite a bit higher volts. Roughly 0.5V each. They sum to the same value as the PN junction potential. That way, if the solar-cell contacts are shorted, there will be zero current, even though in darkness the junction exhibits an energy-hill of one entire volt!

And so, when light strikes the junction, carriers flood the depletion zone, and the staticf junction-potential is "shorted out." But this doesn't affect the two 0.5V steps located where metal contacts touch silicon.

As a result, the potential measured across the terminals has an upper limit: roughly 1.0V. PV output-voltage doesn't rise continuously as light-intensity rises. That one-volt limit was the same value as static built-in potential of the PN junction during darkness. That potential is now missing, so it will now appear at the output terminals of the PV cell.

Very strange, eh? Solar cells are actually driven by the sum of the two potential-steps found at their ohmic contacts. It almost seems like perpetual motion! But the electromotive force at the metal junctions can only pump some charges when photons are injecting energy into the PN junction, and therefore "promoting" valence electrons into the conduction band, without them having to be pushed there by an external power supply. The depletion zone is shorted out by the sudden new population of carriers there. The diode becomes forward-biased. Yet the energy needed to do this ...is exactly the energy being injected by the ohmic contacts, as carriers "fall down" the contact-potentials found at the metal-semiconductor bonds.

"Contact potentials" are weird stuff!

And now you can get an idea about Peltier module function. A thermoelectric module works because p-type and n-type blocks are welded onto little copper straps, with no diodes being formed. Yet the potential-hills are really there. They all sum to zero around the circuit ...unless half of the metal-semiconductor contacts are heated up, and the other half cooled down. As with solar cells, the heated contacts become "shorted out" because of injected energy at the micro-level: phonon absorption, which knocks the carriers uphill over the built-in junction potentials. Heh, a Peltier thermogenerator is a bunch of PHONO-voltaic cells in series! (As in: lattice thermal energy, junctions "illuminated" by phonons not photons.)

Confusing regarding measuring barrier potential of a pn junction using a voltmeter

Answer 2

When light strikes the silicon of a solar cell, it produces electron-hole pairs "all over the place", as you put it. But the only ones that matter are the ones that are created within the depletion region of the PN junction. They are the ones that get separated by the E field within the junction to produce the terminal voltage that you can measure (\$V_{oc}\$). The rest just recombine on their own almost right away.

As you say, this is effectively a current source that is in parallel with the diode junction:

^{simulate this circuit – Schematic created using CircuitLab}

The point is, this current flows all the time. If you don't draw it off through an external circuit, it simply flows through the diode junction itself.

There is no reversal of voltage across the junction. The normal zero-current voltage is merely reduced by the photocurrent until a new equilibrium is reached. It is this difference between the quiescent voltage and the reduced voltage that we can read using an external voltmeter, and the anode is positive with respect to the cathode.