How to solve the above question?
I cannot apply Kirchoff's current equation because of the presence of the dependent voltage source
Also, I am not able to apply supernode because of the 2 kOhm resistor in series. How should I go about it? Please help.
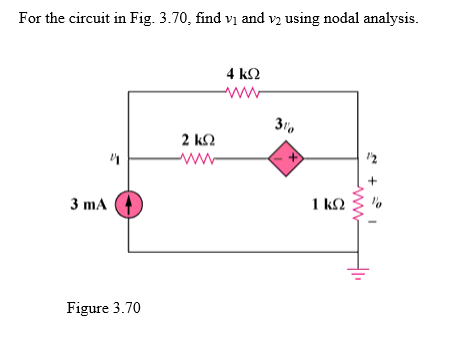
Well, we are trying to analyze the following circuit:
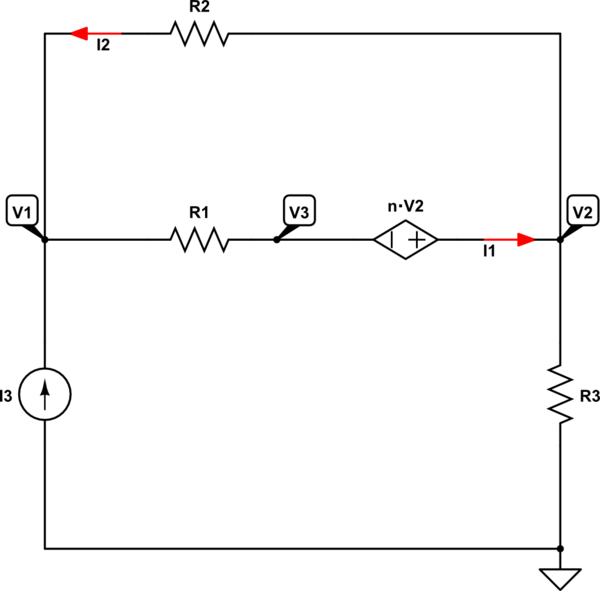
simulate this circuit – Schematic created using CircuitLab
When we use and apply KCL, we can write the following set of equations:
$$\text{I}_1=\text{I}_2+\text{I}_3\tag1$$
When we use and apply Ohm's law, we can write the following set of equations:
$$ \begin{cases} \text{I}_1=\frac{\text{V}_1-\text{V}_3}{\text{R}_1}\\ \\ \text{I}_2=\frac{\text{V}_2-\text{V}_1}{\text{R}_2}\\ \\ \text{I}_3=\frac{\text{V}_2}{\text{R}_3} \end{cases}\tag2 $$
We also see that \$\text{V}_2-\text{V}_3=\text{n}\cdot\text{V}_2\$.
Now, using those equations your problem can be solved.
Everything is in series with the current source, meaning the voltage across the 1K resistor must be 1000*0.003, from there you solve the rest of the circuit
The step after this, as you know the V2 node would be how that current source is split between the 2K + voltage source and the 4K Resistor, if you need to, you can imagine it as 2 voltage sources with an ESR, both in parallel with each other.
