2
\$\begingroup\$

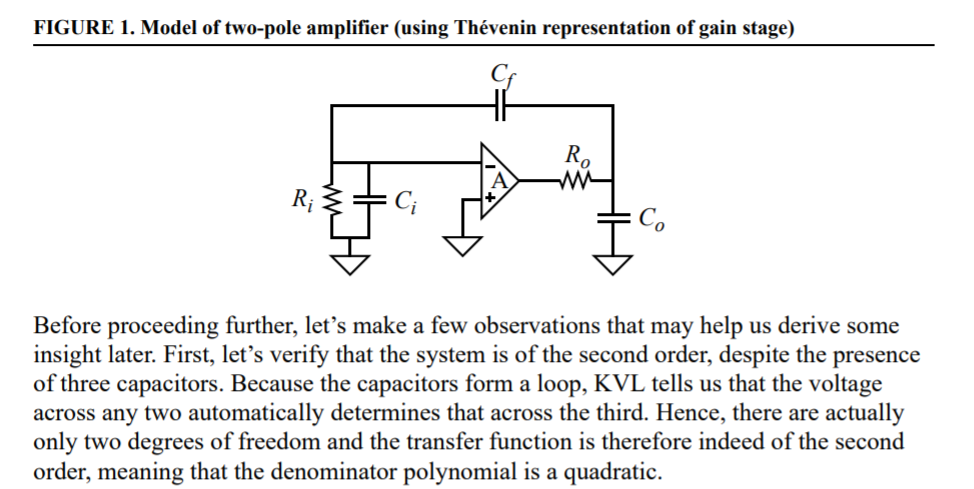
I encounter some engineers who can quickly spot poles and zeros in the circuit by identifying "degree of freedom". For example, a typical technical discussion goes like : "a miller compensation has two degree of freedom, therefore two poles, a feedforward path gives it a zero. A nulling resistor gives it additional degree of freedom, inevitably adds another pole." This kind of technical discussion sounds incomprehensible to me. (The only methods I was taught are nodal analysis, some open/short circuit time constant techniques, and some phasor estimations on load) .

It seems Prof. Thomas Lee started this: -

enter image description here

I wonder are there anyone here know the insight behind those "degree of freedom" estimation ? What are the rationale mathematically?

\$\endgroup\$
7
  • \$\begingroup\$ Have you looked at the circuit in question and recognized what is being said? I've edited your question to make it clearer. Please roll-back if it doesn't meet your requirements. I can roll it back if you are dissatisfied. \$\endgroup\$
    – Andy aka
    Commented Jun 22, 2020 at 9:50
  • \$\begingroup\$ @Andy aka Yes. Thanks for your edit and it looks great. I just cannot understand what "degree of freedoms" have to do with poles. What are the maths behind that? So we can choose two voltages freely by setting three caps, but how does that give us two poles..? \$\endgroup\$
    – Eagle Shou
    Commented Jun 22, 2020 at 9:56
  • \$\begingroup\$ Slow down Mr Eagle! I'm just fixing up your question so that it makes it easier to grasp. Someone, will answer this I'm sure. Personally I don't look at it in terms of degrees of freedom because it doesn't gel with me. I look at the circuit and see that there are two poles and one zero but, if I got that analysis incorrect, it doesn't matter because I can always do the math on it and derive a TF. However, the circuit above is a poor example because, although it is called an amplifier, there are no clear inputs or output. Maybe look for something more clear-cut. \$\endgroup\$
    – Andy aka
    Commented Jun 22, 2020 at 10:00
  • 2
    \$\begingroup\$ I would recommend that you take a look at a seminar that I taught in 2016 at APEC which shows the relationship between the natural time constants of a circuit (passive or active) and the poles of this circuit. Studying a circuit by looking at its time constants - with or without stimulus - lays the foundations for the fast analytical circuits techniques or FACTs. Indeed, when skilled in the art, you can infer how many poles and zeroes are in the transfer function just by looking at the schematic. \$\endgroup\$
    – Verbal Kint
    Commented Jun 22, 2020 at 10:47
  • 2
    \$\begingroup\$ It seems to me that the author of the paper you mentioned calls the degree of freedom the ability of writing state variables equations independent from each others. If you have 3 capacitors, you have three state variables. If none of these equations exclusively depends on the other two, then you have a 3rd-order system. If one of the state variable is a linear combination of the other two, then you lose a degree in the denominator and end up with a second-order system. \$\endgroup\$
    – Verbal Kint
    Commented Jun 22, 2020 at 10:54

1 Answer 1

Reset to default
-1
\$\begingroup\$

As my understanding of system dynamics and control systems, poles are system's internal vibration mode. Imagine a system with two degree of freedom, then they have a 2D vibe modal, each have a own frequency. And the combination builds up the total system's output. Similarly, this circuit system have two energy storage components, the RC oscillator, so this circuit is a 2 degree freedom system. And the feedforward path could be think as the combination of the system's vibration modal. Such as, two combined vibration mode add up can lead to zero output. So, I assume the feedforward is the possibility of the combinations of the poles related vibrations thou. Hope this make some sense and helpful.

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.