Confusion Between Dual Capacitor/Inductor Circuits

Question

I'm trying to physically understand the responses of this pair of (dual) circuits: a step current source driving a capacitor and a step voltage source driving an inductor.

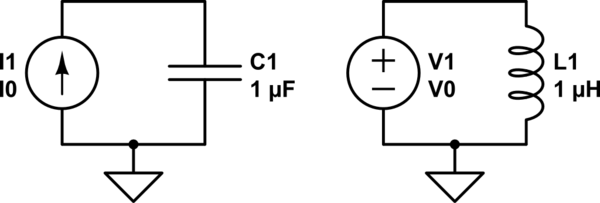
^{simulate this circuit – Schematic created using CircuitLab}

The capacitor output voltage is a ramp and the inductor output current is a ramp, as expected. I understand fine how to mathematically derive these responses, what I'm trying to do is physically explain them.

What I have for the capacitor is that if we assume zero initial charge on the capacitor, then its initial voltage is zero because there is no charge difference. At time t = 0 the current source steps to some \$I_0\$. This means that, by definition of current, the source is now transferring charge onto the plates of the capacitor such that a charge separation develops on the plates over time. Assuming an LTI capacitor such that \$ q = CV \$, the voltage ramps up since the charge difference on its plates is being increased at a constant rate.

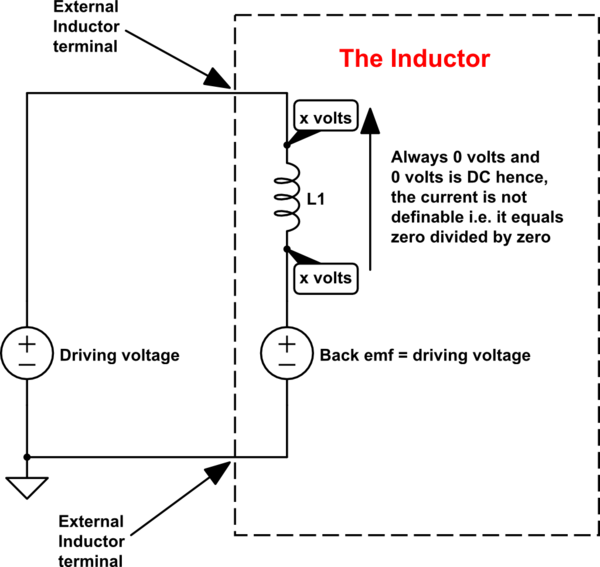
I can't quite articulate an equivalent description of the inductor circuit. I have a basic understanding of the phyiscal origin/operation of an inductor but I'm struggling to put it together here. My understanding is that, assuming an LTI inductor, it is defined by the relationship \$ \phi = Li \$, where \$ \phi \$ is the total flux linked through in the inductor. I also know that by Faraday's law, \$ v(t) = -\frac{d\phi}{dt} \$, so we can differentiate the inductor relation to get \$ v(t) = L \frac{di(t)}{dt} \$ (dropped the minus sign). From here I can mathematically derive current as a function of voltage, but why is this constant (step) voltage source apparently causing a constant change in magnetic flux that causes the finite change in current?

When I think of a change in magnetic flux through a current loop that induces an EMF and a current, I think of something like this: 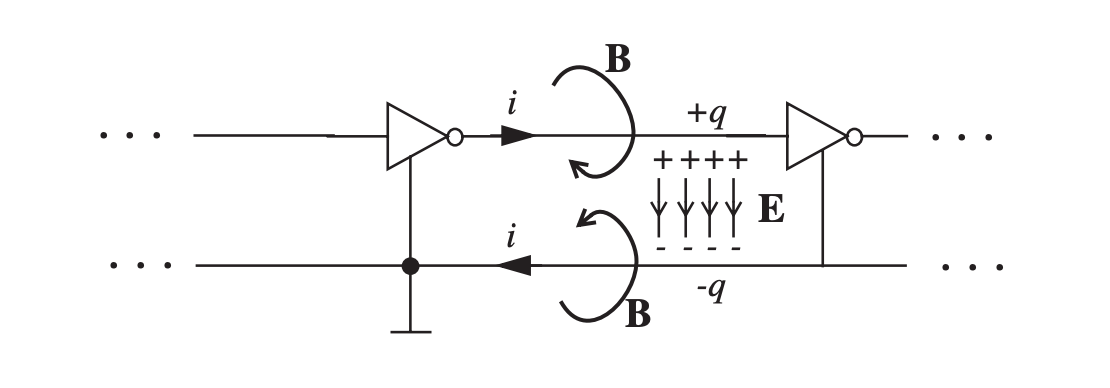I don't see how the voltage source creating a constant voltage across the inductor's terminals does this.

Answer 1

but why is this constant (step) voltage source apparently causing a constant change in magnetic flux that causes the finite change in current?

You seem to be changing the way you express things when you say this so I'm going to clear this up first. You appear to be equating: -

$$\boxed{\text{a constant change in magnetic flux WITH a finite change in current}}$$

In truth, any change in flux is also a change in current. The two are inseparable; one follows the other. I just wanted to clear that up. You probably meant what I re-phrased anyway!

From a comment: -

So does the emf approximately all drop across the inductor because the inductor is what experiences most of the mag field change?

That is correct and what confuses some people is that if the back emf exactly matches the forward applied voltage, then how come there can be any current. Maybe that's what is confusing you. If that is the case, it can be answered but needs a little thought. Basically it boils down to this: -

$$\boxed{\text{back-emf tells us nothing about the current that flows in an inductor}}$$

One more thing, you say this: -

My understanding is that, assuming an LTI inductor, it is defined by the relationship ϕ=Li

This is not quite true. This is correct: -

$$\Phi = \dfrac{L}{N}\cdot i$$

In other words, inductance per turn = \$\Phi\cdot i\$.

Hope this helps.