Proving the formula for parallel resistors

Question

I am currently studying The Art of Electronics, third edition, by Horowitz and Hill. Exercise 1.3 asks to prove the formulas for series and parallel resistors. I am given the following relevant information earlier in the chapter:

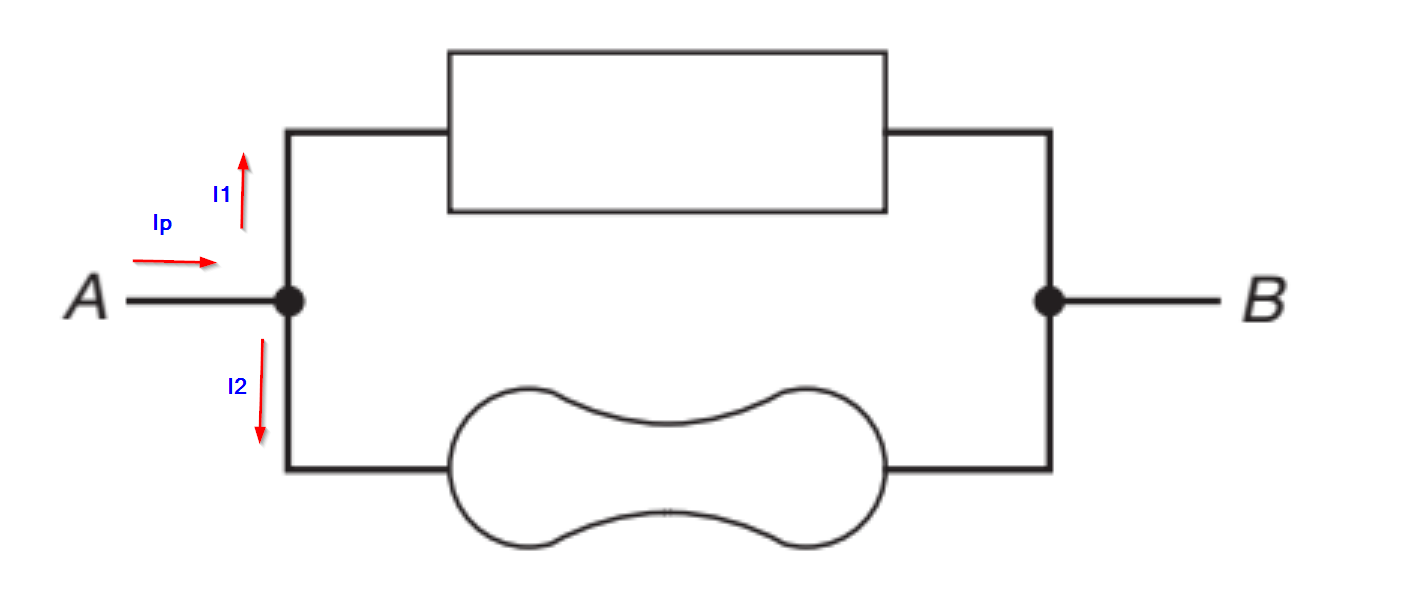
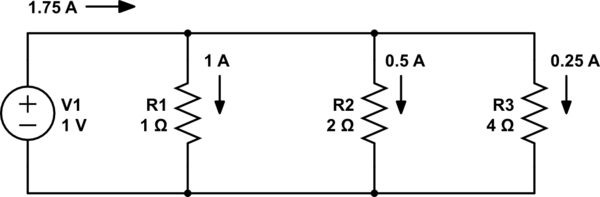
1. The sum of the currents into a point in a circuit equals the sum of the currents out (conservation of charge). This is sometimes called Kirchhoff’s current law (KCL). Engineers like to refer to such a point as a node. It follows that, for a series circuit (a bunch of two-terminal things all connected end-to-end), the current is the same everywhere.
   
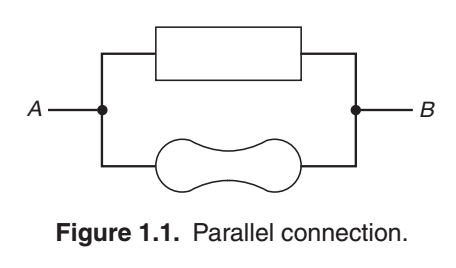
2. Things hooked in parallel (Figure 1.1) have the same voltage across them. Restated, the sum of the “voltage drops” from A to B via one path through a circuit equals the sum by any other route, and is simply the voltage between A and B. Another way to say it is that the sum of the voltage drops around any closed circuit is zero. This is Kirchhoff’s voltage law (KVL).

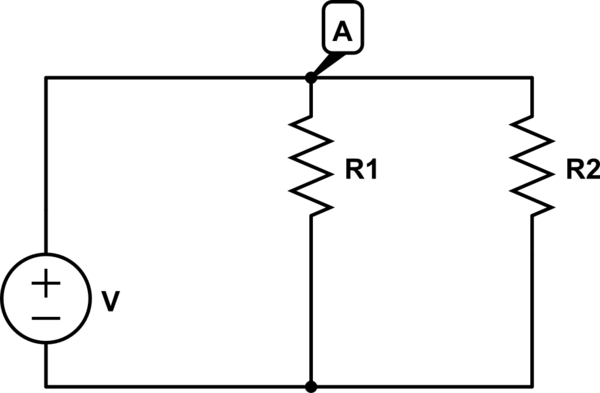
I am trying to use this information (and ohm's law \$ V = IR \$, of course) to prove the parallel case \$R = \dfrac{R_1 R_2}{R_1 + R_2} \$. However, I do not understand how the provided information is sufficient to deduce this.

I am told that this is a good textbook, so perhaps it is just that I am new to electronics, and so I am not understanding something. Or, perhaps it is the case that the authors have failed to provide enough information to solve this problem without external knowledge. I would greatly appreciate it if people would please take the time to explain how it is possible to deduce this with the given information.

Answer 1

AoE is not a particularly theoretically inclined book, it's more of a practical guide with the minimum amount of mathematics that is required to do the analysis. Whether that is "good" or not depends on your goals in learning electronics.

In any case, if we want to replace two resistors with one equivalent, we can calculate the equivalent resistor from the current that would flow. It's given that the voltage across each resistor is the same.

For R1 current is I1 = V/R1, With R2 current is I2 = V/R2, so the total current is:

I1 + I2 = V(1/R1+1/R2) and our "equivalent" resistor Rp = 1/(1/R1+1/R2) = R1R2/(R1+R2).

Adding the currents into the node: Ip - I1 - I2 = 0 (KCL) so Ip = I1 + I2

Answer 2

It might be easier to understand how it works if you use the inverse of resistance - conductance.

In a parallel circuit each component has the same voltage across it, so the current flowing through it is independent of the others. Total current is the sum of the individual currents, so the total conductance is simply the sum of the individual conductances.

Take the example below:-

^{simulate this circuit – Schematic created using CircuitLab}

R1 has a conductance of 1 / 1 Ω = 1 A/V, R2 is 1 / 2 Ω = 0.5 A/V, and R3 is 1 / 4Ω = 0.25 A/V. Add them all together to get a total conductance of 1.75 A/V, then invert the result to get the total resistance of 1 / 1.75 = 0.571Ω.

This gives the general formula for parallel resistances:-

\$\frac{1}{Rt} = \frac{1}{R1} + \frac{1}{R2} + ...\frac{1}{Rn}\$

In the special case of only two resistors in parallel you can rearrange the formula from

\$\frac{1}{Rt} = \frac{1}{R1} + \frac{1}{R2}\$

to

\$Rt = \frac{R_1 R_2}{R_1 + R_2}\$

Answer 3

^{simulate this circuit – Schematic created using CircuitLab}

The total current in the circuit will be It = V/Rt

According to KCL It = I1+I2 where I1 is the current flowing through the R1 resistor and I2 is the current flowing through the R2 resistor.

If we substitute(Ohm's law):

V/Rt = V1/R1+V2/R2. But since the resistors are in parallel V1 = V2.

But since there isn't a resistor between the the voltage source and the resistors V = V1 = V2.

V/Rt = V1/R1+V2/R2 -> V/Rt = V/R1+V/R2 and if we divide by V we will get 1/Rt = 1/R1 + 1/R2.