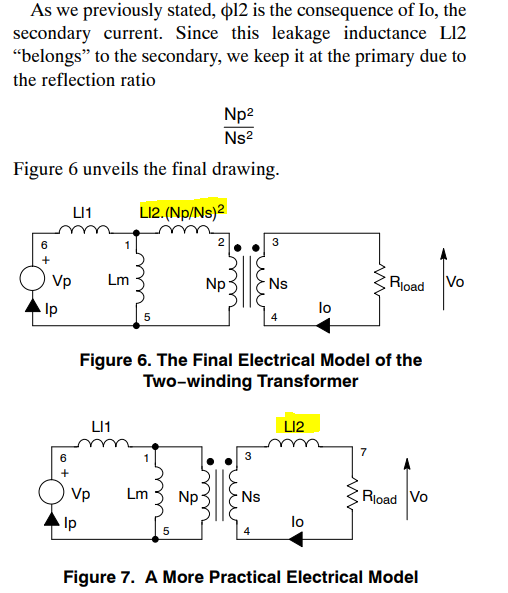
I found the following transformer model in the AN1679/D written by ON SEMICONDUCTOR. The document is really interesting and very well explained.
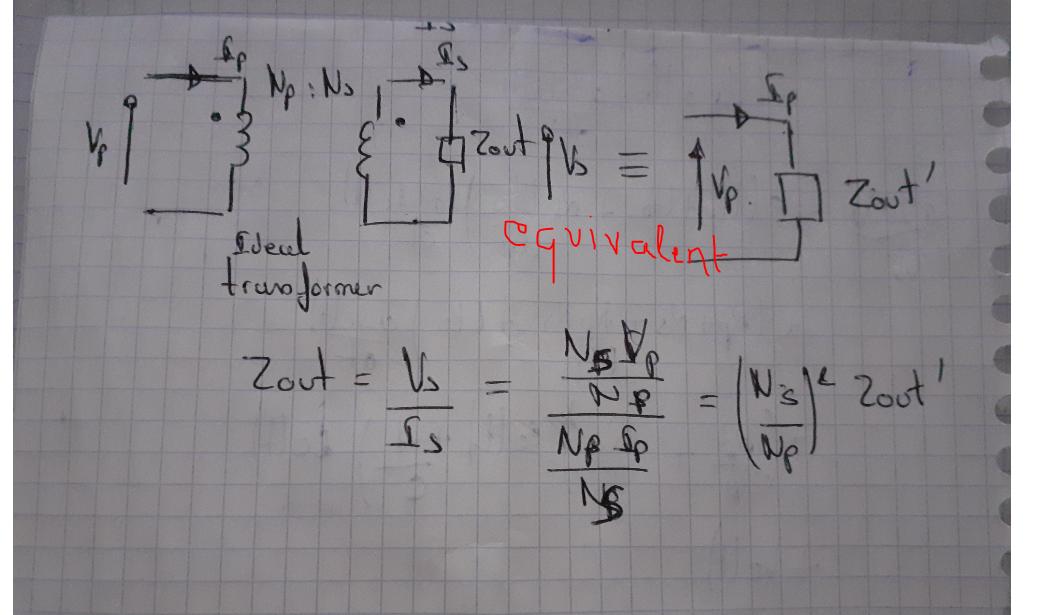
Nevertheless, I do not understand this point, how the two model are equivalent :
If I try to put Ll2 which is at the secondary to the primary, I do not find the relation given :( Here are my calculs :
$$ \mathrm{ L_{l2}*\frac{dI_{0}}{dt} = \frac{N_{p}}{N_{s}}(V_{p}-V_{L1}) -V_{0} } $$
and
$$ \mathrm{ L_{l2'}*\frac{N_{s}*dI_{0}}{N_{p}*dt} = V_{p}-V_{L1} -V_{0} } $$
We then express each relation equal to
$$ \mathrm{ \frac{dI_{0}}{dt} = \frac{dI_{0}}{dt} } $$
For express Ll2 in function of Ll2', and we get the correct relation only if Ns = 1 and Np = 1...
Did I do an error ? I m really interested by this subject !
Thank you very much and have a nice day !