How fast is DC current/voltage?

Question

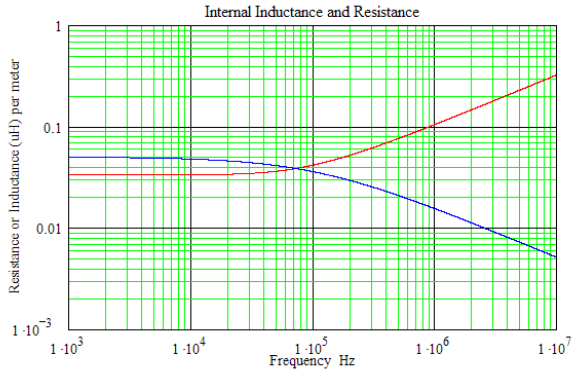
The standard way to calculate the speed of information (current/voltage, EM wave) through a dielectric medium (like PTFE or fiber) is to determine the relative dielectric constant, and compute the velocity factor.

You could use this information, theoretically, to calculate the delay between a signal sent over CAT-7 from New York to London.

However, these properties are microwave/high-frequency properties. How is the propagation speed of information calculated for low-frequency EM waves? How long does it take my DC source to send information around my circuit? How long does it take a battery to turn on my device?

OP Edit When I asked this question, I wasn't careful to put my question into words. The real question is about what the frequency component at 0 Hertz even means. How long do I have to wait to measure it? What does it mean to use a DC meter to measure voltage and current? Why is DC special relative to all other components of a waveform. I ended up providing my own answer because I realize that the answers to my question (rightly) understood me to be asking a different question.

Answer 1

First, as stated, DC carries no information. Switching DC on or off creates a step function, i.e. a waveform with harmonics that may go into the RF. A "perfect" (theoretical) step waveform would have harmonics through radio frequencies (RF), infrared radiation (IR), visible light and all the way to gamma rays... but since decreasing the wavelength increases energy of the minimal photon, that is actually not possible. However, RF harmonics can be heard as a click on a nearby AM radio when opening or closing a DC circuit.

So your question should be how fast a specific frequency propagates in a specific medium, the velocity factor. For example, in RG-8/U cable, a common RF coaxial cable, the signal propagates about 3/4 the speed of light in vacuo. Or for light, the refractive index, n, expresses the ratio to speed in a medium vs. vacuum. In germanium, IR crawls at 1/4 the speed it would have in vacuo.

Answer 2

How is the propagation speed of information calculated for low-frequency EM waves?

Same as for high frequencies, except the inductance is higher due to reduced skin effect. Inductance reduces the rate of current change in the wire, so higher inductance makes the signal travel slower.

For example the graph below (from here) shows resistance (red) and internal inductance (blue) of RG-58 coax from 1 kHz to 10 MHz. Below ~20 kHz the inductance is practically the same as its 'DC' value, so the propagation velocity in this region is constant. At higher frequencies (where skin effect kicks in) the inductance reduces, which makes higher frequency components of the signal propagate faster.

Here is the measured delay time of an 81 foot length of RG-58 cable from 10 kHz to 1 MHz, indicating ~10% increase in propagation velocity at 1 MHz.

How long does it take my DC source to send information around my circuit? How long does it take a battery to turn on my device?

That depends on the transmission line characteristics of the wiring. If your device was powered through the 81 foot length of RG-58 cable above, it would take ~134 ns for the 'DC' voltage to get to the device.

Answer 3

I'm electing to provide my own answer, since none of the other answers satisfies me completely. The following is a summary of this discussion from r/AskPhysics.

Reviewing the other answers, first:

1. @DrMoishe Pippik's answer fails to address the original question which could be posed as "how is DC manifested in real life if DC can't be expressed as a non-zero sum of sinusoids" or "how is DC current/voltage measured if DC can't 'travel' around a circuit because it's not a wave"?
2. @Bruce Abbott's answer fails in a similar way, to couple the reported measurements of DC values to the propagation of waves down a transmission line.
3. @Aditya Rajendran's answer is not right.

My original question (which I'm sure could be phrased in a clearer way) is how DC values can be measured if DC is not a wave which propagates around a circuit. I.e. How does a DC voltmeter/ammeter work? The problem is with terminology. A DC voltmeter/ammeter doesn't measure the DC component of a signal. It just measures something close. Measuring the DC component of a signal would correspond to waiting literally forever in order to ensure the value will never change again. This isn't done in practice.

However, averaging the measured voltage over 1 second, for example, provides an approximation of DC using all frequency components which change value appreciable over that time interval (say of frequencies with periods of 10 seconds or lower). Waiting for more time does lower the minimum frequency (maximum period) that one is using for this average, but it increases the measurement time.

DC voltmeters try to strike a balance between waiting beyond the time of the heat death of the universe to report the actual "DC voltage" and not waiting for long enough time to capture low-frequency components.