Kirchoffs Laws and Class-A Amplifier Circuit

Question

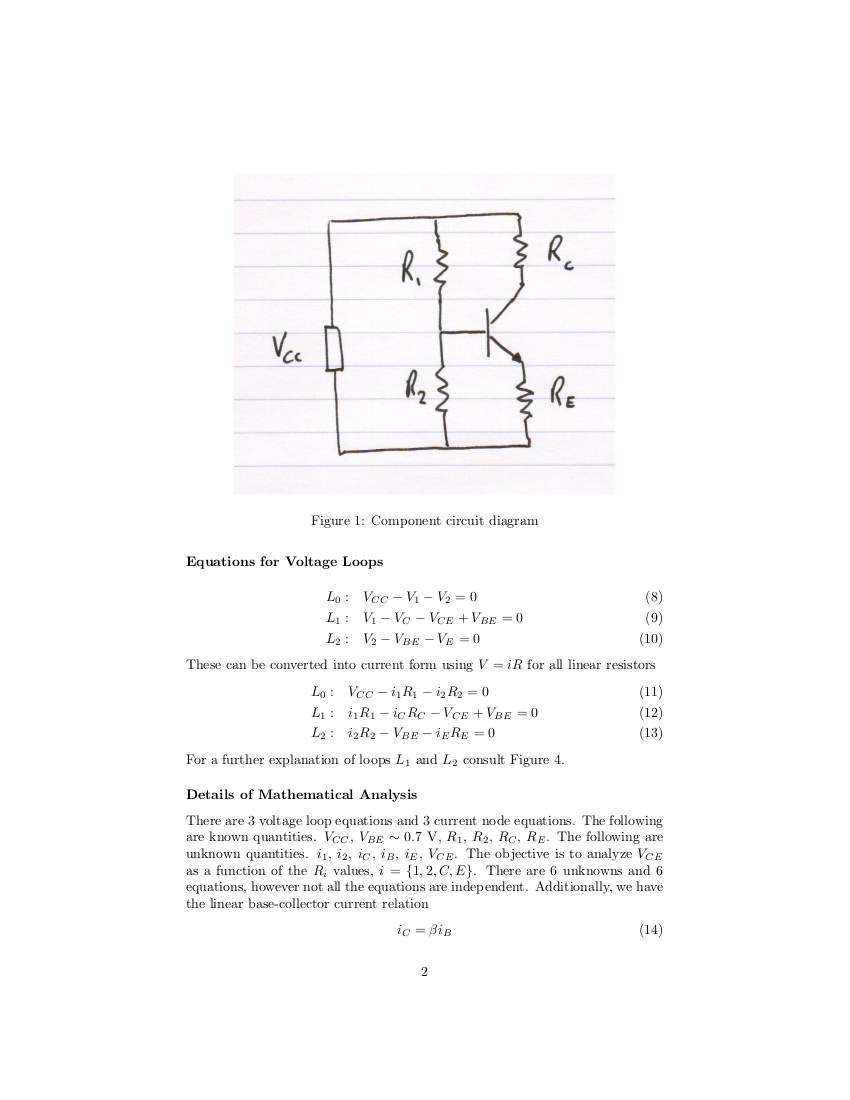
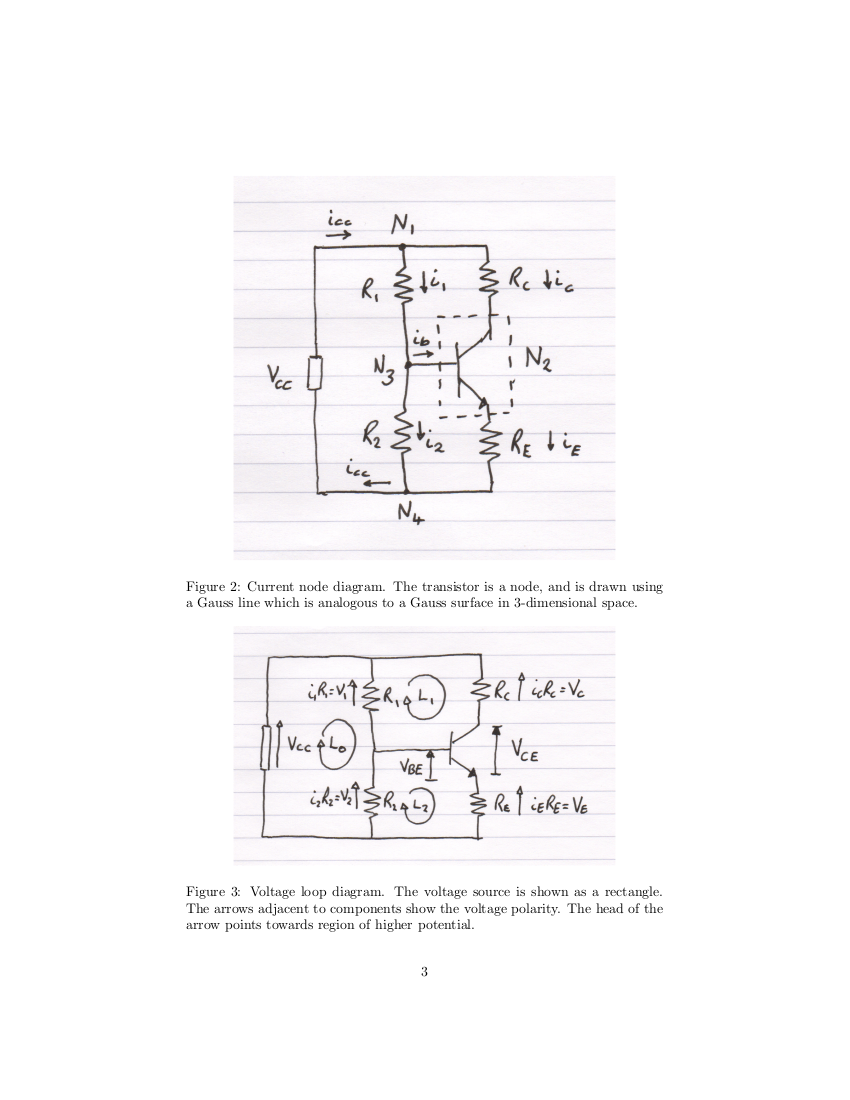
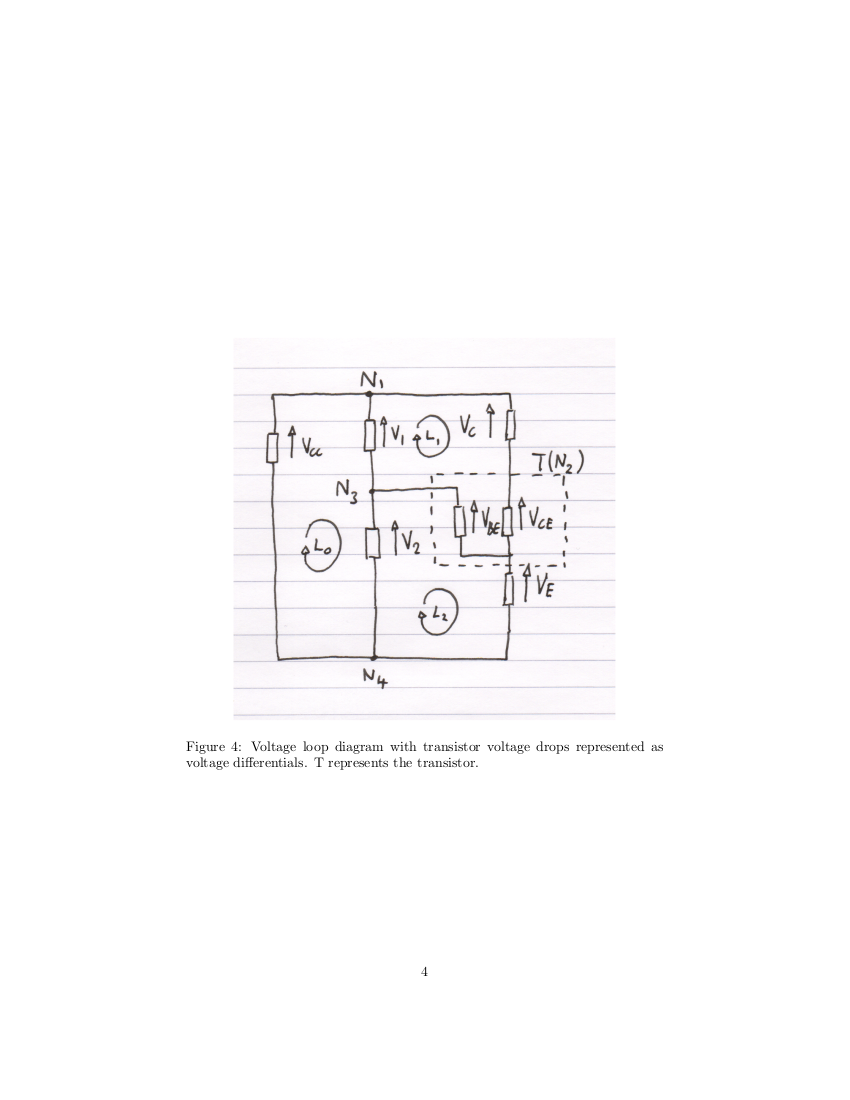
I have been attemtping to use Kirchoffs laws to solve a Class A amplifier circuit containing a single transistor.

I made several attempts at this trying slightly different approaches. I summarized the last attempt I made in a document, with some diagrams, which I will append to this question.

I got to a point where I had all the quantities I wanted in a single equation, with one additional quantity which I did not want (an unknown). This was i_B.

I ended up with this

$$V_{CC}=V_{CE}+\left(-(1+\beta)R_E-\beta R_C\right)i_B$$

I couldn't figure out how to proceed from here. Unless I made a mistake or otherwise missed something, I have used all the possible node/loop equations, some of which I found were not independent.

Possibly I missed a loop equation which is independent of the others or made some other error.

It must surely be possible to solve this system using Kirchoffs laws (plus one additional equation for the base-current to collector-current equation, aka transistor hfe).

Since writing this document I realized that there are a total of 7 possible loops with this circuit, 4 of which can be created from superpositions of the 3 minor loops. The minor loops are created by drawing "the most simple possible square loops on Figure 1/2/3".

I realized I could choose any path through the system to create a voltage loop equation from the parts of the system I am interested in.

Therefore I can do

$$V_{CC}=i_CR_C+V_{CE}-V_{BE}+i_2R_2$$

which picks up all the things I want: V_CC, V_CE, V_BE, but picks up additonal terms i_2 and i_C which I then don't have any equations which I can use to substitute for these terms without bringing in additional unwanted terms.

For example, Node 4 could be used to substitute for i_2, but this brings in i_CC and i_E. If I try to substitute for those things seem to just go in circles without progress.

Answer 1

The mistake is in the negative sign of the equation you draw $$V_{CC}=V_{CE}+\left(-(1+\beta)R_E-\beta R_C\right)i_B$$

If you draw the circuit and plot the currents you get the following diagram

^{simulate this circuit – Schematic created using CircuitLab}

Then, simply follow the Votage drop, you have Vcc that drops with the flow of the currents throughout the resistors and the jump between collector and emitter as follows:

$$V_{CC}=I_{c}R_{C} + V_{CE} + I_{e}R_{e}$$

You also know that

$$I_{c} = \beta I_{b} $$ $$I_{e} = I_{c} + I_{b} $$

If you replace the currents you get

$$V_{CC}=\beta I_{b}R_{C} + V_{CE} +( \beta I_{b} + I_{b} )R_{e}$$

Making it a bit more readable

$$V_{CC} = V_{CE} + I_{b}( \beta R_{c} + (1+ \beta )R_{e}) $$