How do I get a KCL equation involving Vin in this circuit?
KCL equations involve currents, not voltages, that said this might be the answer you are looking for..
In the case of your circuit there is only one KCL equation which can be made to involve \$ Vin \$, namely the sum of the currents into the node between \$ R1 \$, \$ Rin \$ and the current source: \$ I_{R1} + I_{Rin} + gm V_{be} = 0\$ (1)
now from ohm's-law; \$ I_{R1} = \frac{V_{R1}}{R1} \$ (2) and \$ I_{Rin} = \frac{V_{Rin}}{R_{in}} \$ (3)
substituting (2) and (3) into (1); \$ \frac{V_{R1}}{R_{1}} + \frac{V_{Rin}}{R_{in}} + gm V_{be} = 0 \$ (4)
to get \$ V_{in} \$ involved we can apply KVL to the mesh involving the voltages across the two resisors; \$ V_{in} - V_{Rin} + V_{R1} - V_{be} = 0 \Leftrightarrow V_{in} + V_{R1} - V_{be} = V_{Rin} \$ (5)
substituting (5) into (4); \$ \frac{V_{R1}}{R_{1}} + \frac{V_{in} + V_{R1} - V_{be}}{R_{in}} + gm V_{be} = 0 \$ (6)
And now you have a KCL equation (6) involving \$ V_{in} \$
Here the reference directions are taken such that all currents into the node are possitive, and the voltage reference directions follow from the standard conventions.
EDIT; The OP made a mistake in the schematic,
after the correction equation (1) has to be; \$ I_{C1} + I_{R1} + I_{Rin} + gm V_{be} = 0\$ (1b)
and equation (6) becomes; \$ I_{C1} + \frac{V_{R1}}{R_{1}} + \frac{V_{in} + V_{R1} - V_{be}}{R_{in}} + gm V_{be} = 0 \$ (6b),
to find \$ I_{C1} \$ we need to use that; \$ I_{C1} = C_1 \frac{d}{dt} V_{C1} \$ (7),
since \$ V_{C1} = V_{R1} \$ (8) substituting (8) into (7) we can write this as; \$ I_{C1} = C_1 \frac{d}{dt} V_{R1} \$ (9).
substituting (9) into (6b) yields; \$ C_1 \frac{d}{dt} V_{R1} + \frac{V_{R1}}{R_{1}} + \frac{V_{in} + V_{R1} - V_{be}}{R_{in}} + gm V_{be} = 0 \$ (10)
or if you want the solution in the laplace/s -domain; \$ s C_1 V_{R1} + \frac{V_{R1}}{R_{1}} + \frac{V_{in} + V_{R1} - V_{be}}{R_{in}} + gm V_{be} = 0 \$ (10b).
Comment; Please do not remove your previous picture etc, but add the new one as an edit!
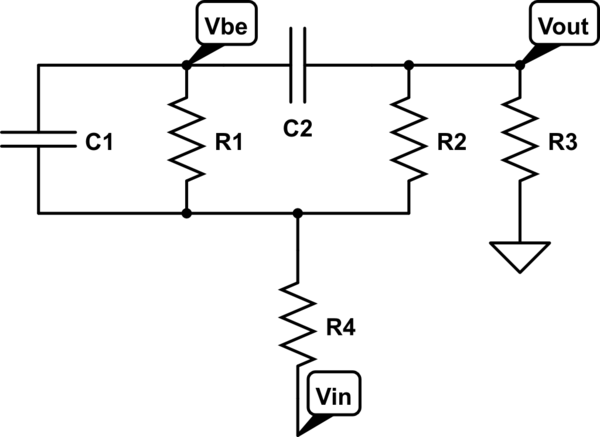

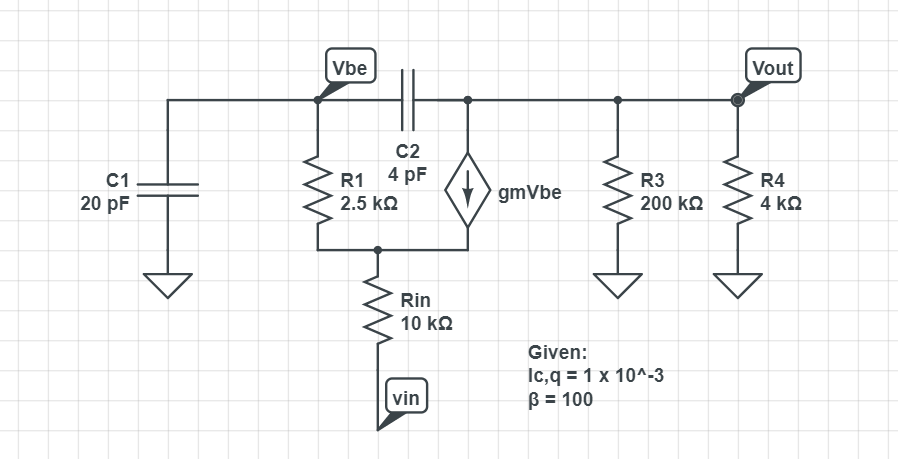
Rin,R1andgmVbe? EliminateVbefrom that node equation using thevout/vbetransfer function. \$\endgroup\$