If I have response like the following, is it still valid to say bandwidth of the circuit is 2.31kHz?
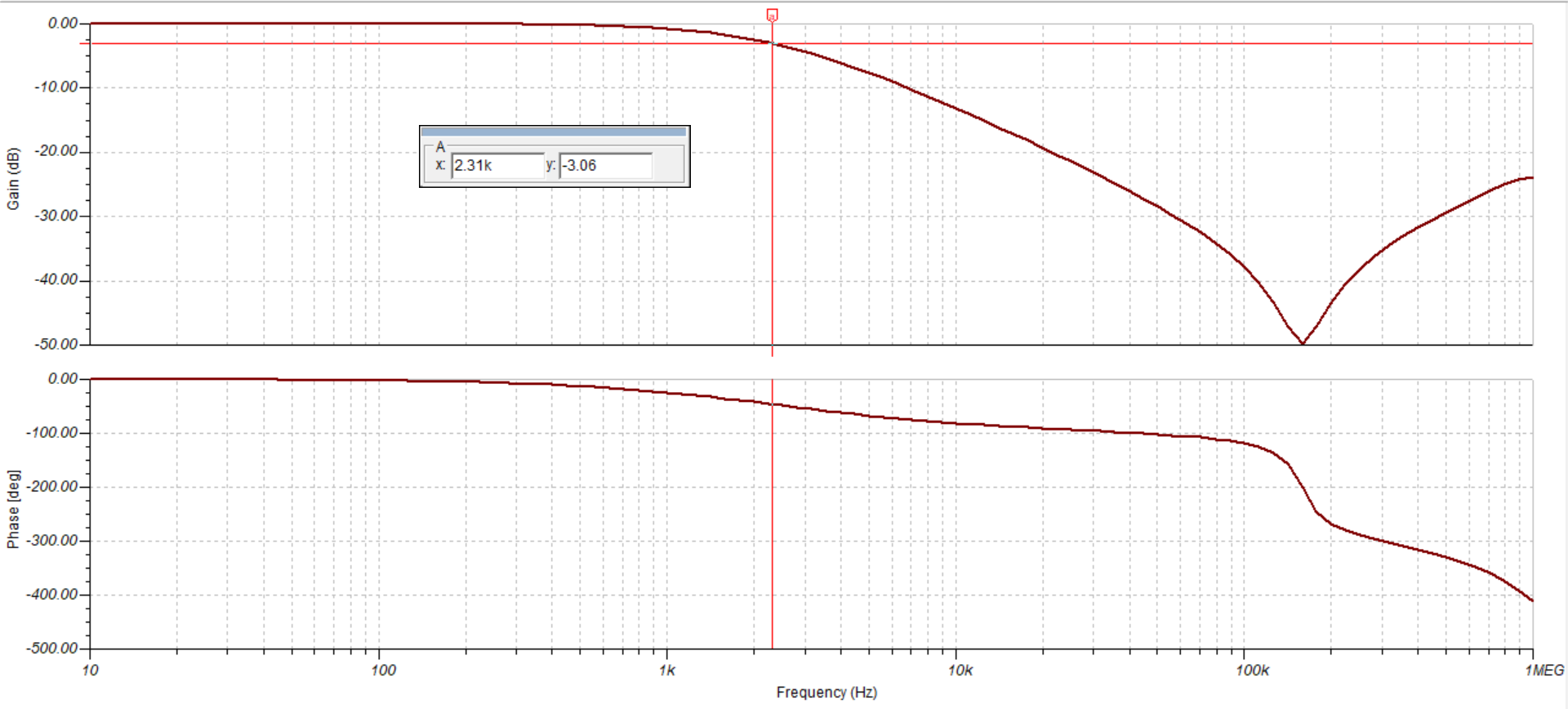
4 Answers
Is -3dB bandwidth defined for any type of transfer function?
Maybe you do not realize what -3dB actually means?
-3 dB means that the amplitude of the voltage (current as well) has dropped by a factor \$\sqrt2\$.
That means that the power (= voltage * current) has dropped a factor \$\sqrt2\$ * \$\sqrt2\$ = \$2\$.
And that is the point: the signal power at the output has dropped by a factor of 2 (compared to the passband).
This happens at (a) certain frequency / frequencies and those frequencies are all -3 dB points with which we can define a certain bandwidth.
So yes, the -3dB bandwidth can be defined for any type/shape of transfer function.
-
\$\begingroup\$ "-3dB means that the amplitude of the voltage has dropped by a factor of \$ \sqrt{2}\$" relative to what? Relative to \$V_{in} \$ at DC? Relative to \$V_{o,max} \$? Can you find the -3dB point the same way for a lowpass filter and a bandpass filter? \$\endgroup\$– CarlCommented Jul 5, 2021 at 16:18
-
1\$\begingroup\$ @Carl Relative to Vin at DC? No relative to what you consider to be the passband. dB only defines one value being relative to another value. Can you find the -3dB point the same way for a lowpass filter and a bandpass filter? Yes, as stated: ...the point: the signal power at the output has dropped by a factor of 2 (compared to the passband). \$\endgroup\$ Commented Jul 5, 2021 at 16:34
-
1\$\begingroup\$ Let's say I have a lowpass filter that is underdamped, such as \$H(s) = \frac{1}{s^2+s+8} \$. The frequency characteristic \$H(j\omega) \$ has a low frequent asymptote with -18dB gain and I suppose this is the passband. However, it also has a peak at the resonant frequency (\$\sqrt{8} = 2.8 \$) and at this point the gain is -9dB. So where would you locate the -3dB point? At -21dB? At -12dB? \$\endgroup\$– CarlCommented Jul 5, 2021 at 18:46
-
1\$\begingroup\$ @Carl There are no strict rules. In this case it is common to ignore the peak so the -3 dB (relative to the flat passband) will be the bandwidth so -21 dB. But if you want to define it differently, be my guest, as long as you make it clear what your definition is. If you prefer to define the bandwidth as the -3 dB point relative to the peak than that is your choice. But it is not common to define it like that. \$\endgroup\$ Commented Jul 5, 2021 at 18:59
-
\$\begingroup\$ really, any? What about a transfer function that never drops down to -3dB? \$\endgroup\$ Commented Jul 6, 2021 at 9:31
"Is -3dB bandwidth defined for any type of transfer function?"
No - in general, it is not. Of course, you CAN define the -3dB bandwidth for any transfer function - if it makes sense. However, for some filter functions (other than Butterworth or Thomson-Bessel response) it is common and agreed practice not to use the 3dB point for defing the bandwidth.
Example: Chebyshev or Cauer (elliptical) responses. In these cases, the end of the passband is defined by the ripple within the passband (when the magnitude in case of a lowpass crosses the value for DC to the last time). For highpass responses this specification applies to the magnitude for very large frequencies.
Because bandpass and bandstop filters can be seen as a lowpass-highpass combination, the above applies to these transfer functions as well.
Yes it is accurate to define the -3 dB BW with the same value in both cases.
When you have complex bandstop characteristics, then you would define those separately with the frequency and Bandstop attenuation.
-
\$\begingroup\$ Not directly related but say my gain at 0Hz had been 5dB, then I would have been looking at 2dB for bandwidth right? \$\endgroup\$ Commented Jul 5, 2021 at 14:47
-
\$\begingroup\$ Not directly related but say my gain at 0Hz had been 5dB...2 dB IF 0 Hz is what you define as "passband" (and for a lowpass filter in your question, this is the case) then yes, at the end of that passband the transfer would be 3 dB lower. So if the transfer is + 5 dB at 0 Hz then the edge is where the transfer is + 5 dB - 3 dB = + 2 dB. \$\endgroup\$ Commented Jul 5, 2021 at 14:55
-
\$\begingroup\$ Yes -3dB Voltage is always the “half-power breakpoint” so it is always relative to passband gain. \$\endgroup\$– D.A.S.Commented Jul 5, 2021 at 15:06
You can choose the bandwidth to be anywhere your requirements ask for it: -3 dB (typical Butterworth and 1st order), -0.1 dB (typical 0.1 dB ripple Chebyshev), +0.5 dB (unusual, but it can be if ripple exists and is higher than 0.5 dB, while the passband has 1+δ, instead of the usual 1-δ), -40 dB (e.g. unscaled inverse Chebyshev), etc. It's not you who defines that, it's the requirements, so if your filter needs to have a bandwidth at -1.23 dB then that's where it will be considered.
In the examples above, I said that -3 dB is typical for Butterworth and 1st order filters. This is true, at least mathematically. In practise, even then exists frequency scaling, which is why custom bandwidths can be chosen (like the -1.23 dB).
Therefore your question:
is it still valid to say bandwidth of the circuit is ...?
is not the right one unless your requirements specify that the bandwidth needs to be there.
To show what I meant, here's a 2nd order Cauer/elliptic with 1 dB ripple and 20 dB attenuation, and the possible points where the bandwidth can be chosen:
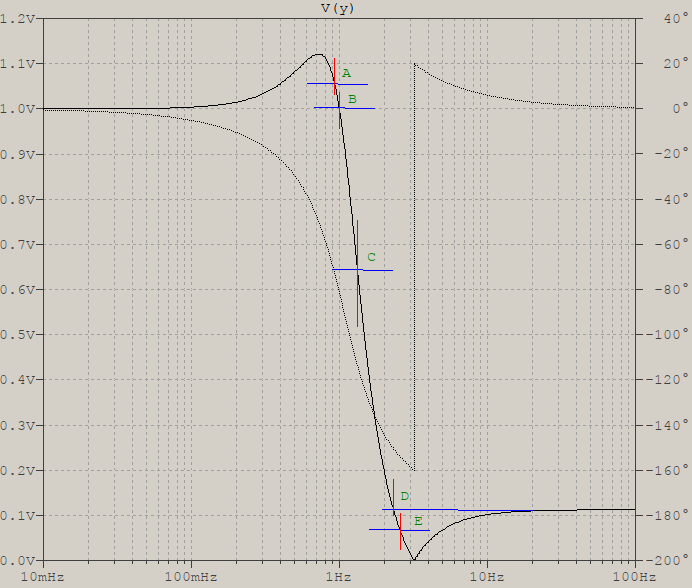
Points A and E are in the ripple zone, which you won't see in practice, but it can be done. Point C is anywhere in the transition region, which can be met in practice, though I probably have too many fingers at one hand for how many cases there are. Point D is right at the stopband point -- unscaled inverse Chebyshev filters default there -- and it can be a requirement in practice (e.g. the minimum end for the highest harmonic, passband doesn't matter much, only external harmonics). The most common point (for filters with ripple in the passband) is B, or the end of the ripple, while C (for points from -6 dB, up) is most common in filters without ripple in the passband.
