I was learning about the difference between op-amp slew rate and bandwidth. Everywhere it says slew rate is a large signal parameter and bandwidth is a small signal parameter.
I have few questions:
What is meant by large/small here, because large/small are relative terms? What is the reference for large and small?
When do we need to give priority to slew rate compared to bandwidth and vice versa?
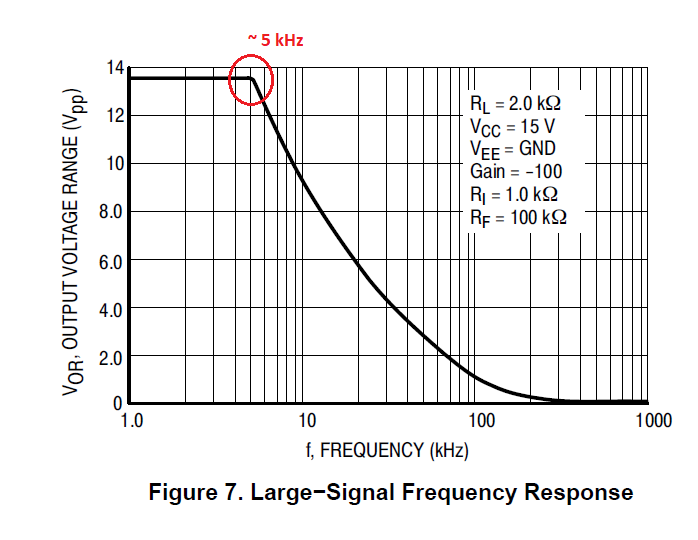
Here you have an answer via a graph which is present in the specification of the op-amp or you can calculate it. (example of LM324 https://www.onsemi.com/pdf/datasheet/lm324-d.pdf figure 7)
LM324 is a "new replacement" of old LM741. The slew rate are the same, but I did not find the graph for LM741, but only slew rate in the datasheet.
Signal input, \$V = A . sin( 2\pi ft )\$
Take derivative ...
\$dV/dt = 2\pi fA . cos( 2\pi ft)\$ --- this is maximum when \$ t=0\$
So, \$(dV/dt)_{max}\$ = \$2\pi fA\$ --- this a hyperbolic function: \$A\$ vs \$f\$
\$ \therefore f_{max} = \text{solve } \{(dV/dt)_{max} = \text{SR}\$, at \$ f=f_{max}\}\$ ; -- where \$\text{SR}\$ = op-amp slew rate
\$\implies f_{max}= \text{SR} / (2A\pi)\$
\$\text{SR}\$ for LM741 = \$0.5V /\mu s\$, and amplitude \$A = 15 V\$
\$\therefore f_{max} = 5.305 \text{ kHz}\$
At low frequencies, \$(dV/dt)_{max}\$ of "sinusoid" is lower than slew rate ... amplitude max.
At high frequencies, \$(dV/dt)_{max}\$ becomes higher ... so amplitude must be lower ...
The breaking point is where \$(dV/dt)_{max} = \text{SR} \$.
I called fmax because it is the highest frequency you can use the full range of output swing. it is also the breakpoint of the "hyperbolic" curve.
How using the \$(dV/dt)_{max}\$. Examples : amplitude \$A = 15 V\$
@ \$50 \text{ Hz}\$, \$(dV/dt)_{max}\$ = \$2\pi fA = 4710 < \text{SR} = 500000 V/s\$ or \$0.5V /\mu s\$ ...
@ \$5000 \text{ Hz}\$, \$(dV/dt)_{max}\$ = \$2\pi fA = 471000 <= \text{SR} = 500000 V/s\$ or \$0.5V /\mu s\$ ...
Amplitude (without distortion) vs Frequency plot:
At low frequencies, dv/dt max of "sinusoid" is lower than slew rate ... amplitude max How do you verify this using the graph?
\$\endgroup\$
It's an approximation, so the definition is also approximative.
Typically "small signal" means signal voltages and current are small enough relative to DC operating point values that the circuit can be modeled by linear equations, therefore neglecting changes in circuit characteristics due to changes in operating point.
For example, in an opamp, in small signal conditions the output voltage will be, say at least 10x lower than the supply voltage. If output current is below output stage bias current so no output devices turn off, then it is a good approximation. If output current is higher so the output stage goes into class-AB it will still work pretty well. If output current is high enough to make the output stage hit any sort of limit, like transistors losing hFe at high current or overcurrent protection, then it definitely is not small signal.
It is possible to measure the small-signal characteristics with the output near clipping. For example if you measure the frequency response and pahse margin of an opamp, it will be different when the output is centered between the rails, and when it is close to clipping. The amplitude of the test signal should be small enough: for example, if it is measured 1V from clipping, then the signal should not be 1V, otherwise it will reach clipping every period. It should be 10-100x lower.
Slew rate is defined as a large signal test because the input signal is supposed to have a fast edge that turn off one transistor in the input stage. When transistors turn on and off, linear approximations do not apply, so it is not small signal conditions.
