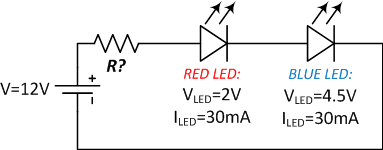
Resistance isn't "encountered" in the sense that you encounter a road block as you travel. Resistance is established instantly everywhere in the circuit, and determines as a whole what current flows. The current entering a chain of resistors or diodes is the same as the current leaving it, and it doesn't get slower as it encounters each resistance.
Current gets reduced everywhere in the path, by the total resistance everywhere along that path. In this sense resistance is like an obstruction in the road, but you have to understand that all traffic everywhere along the road is slowed, not just in the vicinity of the blockage. You could add another blockage a mile further down the road, and you affect the speed of traffic everywhere along the road.
Voltage doesn't "travel" anywhere, it doesn't "arrive" at places, it doesn't go through things. Voltage is something that exists at a point (or more precisely, a voltage difference exists between two points). Voltage is established instantly at every point in a circuit, and while voltage does depend on the obstacles along a current path, it must not be considered a "travelling" thing.
Putting all this together, you can understand that it doesn't matter what order the resistances or diodes appear in a path, the current will not change because you swapped the locations of things. The voltage difference across those things doesn't change because you swapped their positions. The total resistance to current along that path doesn't change depending on the order of the things in it.
So, it should be no surprise that the following three circuits all do exactly the same thing, develop the same voltages across the various components, and have the same current flowing in every case:
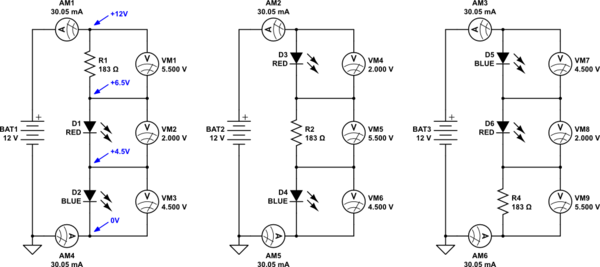
simulate this circuit – Schematic created using CircuitLab
Every blue LED has 4.5V across it, every red LED has 2.0V across it, and every resistor has 5.5V across it. In every case, the current leaving the battery at the top, and entering the chain, is the same as the current leaving the chain at the bottom and returning to the battery.
Do not confuse voltages across things with absolute voltages quoted anywhere. I've done this for the first circuit, where the labels in blue are absolute potentials, not potential differences. Starting at the bottom, I've declared the lowest node to have 0V, which you can also tell by the ground symbol at that same node.
As you cross each component the voltage changes by an amount equal to the voltage difference across the component you traverse. Jumping from the 0V node across D2, the voltage rises by 4.5V, so the voltage at that node is 0V + 4.5V = 4.5V. Jump across D1, and the voltage rises a further 2V, so at the top of D2 we can say the voltage is 4.5V + 2.0V = 6.5V. The last jump across R1 incurs a rise of another 5.5V, so the top node must be 6.5V + 5.5V = 12V. I hope it's completely clear, the contextual difference between declaring a voltage "at" some point, and a voltage difference that exists "across" or "between" two points.
The correct model
Not one of the trillions upon trillions of charges making the journey around is being slowed down because it's passing through something difficult to traverse. There's nothing happening in that loop that that says "first come first serve". Every charge is slowed everywhere by all the obstacles, simultaneously. Every voltage is established instantly, depending on the component, not depending on who's first in line.
If you have any ideas in your head that do not fit with this model, you must drop those ideas immediately, right now, because they are bad things taught by people who either don't understand themselves, or who are trying to explain things to a five year old.
Note: I feel I should point out that when you start dealing with really high frequencies, things get way more complex, and some phenomena related to these "bad" ideas might actually become significant, but not in these early stages of learning.
For now, learn these three things, and understand them like your life depends on it:
- Ohm's Law
- Kirchhoff's Current Law
- Kirchhoff's Voltage Law
They should be a lot easier to deal with once you've dispensed with all the rotten models which don't fit or work. If I haven't made this clear yet, remember that all voltages and all currents are conditions that are established everywhere, simultaneously. All the equations relating them are all true at the same time, and must be solved simultaneously using all the resistances and other impedances present everywhere.
Your example
Let's analyse your circuit using those 3 laws.
Kirchhoff's Current Law tells us that the same current is passing at every place in the loop, through each component. We want that current to be:
$$ I = I_{BATT} = I_{R1} = I_{D1} = I_{D2} = 30mA $$
We know the voltage across the diodes, because we looked it up in the datasheet:
$$ V_{D1} = 2V $$
$$ V_{D2} = 4.5V $$
We know the battery voltage:
$$ V_{BATT} = 12V $$
We don't know the resistance yet, but we can apply Ohm's law:
$$ V_{R1} = I \times R = 30mA \times R_1 $$
Kirchhoff's Voltage Law applied here tells us that all the voltages across R1, D1 and D2 must add up to the battery voltage (we saw this when we walked up the chain to establish absolute voltages earlier). I'll write different versions of the same equation to demonstrate that we don't care about the order:
$$\begin{aligned}
V_{R1} + V_{D1} + V_{D2} &= V_{BATT}
\newline
V_{D1} + V_{R1} + V_{D2} &= V_{BATT}
\newline
V_{D2} - V_{BATT} + V_{R1} &= -V_{D1}
\end{aligned}$$
Putting everything we know into that equation:
$$ 30mA \times R_1 + 2V + 4.5V = 12V $$
Now you have everything you need to solve for R1. The real takeaway from all this is that at no point did I ever refer to the order of the components, because these are simultaneous equations, not "solve one by one in the order they appear in the loop" equations.
The only reason you might need to consider the component order, is when you need to find the absolute voltage somewhere, as we did when we "walked" up the chain adding the voltages across each component as we went. Think about that - that exercise was a (somewhat trivial and contrived, but still valid) application of Kirchhoff's Voltage Law. We saw that the voltage across two or more components must be the sum of the individual voltages across them.