How should the differential gain of an op-amp be interpreted?

Question

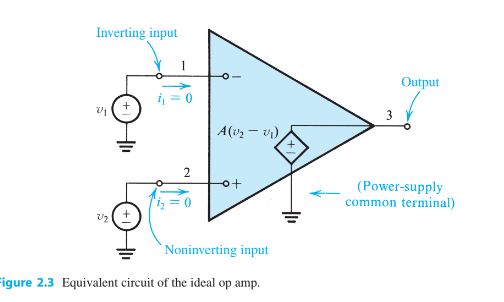
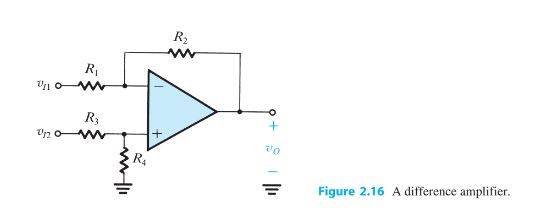
Let us consider an open-loop op-amp circuit:

The output voltage including the common mode signal is given by: $$ \mathbf{v}_{\mathbf{o}}=\mathbf{A}_{\mathbf{d}} \mathbf{v}_{\mathbf{d}}+\mathbf{A}_{\mathbf{c}} \mathbf{v}_{\mathbf{c}} $$

Here A_d and A_c refer to the differential mode gain and the common mode gain of the circuit and the V_d and the V_c refer to the differential mode voltage and the common mode voltage of the circuit.

Since the circuit is the op-amp itself, so all the parameters are for the op-amp and hence A_d becomes the differential mode gain of the op-amp which is equal to the open-loop gain of the op-amp, V_d is internal differential voltage of the op-amp, A_cm and V_cm are internal common-mode signal parameters of the op-amp. Hence,

$$ \mathrm{v}_{\mathrm{d}}=\mathrm{v}_{\mathrm{+}}-\mathrm{v}_{\mathrm{-}} $$ $$ v_{\text {c}}= \frac{v_{\text {+}}+v_{\text {- }}}{2} $$ $$ A_{\text {d}}= A_{\text {OL}} $$

Now consider the second circuit in a closed-loop configuration (negative feedback):

Output voltage including the common-mode signal is given by: $$ \mathbf{v}_{\mathbf{o}}=\mathbf{A}_{\mathbf{d}} \mathbf{v}_{\mathbf{d}}+\mathbf{A}_{\mathbf{c}} \mathbf{v}_{\mathbf{c}}=\mathbf{A}_{\mathbf{OL}} \mathbf{v}_{\mathbf{id}} $$ $$ \mathrm{v}_{\mathrm{id}}=\mathrm{v}_{\mathrm{+}}-\mathrm{v}_{\mathrm{-}} $$ $$ \mathrm{v}_{\mathrm{d}}=\mathrm{v}_{\mathrm{I2}}-\mathrm{v}_{\mathrm{I1}} $$ $$v_{\text {c}}= \frac{v_{\text {I1}}+v_{\text {I2 }}}{2}$$ $$ A_{\text {d}}= A_{\text {CL}} $$

Now in a closed-loop configuration, the differential gain of the circuit is the closed-loop gain of the circuit and is no longer equal to the differetial gain of the op-amp (also referred to as the open-loop gain of the op-amp).

The same can be said about the differential mode voltage V_d, common-mode voltage V_c and the common mode gain A_c of the circuit. The V_id is the differential voltage of the op-amp which can still be related to output voltage of the op-amp (same as th output voltage of the circuit) using the open loop gain of the op-amp.

But why is it that the internal common mode signal parameters are not included in the op-amp closed configuration circuit? I mean to say: why doesn't this equation exist?: $$ \mathbf{v}_{\mathbf{o}}=\mathbf{A}_{\mathbf{d}} \mathbf{v}_{\mathbf{d}}+\mathbf{A}_{\mathbf{c}} \mathbf{v}_{\mathbf{c}}=\mathbf{A}_{\mathbf{OL}} \mathbf{v}_{\mathbf{id}}+\mathbf{A}_{\mathbf{c}}^{'} \mathbf{v}_{\mathbf{c}}^{'} $$ $$ v_{\text {c}}^{'}= \frac{v_{\text {+}}+v_{\text {-}}}{2} $$

Why are internal common mode signal parameters not included in the internal gain equation of the op-amp? Is my interpretation of all these concepts correct?

Sidenote:In this way, CMRR (common mode rejection ratio) which is the ratio of A_d to A_c can also be defined in two says: CMRR of the op-amp and CMRR of the circuit.

References:
Opamp Differential Amplifier Wikipedia
Differential Amplifier IIT
Sedra Smith Seventh Edition (Specific pages)
Gayakwad Linear Integrated Circuits (Specific pages)

Answer 1

Why internal common mode signal parameters not included in the internal gain equation of the opamp? Is my interpretation of all these concepts correct?

You are correct in the sense that considering the the internal common mode gain would result in a more accurate model do describe the opamp and the difference amplifier.

Considering only the opamp (not the difference amplifier circuit), depending on the specific opamp, it is common to disregard the common mode gain since it frequently adds complexity to the analysis without providing practical better results. This becomes clear when you model the common mode gain as an extra input offset voltage, proportional to the common mode voltage. When you do this, frequently the actual offset voltage or offset currents may represent a more significant influence.

When you consider the difference amplifier circuit, the significance of the internal common mode gain becomes even less relevant, since the resistor value mismatch, in practice, will be more relevant to describe the circuit common mode gain.

All models are wrong. We choose the simpler ones that explain the circuit within our requirements.