Find RL circuit average power when no AC amplitude is given

Question

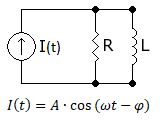
I am asked to find the average power of this RL circuit, assuming that \$R\$, \$L\$ and the frequency (\$\omega\$) and phase (\$\phi\$, can be zero) are given. But nothing is said about the amplitude \$A\$ of the alternating current source.

I can calculate the voltage across the \$R\$ and \$L\$ using Ohm's law and phasors, but I will get the amplitude \$A\$ in my expression. Then I can use the following formula of average power, depending on the phasors of voltage and current:

$$P_{\text{avg}} = \dfrac{V_m \cdot A \cdot \cos(\theta_I - \theta_V)}{2}$$

And here the \$A\$ will be squared. So we can't throw it away. Maybe there is another (unknown to me) way to solve this specific problem, or it is impossible to solve this question without the amplitude \$A\$. In fact, it seems weird to get a power value when the amplitude \$A\$ can be zero.

Answer 1

In your problem, you are not given numerical values of R, L frequency and phase (at least you have not shown them in your question). Why are you concerned that a numerical value for amplitude is not given? You simply solve the problem using symbols for all of the parameters. If values are given later, then you substitute them in your answer. Its not weird to have the power be 0 if the input amplitude is 0. That is to be expected and, in fact, is a quick check on your symbolic answer.

Answer 2

Well, the complex input impedance is given by:

$$\underline{\text{Z}}_{\space\text{i}}=\text{R}\space\text{||}\space\text{j}\omega\text{L}=\frac{\text{R}\cdot\text{j}\omega\text{L}}{\text{R}+\text{j}\omega\text{L}}\tag1$$

And the complex input current is given by:

$$\underline{\text{I}}_{\space\text{i}}=\text{A}\exp\left(-\varphi_\text{i}\text{j}\right)\tag2$$

So, the real power in the circuit is given by:

$$\text{P}=\text{V}_\text{rms}\cdot\text{I}_\text{rms}\cdot\cos\left(\varphi\right)=\underbrace{\frac{1}{\sqrt{2}}\cdot\left|\underline{\text{I}}_{\space\text{i}}\cdot\underline{\text{Z}}_{\space\text{i}}\right|}_{=\space\text{V}_\text{rms}}\cdot\underbrace{\frac{1}{\sqrt{2}}\cdot\left|\underline{\text{I}}_{\space\text{i}}\right|}_{=\space\text{I}_\text{rms}}\cdot\underbrace{\cos\left(\arg\left(\underline{\text{Z}}_{\space\text{i}}\right)\right)}_{=\space\cos\left(\varphi\right)}=$$ $$\frac{\left|\underline{\text{I}}_{\space\text{i}}\right|^2}{2}\cdot\left|\underline{\text{Z}}_{\space\text{i}}\right|\cdot\cos\left(\arg\left(\underline{\text{Z}}_{\space\text{i}}\right)\right)=\frac{\text{A}^2}{2}\cdot\frac{\text{R}\omega\text{L}}{\sqrt{\text{R}^2+\left(\omega\text{L}\right)^2}}\cdot\cos\left(\arg\left(\text{R}\cdot\text{j}\omega\text{L}\right)-\arg\left(\text{R}+\text{j}\omega\text{L}\right)\right)=$$ $$\frac{\text{A}^2}{2}\cdot\frac{\text{R}\omega\text{L}}{\sqrt{\text{R}^2+\left(\omega\text{L}\right)^2}}\cdot\cos\left(\frac{\pi}{2}-\arctan\left(\frac{\omega\text{L}}{\text{R}}\right)\right)=$$ $$\frac{\text{A}^2}{2}\cdot\frac{\text{R}\omega\text{L}}{\sqrt{\text{R}^2+\left(\omega\text{L}\right)^2}}\cdot\frac{\frac{\omega\text{L}}{\text{R}}}{\sqrt{1+\left(\frac{\omega\text{L}}{\text{R}}\right)^2}}=\frac{\text{R}\left(\text{A}\omega\text{L}\right)^2}{2\text{R}^2+2\left(\omega\text{L}\right)^2}\tag3$$