Shouldn't the total resistance of resistors in parallel be the same as the smallest resistor?

Question

I managed to confuse myself again about electricity. I just read that if you have several resistors in parallel, the total resistance is lower than the lowest of the resistors, not the equal to the lowest resistor. Intuitively, this should should be false if current takes the path of least resistance. It also means that if one of the resistors is replaced with a bare wire, the total resistance would be lower than the circuit's wire? What if the entire circuit wiring is a superconductor? Is it possible to have a resistance lower than 0?

Answer 1

Current doesn't all go down the one path of least resistance, it divides among all paths, inversely proportionally to the resistance of each path. Therefore resistors in parallel will have lower resistance than the lowest value parallel resistor.

This goes for resistors in parallel with a wire as well. A wire is just a resistor with a very small resistance.

Once you have a superconductor you have no resistance, and putting anything in parallel will not result in a negative resistance.

Another way to think about it is that elements in parallel have the same voltage across them by definition. For resistors that means that each element will have V(parallel)/R(element) amps of current flowing by Ohm's law. So clearly the current divides among the parallel resistors.

You can read about current division here.

Answer 2

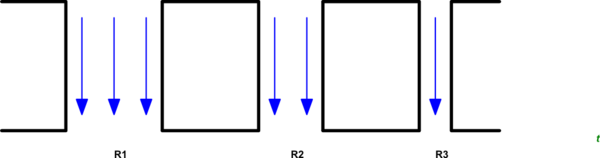
^{simulate this circuit – Schematic created using CircuitLab}

Figure 1. Three parallel paths.

Consider three roads or water pipes or corridors (or wires). R2 has a resistance half that of R3 so twice as much traffic / water / people (or current) can flow through it. R1 has one third of the resistance of R3 so three times as much traffic / water / people (or current) can flow through it.

As shown in the diagram we can have a total of six times the traffic through the combination of R1, R2 and R3 than we can through R1 alone. The combination allows higher traffic than R1 alone so the combined resistance must be lower than that of R1.

^{simulate this circuit}

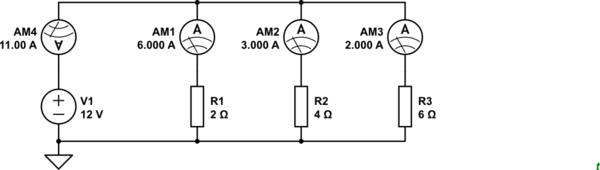
Figure 2. Three resistors in parallel. R1 is the lowest value. (AM4 is upside down to preserve polarity.)

The simulation shows that with a 12 V supply and R1 = 2 Ω we get 6 A flowing through that branch as expected from Ohm's Law, \$ I = \frac V R = \frac {12} 2 = 6 \ \text A \$.

Clearly with all three in parallel giving a total of 11 A drawn from the power supply we can calculate that the effective resistance is \$ R = \frac V I = \frac {12} {11} = 1.091 \ \Omega \$ and this is less than 2 Ω, the lowest value resistor.

Intuitively, this should should be false if current takes the path of least resistance.

1. Your intuition is not calibrated correctly.
2. The statement, while popular, is incorrect. Current takes all paths and splits inversely proportional to each path's resistance.

It also means that if one of the resistors is replaced with a bare wire, the total resistance would be lower than the circuit's wire?

Correct. Bare wires have resistance.

What if the entire circuit wiring is a superconductor? Is it possible to have a resistance lower than 0?

That's too cold for me to be interested.

Answer 3

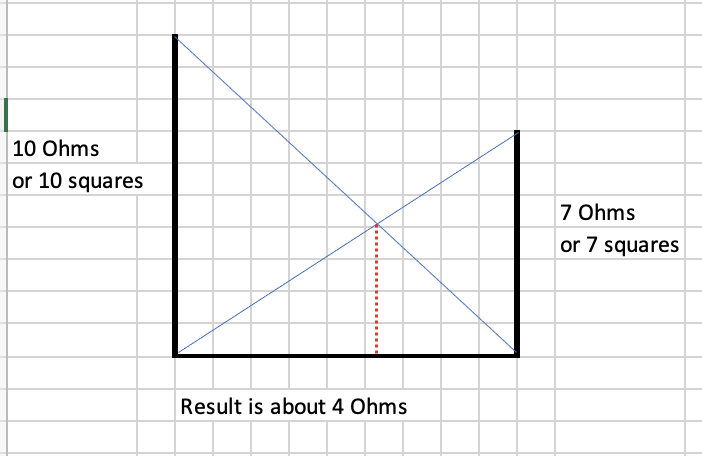
If you want a rapid check of your maths when calculating parallel resistors, the following graphic method works:

So, given a base of any length, obviously greater than zero and for accuracy I chose a value around half the value of the sum of the two resistors, draw 1 resistor with the number of squares to match its value; scale as you wish. Do the same for the second resistor.

Then join the top of the first resistor to the base of the second and the same for the second to the base of the first.

Where they intersect measure perpendicular to the base will give you the result.

You can see I missed the top of both, but close enough to prove it works.

Note: for the base length, select a length to give about a right angle between the diagonals so the intersect is "sharp"...

I will let you do the maths to see the real result.

Funny story: my Dad used this in an exam and it p1ssed the professor off enough such that he got zero on that question. Why? Because the prof did not know the method...

Answer 4

If you turn all of the faucets in your house on at the same time, does water only flow out of the faucet with the easiest path for the water? Or does it instead flow out all of them at varying rates depending on the resistance to the water flow (pipe size, faucet size, bends in the line, etc)?

Electricity acts very similar.
Water pressure -> voltage
water flow rate -> current

If you have a pipe/faucet with 0 resistance, then at that point all other faucets will stop flowing because any resistance from the other lines would be seen as too much resistance.

Answer 5

There are two fundamental electrical properties;

1. Conductors (with resistance)
2. Insulators aka dielectrics (with leakage R) and capacitance between conductors.
   • Capacitors will also conduct according the rate of change in voltage applied. Ic=CdV/dt

THe path of least resistance will conduct the most, but not all of the current. That's more accurate.

Charged clouds when the breakdwon voltage exceeds the cloud charge will conduct a stream of charges which follow the moist molecules and dust with the path of least resistance and ionize and create a tiny arc made of a daisy chain of tiny arcs. This is possibly the origin of the popular idiom. Which is why tree are a bad thing to hide under in a lightning storm but sharp lightning rods to earth provide security to buildings. As the tree is slightly closer to than the surroundings.

Answer 6

It's a lot easier to relate to parallel resistors if you flip the units.

Meet conductance, which is the inverse of resistance. The unit is siemens, and is 1 / ohms.

Say our resistors are 2, 4 and 6 ohms. What are their conductances? 0.5, 0.25 and 0.16667 siemens.

When resistors are in parallel, conductance simply adds.

0.5 + 0.25 + 0.166667 siemens = 0.916667 Siemens.

So to flip that back to resistance, 1 / 0.916667 Siemens = 1.0909 ohms.

Answer 7

Another way of looking at the problem.

^{simulate this circuit – Schematic created using CircuitLab}

Because the voltage is the same across each resistor, current will flow through each resistor. Thus, the total current in the above example is: $$ I_T = {V \over R1} + {V \over R2} + {V \over R3} $$ Using Ohm's law, we can find \$R_T\$. $$ R_T = {V \over I_T} = {V \over {V \over R1} + {V \over R2} + {V \over R3}} = {1 \over {{1 \over R1} + {1 \over R2} + {1 \over R3}}} $$ Amazing, it looks like \$ 1 \over \text{sum_of_conductances} \$ which is the equation you find in textbooks.
This example is extensible to any number of resistors in parallel.
The trick to understanding this problem, and many other circuit analysis problems, is understanding where the current flows.

I urge you to do a quick experiment and take three different valued resistors (use values between 100 and 1000 ohms), connect them in parallel across a power supply (perhaps 5VDC) and perform the following steps:

• Measure the voltage across each resistor
• Measure the current flowing through each resistor. Does this correspond the calculated current through each resistor?
• Sum up the measured currents and calculate the total resistance. Does this correspond to the calculated resistance and the measured resistance?