Impedance matching method in multilevel inverter

Question

I want to use a five-level inverter as an excitation source for magnetostrictive detection,which should be able to output a 100kHz sinwave. I learned that only if the output impendence of the source is equal to the load, can this source get maximize power transfer. As this works on magnetostrictive detection, the load type can be consider as an inductance.So I wonder maybe an impendence matching network is necessay.

In the post below, I introduced my inverter in detail.

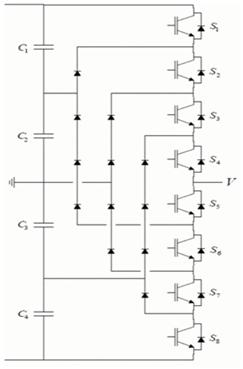
Diode-clamped five-level inverter simulation with PSpice

Here is how the inverter looks like.

To design a matching network, I should firstly calculate the equivalent output impedance of the inverter. But I don't know much about deriving equivalent impemdance of a five-level inverter.

Does anyone can help me about that? Thanks a lot.

Answer 1

I learned that only if the output impendence of the source is equal to the load, can this source get maximize power transfer.

Nearly right - if the resistive impedance of the load matches the output resistance of the source (i.e. the other way around to what you said) then you will get maximum power transfer. I'm saying it this way because clearly if the load is 1 kohm and the source impedance is 1 kohm, you won't get as much power transferred as when the source is a lot less than 1 kohm. Do you see the difference here?

the load type can be consider as an inductance. So I wonder maybe an impendence matching network is necessary.

I see no reason to have an impedance matching network. Also, if the load is basically inductive then power transfer goes out of the window because pure inductors (or capacitors) do not consume power.

To design a matching network, I should firstly calculate the equivalent output impedance of the inverter. But I don't know much about deriving equivalent impedance of a five-level inverter.

This isn't required to be done.