Experimental material impedance measurement

Question

Imagine we have a square-shaped conductive material to which we connect 3 electrodes E1, E2, and E3. Suppose we assume for this material a 'pi' two-port network model with impedances Za, Zb, and Zc (figure below), i.e., an electrical impedance between each pair of electrodes.

I was wondering what would happen if we take an LCR meter and measure impedance between electrodes E1 and and E2 while E3 is not connected to anything. Would we measure just Za or instead Za in parallel to (Zb and Zc) in series?

Plate illustration:

Answer 1

Obviously you expect something rigorous which proves the already repeated claim "you must take into the resistance between E1 and E2 all parts of your model, also the series of Zb and Zc"

We must start by checking is the delta model made of three resistors Za, Zb and Zc at all useful. Physicists fortunately have proven that the plate can be modeled with a big resistor network (= a finite element model) and it can be used to solve how currents and voltages actually distribute inside the plate. The result becomes more accurate if the sizes of the elements are reduced (and the number of the elements is increased).

A coarse model:

I named terminal E3 a GND because the circuit theory allows one node to be the GND. That makes the model of the plate essentially to a 2 port.

After believing that a resistor network really can present the plate as accurately as wanted (by increasing the number of elements) we can apply a circuit theory fact: Any passive resistor 2-port can be reduced to an equivalent three resistor 2-port; as well to a vye as to a delta:

Proving it needs some tricky manipulations of the circuit equation matrices. I must skip it, but university level circuit theory books have it.

When it's shown that a delta circuit model behaves like your plate when the plate is used as a circuit we have no excuses to forget any of the three resistors in the model when we calculate the resistance between E1 and E2.

You actually wrote of impedances, not resistances. If the frequency is high enough all circuit models become finally useless because they do not take into the account wave effects including radiation. At reasonably low frequency the reduction to a delta is OK, but the impedances depend on the frequency.

Answer 2

I was wondering what would happen if we take an LCR meter and measure impedance between electrodes E1 and and E2 while E3 is not connected to anything. Would we measure just Za or instead Za in parallel to (Zb and Zc) in series?

You would measure the latter just like you were measuring this: -

Answer 3

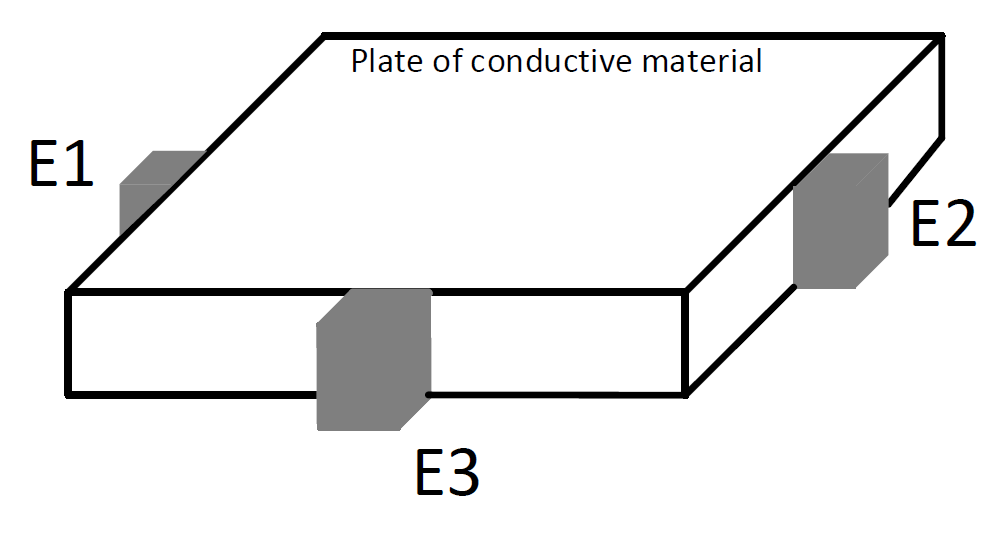
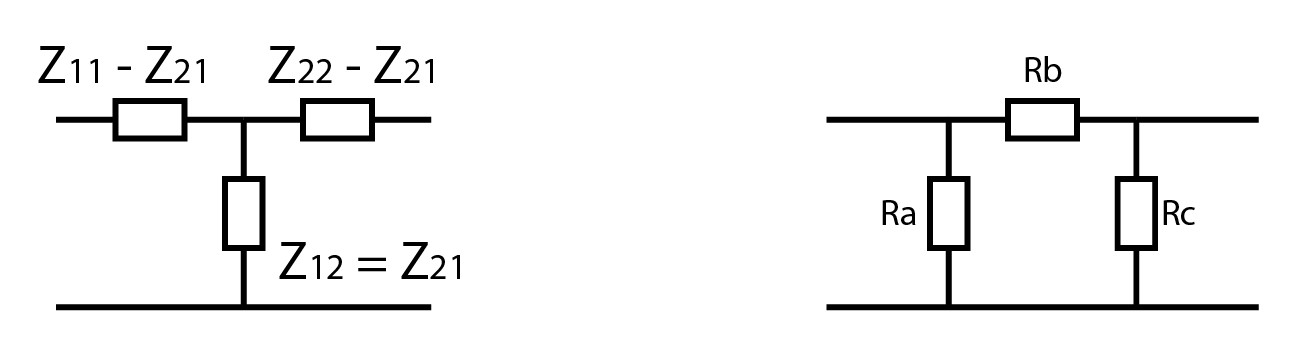Imagine you have a black box with three terminals, and you're told it only has resistors inside it, connected to all three terminals. How much information about the inside can you glean from resistive measurements at the three terminals

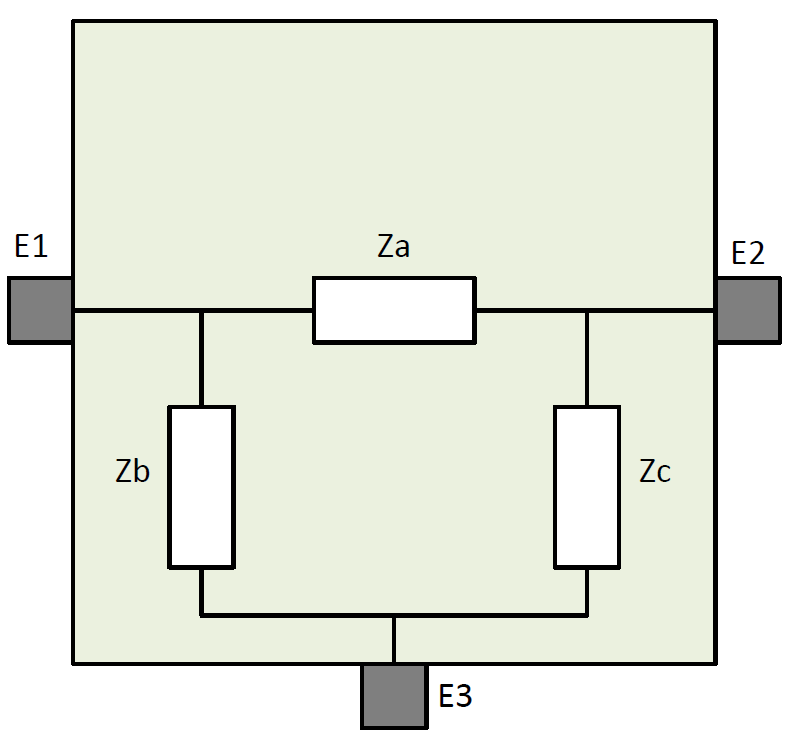
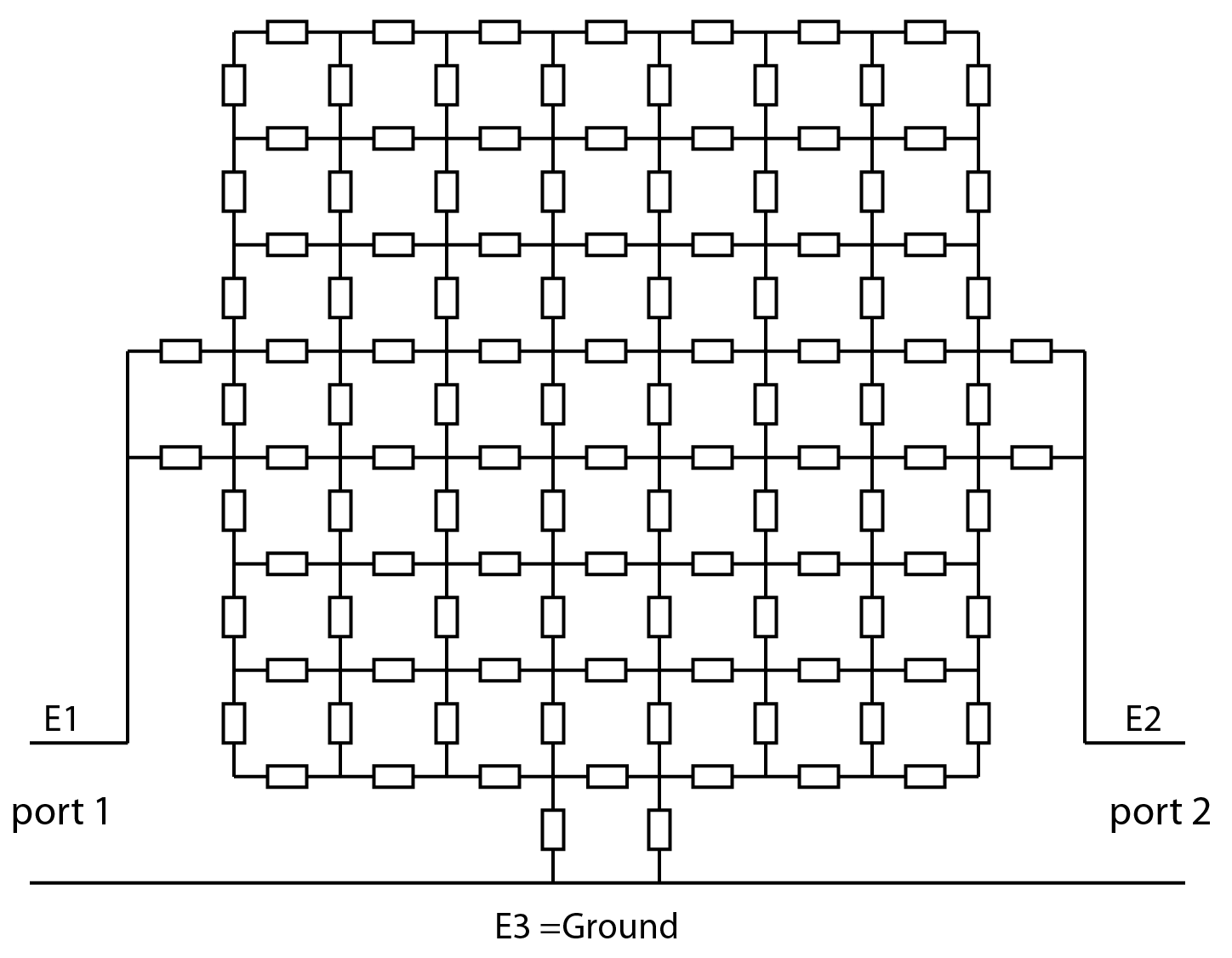
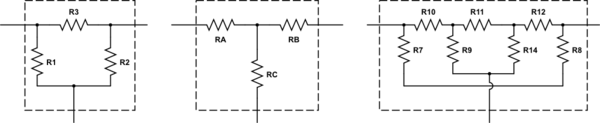
You can model it in an infinite number of ways. The first two shown below are the simplest, and capture all of the information you can gather through measurements. You can riff to your heart's content on the third way, adding more and more resistors to get the other infinite number of models. But, with three independent measurements, you can only determine three scalar values within the black box. With the first two simple arrangements, that means you can resolve all three model resistor values. With the third, you can't resolve individual resistor values.

^{simulate this circuit – Schematic created using CircuitLab}

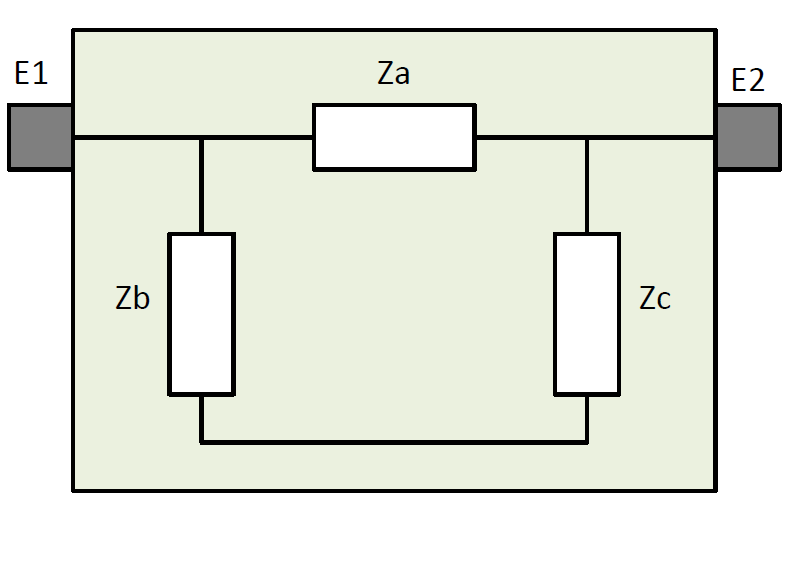
The first configuration is called a Delta ( or Δ) arrangement, the second is a Star (or Y, or Wye), both for fairly obvious reasons. There is a thing called Wye-Delta transformation, that allows you to convert between R1/2/3 and RA/B/C. If you know 1,2,3 you can compute A B and C, and vice versa. The Y and Delta configurations are both completely equivalent ways, both completely valid models, with different values for the resistors of course, of representing any resistive mess inside the box that you want to configure.

You could even have a resistive sheet inside the box, with three terminals clipped to it. It would then be modelable as a Star or a Delta. In your OP, you have modeled it as a Delta arrangement.

With your Delta model, the easiest way to get numerical values for all three resistors is to treat it as a Wye model, measure the terminals pair-wise which gives you three equations of the form Rx + Ry = measurement, which than then be solved trivially, then use the Y-Δ transformation to get back to your Delta model.

Answer 4

One can just consider this as a 3 ports "network".

Can be simulated with a "uniform" resistor network ... here, it is simplified.

Result matrix Y is obtained by DC Dynamic Analysis of this network.

So, it is not possible to "add" simply "Za", "Zb", or "Zc".