Not an answer -- just something to consider for another time
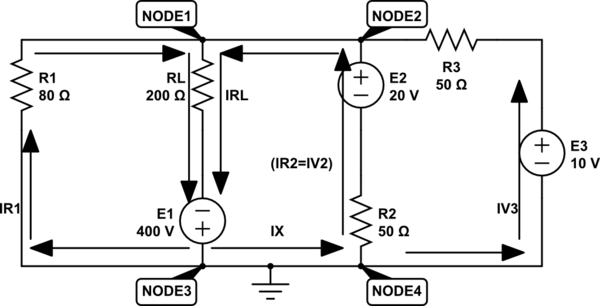
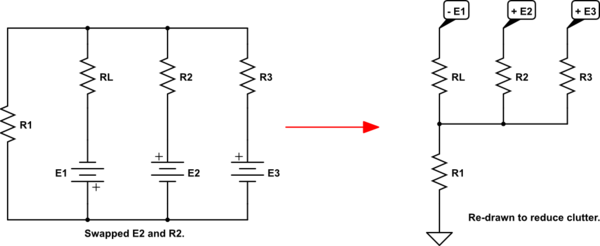
Keeping the voltages positive (you did, as evidenced by your setting for \$E_1\$), the left side is a very close schematic to yours except that I swapped the series devices of \$R_2\$ and \$E_2\$ (without affecting the analysis):
simulate this circuit – Schematic created using CircuitLab
On the right side, is the equivalent circuit but drawn without busing around power and ground lines. (I'm allowed to select exactly one node and call it ground and I think you can easily see which one I picked!) It's easier to read, now. The three KVL loops are easier to see on the right: (1) start from \$-E_1\$ and work down to ground; (2) start from \$+E_2\$ and work down to ground; and (3) start from \$+E_3\$ and work down to ground.
Or, with KCL, you only have one unknown node to solve.
An answer
There are six readily available loops, but you only need the right three of them. My suggestion is to take the obvious three:
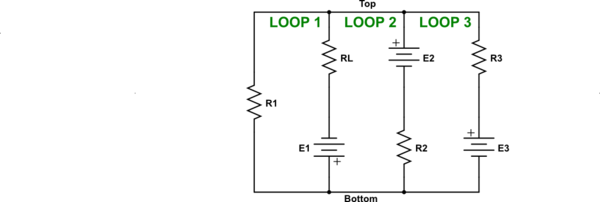
simulate this circuit
Let's assume to start in the lower left-hand corner of each, call that corner \$0\:\text{V}\$, and then walk around the loop clockwise (the direction I'd like to assume for each loop current.) The loop currents will be called \$I_1\$, \$I_2\$, and \$I_3\$.
Do note that my choice (always clockwise) isn't the choice you wrote. What matters is consistency of application, not the choice itself.
The three equations from my choice are:
$$\begin{align*}
0\:\text{V} - R_1\,I_1 -R_{_\text{L}}\left(I_1-I_2\right) + E_1 &=0\:\text{V}
\\\\
0\:\text{V} - E_1 - R_{_\text{L}}\left(I_2-I_1\right) - E_2 - R_{2}\left(I_2-I_3\right) &=0\:\text{V}
\\\\
0\:\text{V} - R_2\left(I_3-I_2\right) + E_2 - R_3\,I_3 -E_3 &=0\:\text{V}
\end{align*}$$
These do solve out correctly and they specify one set of equations that are defensible.
Now, let's go over your equations:
loop 1: E1-R1-RL
Here you are mixing apples and oranges and you wrote an expression and not an equation.
If you do start at the point in between \$R_{_\text{L}}\$ and \$E_1\$, then you would write \$E_1 - R_1\,I_1 -R_{_\text{L}}\left(I_1-I_2\right)=0\:\text{V}\$. Note that by multiplying a current (or sum of currents) by a resistance you get a voltage difference. You can add voltage differences to voltage differences and you are fine. You cannot add a voltage difference to a resistance, though. That does not make any sense.
So your expression is mixing dimensions and it should become obvious to you that you cannot do this. If you ever see that happen, you know something is very wrong.
Now, you could have written:
$$E_1-E_{R_1}-E_{R_{_\text{L}}}=0\:\text{V}$$
And that would have been okay. You still would not have the currents showing there, but at least it is an accurate statement if you also understand that \$E_{R_1}=R_1\,I_1\$ and \$E_{R_{_\text{L}}}=R_{_\text{L}}\left(I_1-I_2\right)\$.
Does that more make sense, now?