4
\$\begingroup\$

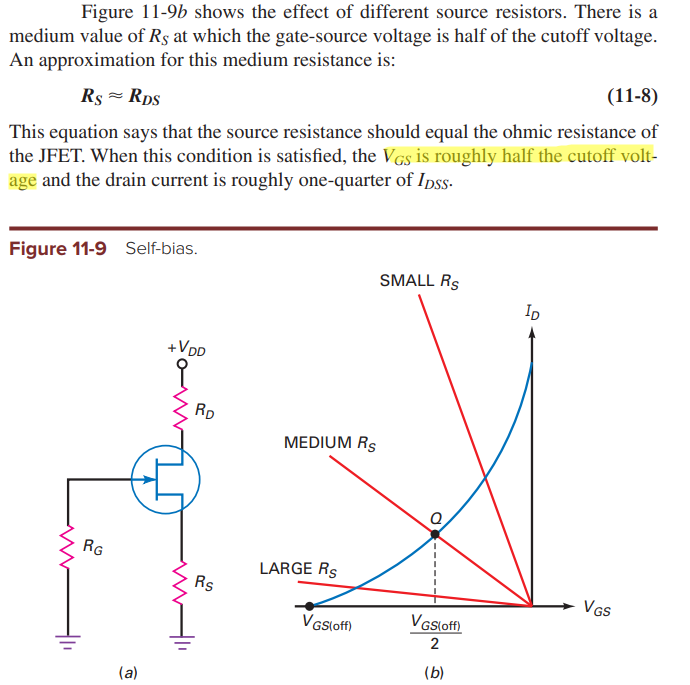
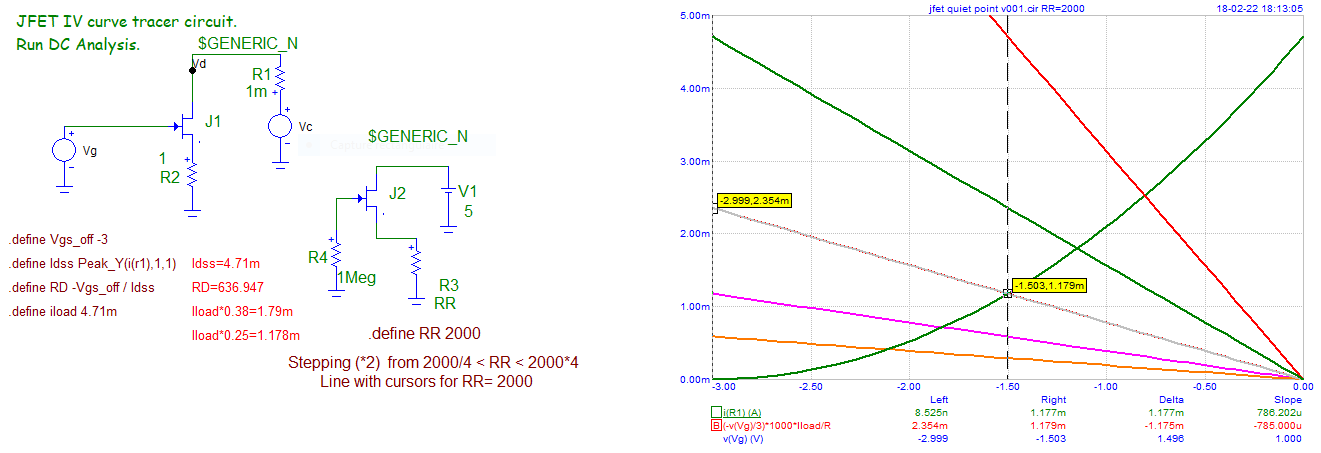
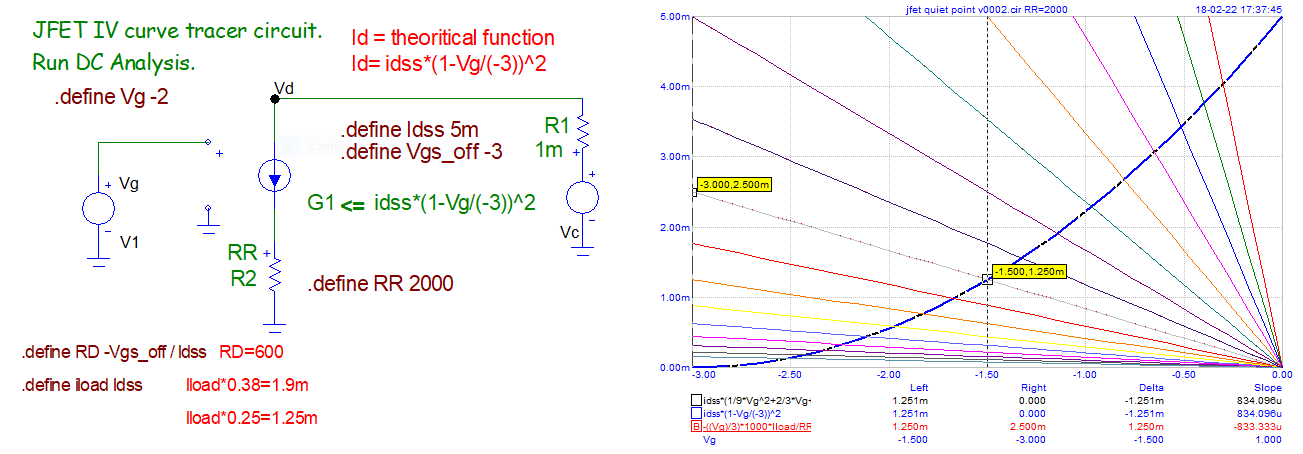
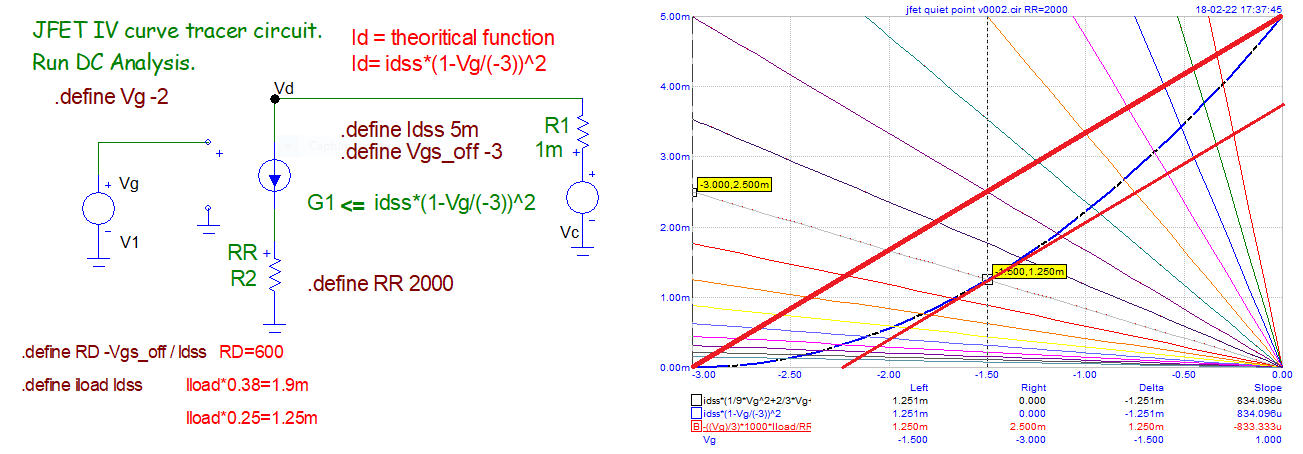
When \$R_s=R_{DS} = \frac{-V_{GS(OFF)}}{I_{DSS}}\$, my textbook seems to suggest that \$I_{D}\$ equals \$\frac{I_{DSS}}{4}\$, but I'm not able to derive this. Any help?

My work:
\$I_D = I_{DSS}(1-\frac{V_{GS}}{V_{GS(OFF)}})^2 \$

\$V_{GS} = -I_DR_s = I_D\frac{V_{GS(OFF)}}{I_{DSS}} \implies \frac{V_{GS}}{V_{GS(OFF)}}= \frac{I_D}{I_{DSS}}\$

Solving these two equations (parabola, straight line) gives me
$$\frac{I_D}{I_{DSS}} = \frac{3 \pm \sqrt{5}}{2} $$

\$\frac{3-\sqrt{5}}{2} \approx 0.38 \ne \frac{1}{4}\$

What does the author mean when he says the drain current will be \$1/4\$ of \$I_{DSS}\$ and \$V_{GS}\$ will be half the cutoff voltage?

From my work, the operating point has equal ratios: \$\color{purple}{(0.38V_{GS(OFF)}, 0.38I_{DSS})}\$

I don't see how the operating can ever be \$\color{red}{(\frac{1}{2}V_{GS(OFF)}, \frac{1}{4}I_{DSS})}\$ as the author claims.

enter image description here

\$\endgroup\$
23
  • 1
    \$\begingroup\$ textbook source: archive.org/details/ElectronicPrinciples8thEdition/page/424/… \$\endgroup\$
    – across
    Commented Feb 18, 2022 at 7:56
  • 2
    \$\begingroup\$ They are discussing the ratio of Id to Idss (and I know you know that much already from your writing above) vs the ratio of Vgs to Vgsoff. In short, if \$A=\frac{I_D}{I_{DSS}}\$ and \$B=\frac{V_{GS}}{V_{{GS}_{OFF}}}\$ then \$A=\left(1-B\right)^2\$. No need to solve two equations simultaneously to know that when \$B=\frac12\$ that \$A=\frac14\$. Solve them simultaneously if not looking to compare the ratios but instead computing the actual intersection, given some curve. But expect different values for different curves. \$\endgroup\$
    – jonk
    Commented Feb 18, 2022 at 9:42
  • 1
    \$\begingroup\$ Perhaps you should write a little more in your question, rather than writing it all down in comments. But looking forward in the text I see where you get your equation from the author. I can also see where that term comes from in the simultaneous solution, too. If you solve for Id and then divide that by Idss to get the ratio, you should see there is a factor of (Vgsoff/Idss) in the result. But it is not the only factor. So I am not sure I can explain their claim, just yet. \$\endgroup\$
    – jonk
    Commented Feb 18, 2022 at 10:04
  • 2
    \$\begingroup\$ Start with \$\frac{I_{_\text{D}}}{I_{_\text{DSS}}}=\left(1-\frac{V_{_\text{GS}}}{V_{_{{\text{GS}\,}_\text{OFF}}}}\right)^2\$, \$V_{_\text{GS}}=-R_{_\text{S}}\,I_{_\text{D}}\$, and \$R_{_\text{S}}=\frac{-V_{_{{\text{GS}\,}_\text{OFF}}}}{I_{_\text{DSS}}}\$ (their claim on the following page.) Then the claim they make is this: $$\frac{I_{_\text{D}}}{I_{_\text{DSS}}}=\left(1-\frac{R_{_\text{S}}\,I_{_\text{D}}}{R_{_\text{S}}\,I_{_\text{DSS}}}\right)^2=\left(1-\frac{I_{_\text{D}}}{I_{_\text{DSS}}}\right)^2$$ \$\endgroup\$
    – jonk
    Commented Feb 18, 2022 at 10:37
  • 2
    \$\begingroup\$ @Antonio51 Thanks! Excellent catch! But I'd love it if you wrote more, including the equation derivations so that it is captured here. :) Makes a LOT of sense. Spice should be able to capture the slope at that point with .MEAS card, I think. \$\endgroup\$
    – jonk
    Commented Feb 18, 2022 at 19:34

1 Answer 1

Reset to default
3
\$\begingroup\$

Here is a simulation with generic JFET.
At the left, the characteristic (Id vs Vg) is traced.
An after, also load line.
At the right, test with the values found of Idss.

enter image description here

EDIT: NB: Chosen points are not the same as above. (Vgsoff = -3V, Idss = 5mA)
As I was curious about the OP question, I made also a simulation with the theoretical function.
And the result seems that "RDS" is 600 Ohm = 3 V/ 0.005 mA.

enter image description here

But, I have some explanation after simulating ...
In fact, I "found" that RDS (defined as \$RDS = Vgs_off/Idss\$) is :
the value of the "dynamic" resistance at the chosen Quiet Point,
Q point in ratios (\$Vgs = 1/2 * Vgsoff, Id = 1/4 * Idss\$).

Graphical construction:

  1. draw the line through the two characteristic points of the graph (Vgsoff and Idss).
  2. Draw the tangent to the point of contact with the parabola
    at (Vgs = 1/2 * Vgsoff, Id = 1/4 Idss). This tangent is parallel to the other line and is, by definition, the dynamic resistance at the contact point.

enter image description here

This is confirmed by my calculus with Maple sheet.
Calculate the derivative (or measure it on graph) at the quiet point, and the value is exactly RDS.

enter image description here

enter image description here

\$\endgroup\$
8
  • \$\begingroup\$ Nice graphs! I have a question... Is 636.947 ohm value the same as VGSOFF/IDSS ? but from the slope of red-line it seems you're using 1600 ohm resistor at source? Also did you use datasheet to find the value of VGSOFF/IDSS ? \$\endgroup\$
    – across
    Commented Feb 18, 2022 at 14:47
  • \$\begingroup\$ Right. The second graph is ok, I think. I must correct the first, I made a "little" error ("*" replaced by "/") in the function of the load line. (not simple when the graph is on the negative x-axis ...) \$\endgroup\$
    – Antonio51
    Commented Feb 18, 2022 at 16:52
  • 1
    \$\begingroup\$ Simulations made with microcap v12 free software spectrum-soft.com/download/download.shtm \$\endgroup\$
    – Antonio51
    Commented Feb 18, 2022 at 17:36
  • 1
    \$\begingroup\$ I feel you've just demonstrated the Mean Value Theorem : instantaneous rate equals average rate at some point. Average rate of current in the interval \$(V_{gsoff},0)\$ is \$\frac{I_{DSS}}{V_{gsoff}}\$. Instantaneous rate of current is $$\frac{dI_D}{dV_{gs}} = \frac{d}{dV_{gs}} I_{DSS}(1-\frac{V_{gs}}{V_{gsoff}})^2 = I_{DSS}\cdot 2\cdot(1-\frac{V_{gs}}{V_{gsoff}})(\frac{-1}{V_{gsoff}}) = \frac{2I_{DSS}}{V_{gsoff}^2}(V_{gs}-V_{gsoff})$$ \$\endgroup\$
    – across
    Commented Feb 19, 2022 at 1:42
  • 1
    \$\begingroup\$ When \$Vgs=\frac{V_{gsoff}}{2}\$, that expression equals \$\frac{I_{DSS}}{V_{gsoff}}\$ and this is the maximum possible conductance for the jfet in ohmic region! Wow! so the author isn't entirely inaccurate haha Thanks again:) \$\endgroup\$
    – across
    Commented Feb 19, 2022 at 1:46

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.