0
\$\begingroup\$

Consider an ideal LC tank oscillator offset by an ideal battery*:

enter image description here

The core question is this: is the energy balance here maintained by the charge-voltage relationship of the battery? Energy seems to be violated looking at just the cap and inductor:

  1. The inductor current and capacitor voltage oscillate sinusoidally.
  2. The capacitor energy is quadratic in V_cap relative to ground which is offset by V_rail, while the inductor mean current is 0, so the capacitor's energy peaks are taller than the troughs while the inductor will have equal positive and negative energy peaks.

This is where the asterisk (*) comes in. I'm not sure what an "ideal battery" model actually entails. If it holds voltage constant, is it inherently non-physical? How do real-world batteries behave?

** This is motivated by an actual design decision I am making for a clamping diode (**) to know the expected power dissipation from clamping positive overshoots vs. negative ones near ground.

\$\endgroup\$
3
  • \$\begingroup\$ In your energy balance, you will need to consider the energy flowing into and out of the battery as the LC oscillates. You can choose a battery with an ESR much, much lower than the impedance of the L and C, so you get 'ideal' battery behaviour. Or you can replace the battery with an arbitraily (infinitly) large ideal capacitor. \$\endgroup\$
    – Neil_UK
    Commented Apr 15, 2022 at 8:31
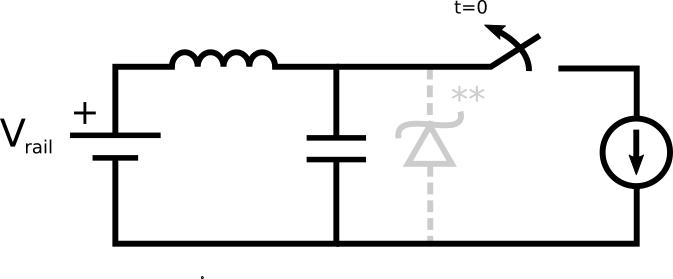
  • \$\begingroup\$ @Neil_UK Yep! Realized that just too late after making the question and kicking myself for it. I'm surprised that the difference between the cap and inductor power really is just an i_L(t)*V_rails term! Thanks for the pointer. \$\endgroup\$
    – concat
    Commented Apr 15, 2022 at 8:39
  • \$\begingroup\$ If it holds voltage constant, then a current in one direction is dumping some joules of VI * t energy out of the battery, and reversing the current makes the battery suck joules of VI * t energy into itself. Caps do similar, but where the voltage across the plate is changing as the EM energy is absorbed or radiated from its plates, from or to the external circuit.) \$\endgroup\$
    – wbeaty
    Commented Apr 15, 2022 at 9:10

1 Answer 1

Reset to default
1
\$\begingroup\$

As @Neil_UK pointed out, I forgot to consider the energy flow to and from the battery, which just has power of P_bat = -i_L(t) * V_rail. This balances the power.

Given I_L = I = i_0 * exp(jwt), w = 1/√LC:

V_C = -jI / (Cw) + V_rails 
    = -√(L/C) * jI + V_rails
E_C = C * Re[V_C ^ 2] / 2
    = C * Re[(-√(L/C) * jI + V_rails) ^ 2] / 2
    = Re[(-√L * jI + √C * V_rails) ^ 2] / 2
P_C = Re[(-√L * jI + √C * V_rails) * (√L * I * w)]
    = -√(L/C) * Re[jI^2] + V_rails * Re[I]

E_L = L * Re[I^2] / 2
P_L = Re[jI^2] * L * w
    = √(L/C) * Re[jI^2]

P_bat = -V_rails * Re[I]

Therefore P_C + P_L + P_bat = 0.
\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.