1
\$\begingroup\$

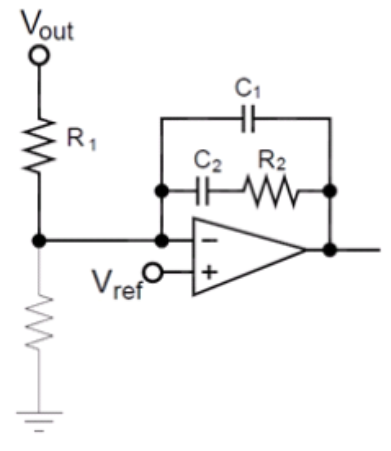
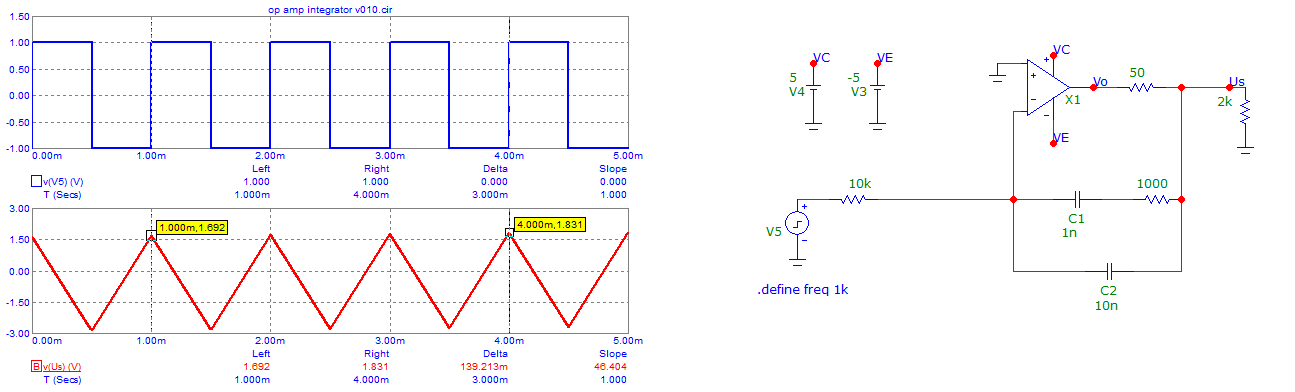
I'm looking to find the transient response to a step impulse of a type-2 compensator.

Type II compensator

I already found the transfer function, but when it comes to finding the transient response by using the inverse Laplace transform I don't get a good result. Anyway, here is the transfer function:

$$ Hd(s)=\frac{1+R_2C_2 s}{s(R_1(C_1+C_2)+R_1R_2C_1C_2s)} $$ Reacting to a step function I multiply it by 1/s which gives: $$ Hd(s)=\frac{1+R_2C_2 s}{s^2(R_1(C_1+C_2)+R_1R_2C_1C_2s)} $$ Then using partial fraction I get: $$ \frac{1+R_2C_2 s}{s^2(R_1(C_1+C_2)+R_1R_2C_1C_2s)}=\frac{A}{s^2}+\frac{B}{s}+\frac{C}{R_1(C_1+C_2)+R_1R_2C_1C_2s} $$ When I perform the inverse Laplace transform on the result it gives an unexpected unreal time response. $$ Hd(t)=\frac{(R_1R_2C_1C_2)^2-(1+R_2C_2)(R_1(C_1+C_2))(R_1R_2C_1C_2)e^{\frac{-(R_1(C_1+C_2)t}{(R_1R_2C_1C_2)}}}{(R_1(C_1+C_2))^2(R_1R_2C_1C_2)}+\frac{(1+R_2C_2)(R_1(C_1+C_2))-R_1R_2C_1C_2}{(R_1(C_1+C_2))^2}+\frac{t}{R_1(C_1+C_2)} $$ It's quite a big formula, but what bother me is the last term because the response should not be linear.

Has anybody already calculated the transient response of a type-2 compensator?

\$\endgroup\$
2
  • 2
    \$\begingroup\$ Have you noted that this circuit is an "integrator"? \$\endgroup\$
    – Antonio51
    Commented Aug 11, 2022 at 8:44
  • 1
    \$\begingroup\$ You will make your life easier if, in the original formula for \$Hd(s)\$, you replace: \$R_2C_2=\tau_2,\;R_1(C_1+C_2)=\tau_1,\;R_1R_2C_1C_2=\tau_{12}\$, because then the step response will show up in a more readable form:$$s(t)=\dfrac{t}{\tau_1}+\dfrac{\tau_1\tau_2-\tau_{12}}{\tau_1^2}\left(1-\text{e}^{-\frac{\tau_1}{\tau_{12}}t}\right)$$The presence of the free term with \$t\$ tells you it's an integrator, if the fact that it has no DC path in the feedback, only capacitors, won't. \$\endgroup\$
    – a concerned citizen
    Commented Aug 11, 2022 at 10:09

1 Answer 1

Reset to default
0
\$\begingroup\$

This circuit is "fundamentally" an "integrator".
So the last term shows that output is growing with time.
The last term seems thus ok.

enter image description here

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.