2
\$\begingroup\$

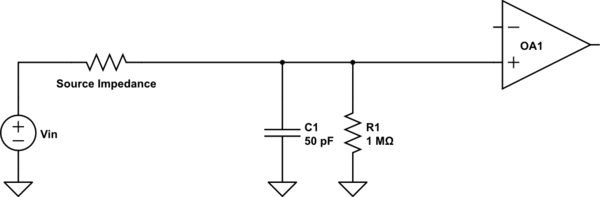
The manual of a signal analyzer I am working with states that the input impedance is \$ 1 \ {\rm{M\Omega}} + 50 \ {\rm{pF}} \$, the manual also states that the input noise is \$< 10 \ {\rm{nV_{rms}/\sqrt{Hz}}}\$.

If I assume we are dealing with Johnson-Nyquist noise (which is an RMS voltage) $$V^{\rm{(JN)}}_{\rm{rms}} = \sqrt{4 k_{\rm{B}} T R \Delta f} \text{,}$$ and that we are working with room temperature conditions (\$T = 293 \ {\rm{K}}\$), to get input noise of \$< 10 \ {\rm{nV_{rms}/\sqrt{Hz}}}\$ would require an impedance of about \$6 \ {\rm{k \Omega}}\$.

Can anyone help me see where this discrepancy arises from, what am I missing here?

\$\endgroup\$
1
  • 1
    \$\begingroup\$ renesas.com/us/en/document/apn/… gives an excellent discussion of the relationship between opamp internal noise sources and any external resistors around the opamp. \$\endgroup\$
    – glen_geek
    Commented Sep 30, 2022 at 13:28

1 Answer 1

Reset to default
2
\$\begingroup\$

The noise density value is the input-referred noise of the signal amplifier.

The input impedance is in parallel with the signal. So for a low impedance signal, the device input impedance becomes irrelevant.

If you do not connect any signal source, however, the input impedance becomes the signal source. In that case, the measured noise will be the Johnson noise of the input impedance and be much higher indeed than 10 nV/rtHz (at low frequencies).

schematic

simulate this circuit – Schematic created using CircuitLab

\$\endgroup\$
8
  • \$\begingroup\$ Okay this is helpful and explains a lot to an extent. So if I don't connect any signal source to my input, would my noise then be \$\sqrt{4 k_{\rm{B}} T \ 1\ {\rm{M\Omega}} \ \Delta f}\$? Or do I also need to consider the capacitance? \$\endgroup\$
    – user27119
    Commented Sep 30, 2022 at 14:08
  • \$\begingroup\$ Also, could you expand upon what you mean by "at low frequencies" surely the noise is only bandwidth dependant? \$\endgroup\$
    – user27119
    Commented Sep 30, 2022 at 14:33
  • \$\begingroup\$ @N.B. yes if there is no signal source, the input noise density is √(4 kB T 1Mohm). The noise density formula has no bandwidth. And this noise density depends on frequency because at higher frequency the 50 p cap shorts the 1 M resistor, and the noise density is thus very small at high frequency. \$\endgroup\$
    – tobalt
    Commented Sep 30, 2022 at 14:36
  • \$\begingroup\$ Thanks for the capacitance explanation. Surely the bandwidth must come into play? If I connect nothing to my analyser and measure an FFT spectrum, then the resultant spectrum be bandwidth dependant? After all the "input signal" in this case will be a voltage and \$\sqrt{4 k_{\rm{B}} T R}\$ will have units of \$\rm{V/\sqrt{Hz}}\$ \$\endgroup\$
    – user27119
    Commented Sep 30, 2022 at 14:47
  • \$\begingroup\$ @N.B. yes V / √Hz is the unit of spectral voltage noise density. And this is frequency dependent. If you want to calculate Vrms noise, then you integrate the squared noise density. In simple case of constant noise density (which usually isnt the case) you can thus simply multiply by √BW. \$\endgroup\$
    – tobalt
    Commented Sep 30, 2022 at 15:06

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.