2
\$\begingroup\$

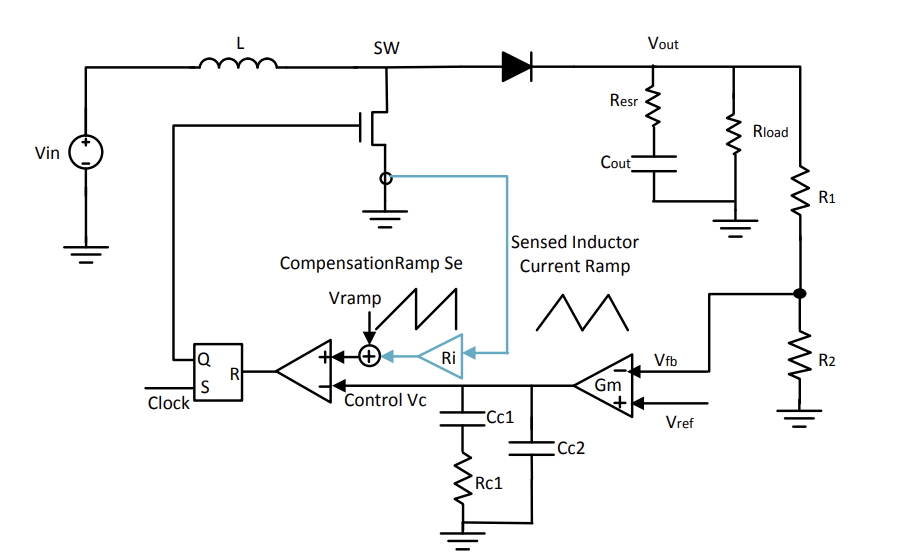
The image below is about peak current mode control for a boost converter. Someone said that it's easier to make it stable by feedback a very small of output like 1/10 Vout (or R2 << R1).
However, he couldn't explain why. Can anyone explain?

EDIT:

From the comment, I realized that the statement is wrong. So I'd like to edit my question a bit. Let's assume that you can have any reference voltage you want, then how would the feedback resistors affect the stability? Or even you have a fixed voltage reference, you still have infinite choices for R1, R2 to get the same feedback ratio. How would the selection affect the stability?

enter image description here

\$\endgroup\$
8
  • \$\begingroup\$ @tobalt I suppose that it works as open loop without regulating the output in that case. \$\endgroup\$
    – internet
    Commented Nov 20, 2022 at 19:47
  • \$\begingroup\$ ride a bicycle with your eyes closed to understand the importance of feedback \$\endgroup\$
    – jsotola
    Commented Nov 20, 2022 at 19:49
  • \$\begingroup\$ lol, so that is wrong then? \$\endgroup\$
    – internet
    Commented Nov 20, 2022 at 19:50
  • \$\begingroup\$ Is the question "why there is feedback at all" or "why is the feedback a divided down version of output instead of the output directly"? \$\endgroup\$
    – Justme
    Commented Nov 20, 2022 at 19:53
  • 1
    \$\begingroup\$ @internet, the division ratio of the resistive divider can play a role in the overall loop gain as I described in my answer here. \$\endgroup\$
    – Verbal Kint
    Commented Nov 21, 2022 at 6:47

1 Answer 1

Reset to default
4
\$\begingroup\$

The diagram shows an ideal block diagram.

In real life, you don't have ideal wires, resistors and comparators.

You always have to deal with non-idealities, such as wires having resistance, inductance, and capacitance to nearby wires.

The feedback input will also not have infinite input impedance or zero pin capacitance.

When you use a chip, it will have a section which suggests what resistance ranges to use for best results.

If you ignore the capacitance, then input impedance defines how large the resistor values can be before the error due to input impedance becomes large enough to affect the output.

And when taking the feedback pin capacitance into account, the divider impedance being high takes more time to charge the pin capacitance so it has a lag reacting to changes in output voltage.

So the feedback divider must have only a suitable amount of lag, not too much or little, to make the whole feedback loop work in stable fashion.

In some cases a feedforward capacitor is put between output and feedback pin to alter the feedback properties to make the whole system more stable and react better to transients on output.

\$\endgroup\$
8
  • \$\begingroup\$ +1 nice point about the input impedance and capacitance. What if the error amp has infinite input impedance and zero input capacitance, how R1, R2 still affect the stability? \$\endgroup\$
    – internet
    Commented Nov 20, 2022 at 21:32
  • \$\begingroup\$ Why would it if the whole circuit is ideal? \$\endgroup\$
    – Justme
    Commented Nov 20, 2022 at 21:33
  • \$\begingroup\$ Just assume that the controller components are ideal so we can focus on the main reason, I think it would affect the overall loop gain? \$\endgroup\$
    – internet
    Commented Nov 20, 2022 at 21:35
  • \$\begingroup\$ Why it would affect it? For any output voltage the feedback gets same voltage, regardless of the resistors being 1 ohm or 1 megaohm in an ideal circuit? \$\endgroup\$
    – Justme
    Commented Nov 20, 2022 at 21:39
  • 2
    \$\begingroup\$ In a practical circuit, yes. But you asked if the error amp input is ideal, infinite impedance, no capacitance. In an ideal circuit, output is exactly identical since the divider impedance is irrelevant. \$\endgroup\$
    – Justme
    Commented Nov 20, 2022 at 22:00

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.