How to find a missing resistor?

Question

This seems to be a really simple question. I'm not really sure where I went wrong in my solution. Please forgive me if this seems irritatingly simple, I've only just started learning about electric circuits here in university, and am having a hard time. But I'm willing to learn and learn and learn until I get it right.

May you please tell me how this was solved so that I may know where I went wrong and correct my mistake.

Answer 1

The switch has been closed for a long time

This allows you to neglect the capacitor at this point

Before the switch is opened, the voltage across R1 is 5V
R1 is 3 Ω
R2 is 9 Ω

This allows you to calculate V1, and the capacitor voltage

The energy stored in the capacitor is 3.375 J

Lets you compute the value of C1

After one second with S1 open, the new capacitor voltage is 1.6255... V

Lets you compute the RC time constant, and so the series combo of R2 and R3. You know what R2 is.

Answer 2

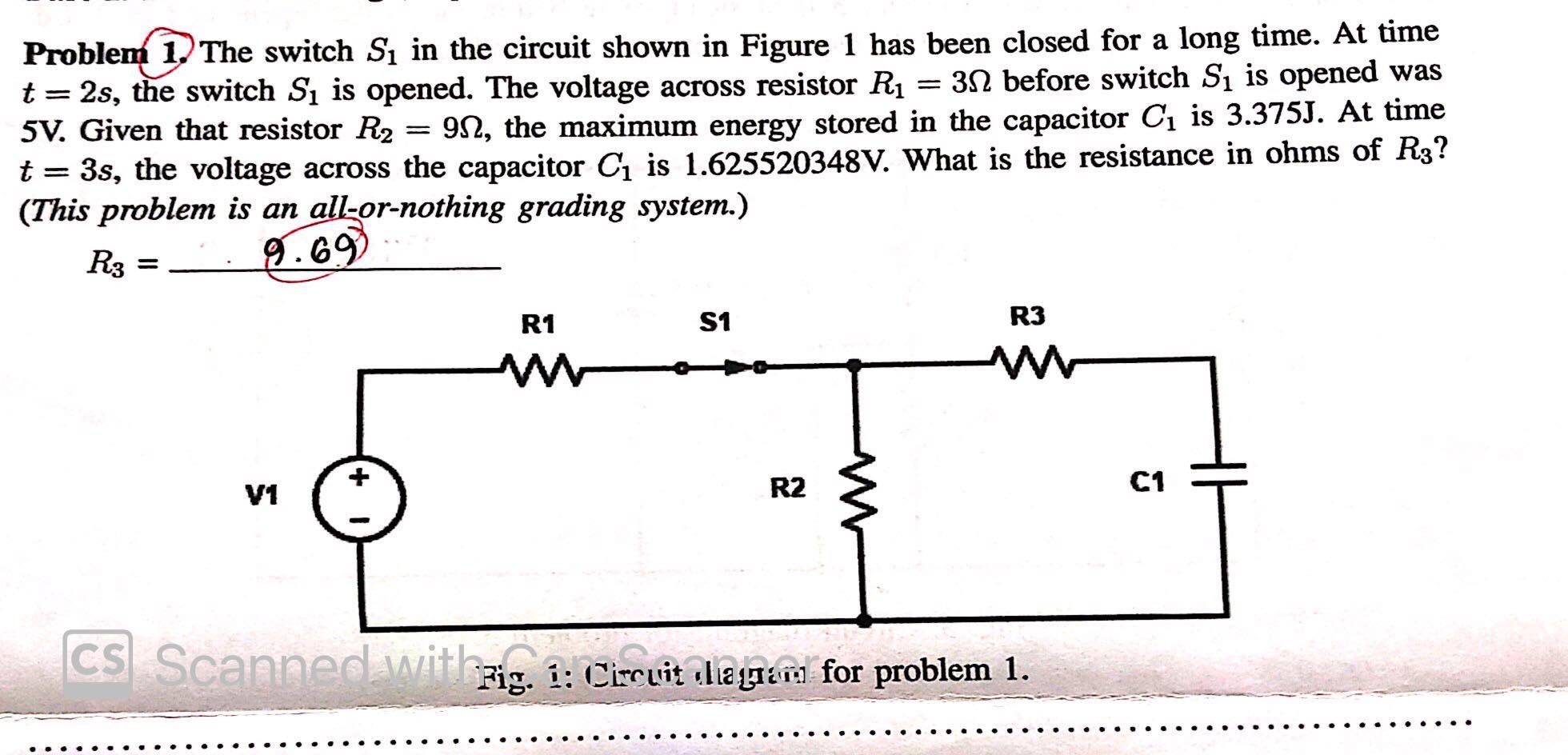
The problem is just making sure you can read and understand what's written. So the author gave you information in odd ways.

First off, you know that the voltage across the capacitor must be \$15\:\text{V}\$ at the moment the switch is opened. This follows because you know there is \$5\:\text{V}\$ across the \$R_1=3\:\Omega\$ resistor and you know that \$R_2=9\:\Omega\$ therefore, being three times larger, must have \$15\:\text{V}\$ across it and this must be what the capacitor was charged up to.

From this, and the given energy stored, you can work out the capacitance is \$30\:\text{mF}\$ and that the charge stored on it is \$450\:\text{mC}\$.

Now, at the end, you are told the voltage across the capacitor. So from this you can work out the final charge as \$1.625520348\:\text{V}\cdot 30\:\text{mF} = 48.7656104 \:\text{mC}\$.

Since you know that \$\frac{Q}{C}=i\cdot R\$ where \$i=\frac{\text{d}\,Q}{\text{d}t}\$ it follows that you need the solution to the differential equation, which is \$Q_t=Q_0\cdot\exp\left(-\frac{t}{R\,C}\right)\$. In short, all you need to do is find the value x in:

$$450\:\text{mC}\cdot\exp\left(-\frac{3\:\text{s}-2\:\text{s}}{\left(9\:\Omega+x\right)\cdot 30\:\text{mF}}\right)=48.7656104 \:\text{mC}$$

That solves out almost exactly to \$6\:\Omega\$. Which is surprisingly 'nice'.